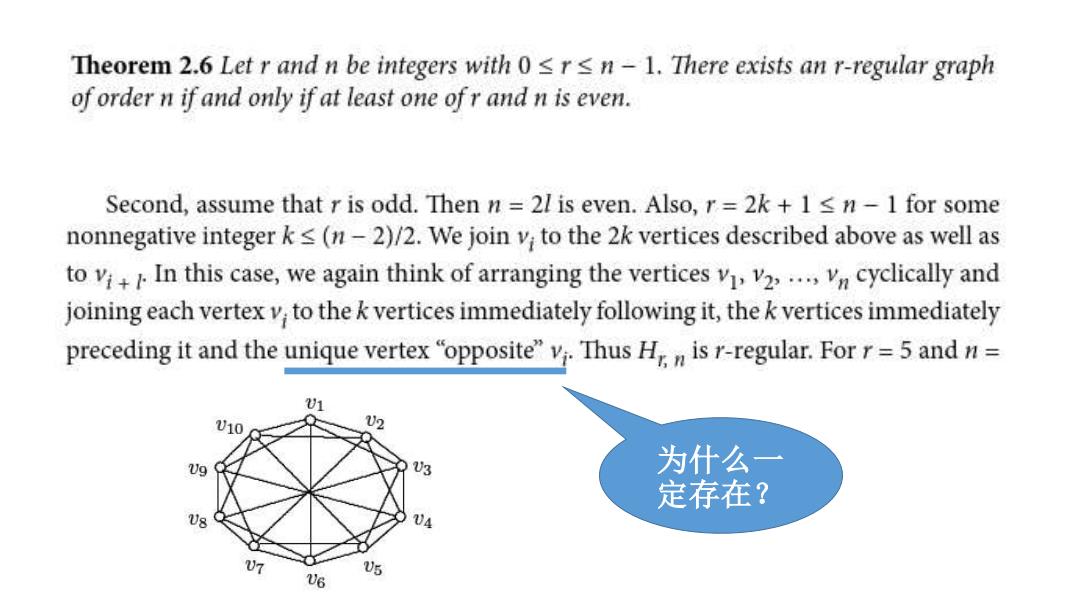
Theorem 2.6 Let r and n be integers with 0sr s n-1.There exists an r-regular graph of order n if and only if at least one of r and n is even. Second,assume that r is odd.Then n 2l is even.Also,r=2k +1 s n-1 for some nonnegative integer ks(n-2)/2.We join v;to the 2k vertices described above as well as to vi+In this case,we again think of arranging the vertices vv..ncyclically and joining each vertex v;to the k vertices immediately following it,the k vertices immediately preceding it and the unique vertex"opposite"vThus Hn is r-regular.Forr=5 and n= U10 Ug 为什么一 定存在？ U U7 U5 U6为什么一 定存在？