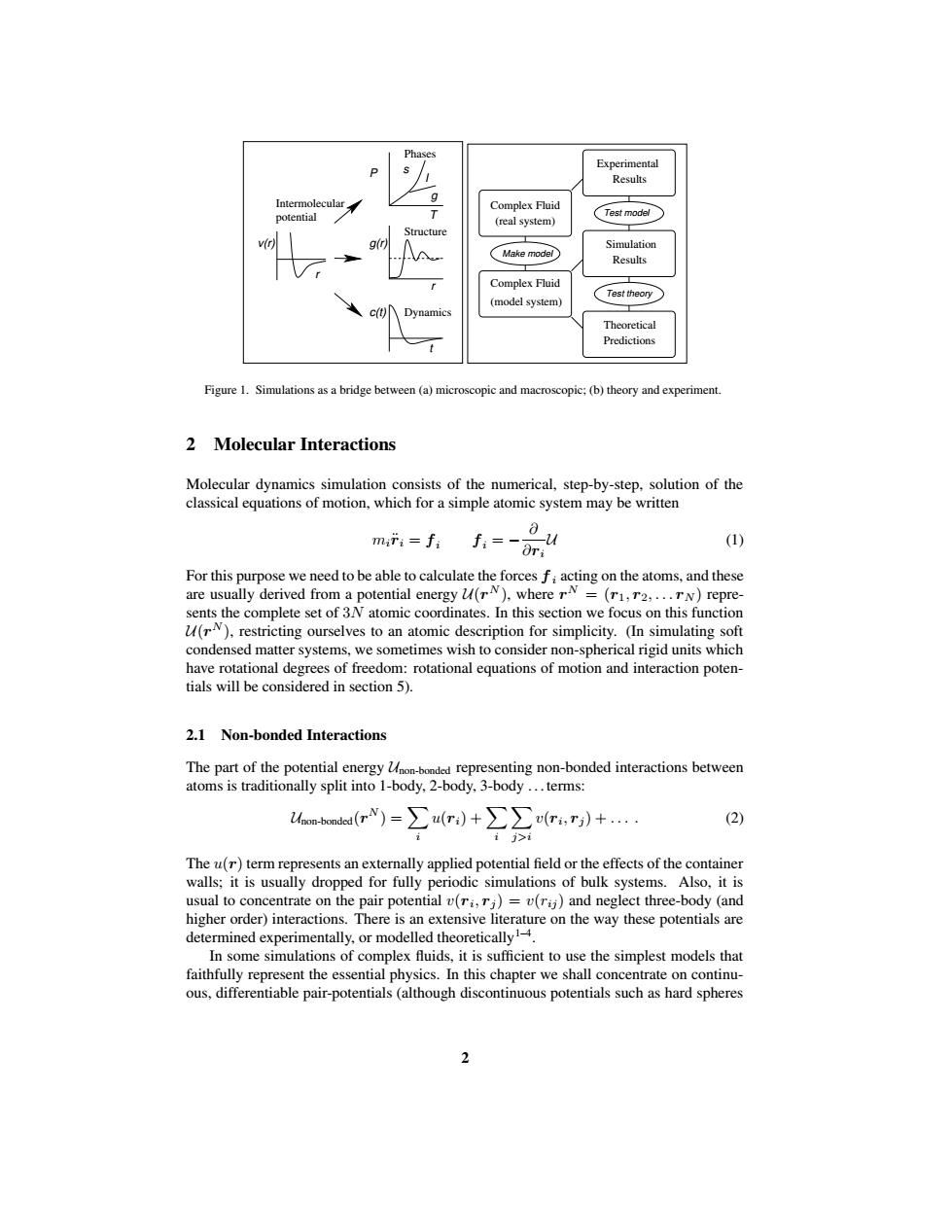
Experimental Results Intermolecular Complex Fluid potential Test model (real system) Structure v(r g(r Simulation Make model Results Complex Fluid Test theory (model system) c(t) Dynamics Theoretical Predictions Figure 1.Simulations as a bridge between (a)microscopic and macroscopic;(b)theory and experiment. 2 Molecular Interactions Molecular dynamics simulation consists of the numerical,step-by-step,solution of the classical equations of motion,which for a simple atomic system may be written miri=fi fi=-Ori (1) For this purpose we need to be able to calculate the forces f;acting on the atoms,and these are usually derived from a potential energy u(rN),where rN =(r1,r2,...rN)repre- sents the complete set of 3N atomic coordinates.In this section we focus on this function (rN),restricting ourselves to an atomic description for simplicity.(In simulating soft condensed matter systems,we sometimes wish to consider non-spherical rigid units which have rotational degrees of freedom:rotational equations of motion and interaction poten- tials will be considered in section 5). 2.1 Non-bonded Interactions The part of the potential energy Uhon-bonded representing non-bonded interactions between atoms is traditionally split into 1-body,2-body,3-body...terms: a-bondod(r）=∑a(r)+∑(r,r)+ (2) i j>i The u(r)term represents an externally applied potential field or the effects of the container walls;it is usually dropped for fully periodic simulations of bulk systems.Also,it is usual to concentrate on the pair potential v(ri,rj)=v(r)and neglect three-body (and higher order)interactions.There is an extensive literature on the way these potentials are determined experimentally,or modelled theoretically1-4. In some simulations of complex fluids,it is sufficient to use the simplest models that faithfully represent the essential physics.In this chapter we shall concentrate on continu- ous,differentiable pair-potentials(although discontinuous potentials such as hard spheres 2Intermolecular T r t c(t) P Structure Dynamics Phases s l g g(r) r v(r) potential Complex Fluid (real system) Complex Fluid (model system) Make model Simulation Experimental Results Results Predictions Theoretical Test theory Test model Figure 1. Simulations as a bridge between (a) microscopic and macroscopic; (b) theory and experiment. 2 Molecular Interactions Molecular dynamics simulation consists of the numerical, step-by-step, solution of the classical equations of motion, which for a simple atomic system may be written mir¨i = fi fi = − ∂ ∂ri U (1) For this purpose we need to be able to calculate the forces f i acting on the atoms, and these are usually derived from a potential energy U(r N ), where r N = (r1, r2, . . . rN ) repre￾sents the complete set of 3N atomic coordinates. In this section we focus on this function U(r N ), restricting ourselves to an atomic description for simplicity. (In simulating soft condensed matter systems, we sometimes wish to consider non-spherical rigid units which have rotational degrees of freedom: rotational equations of motion and interaction poten￾tials will be considered in section 5). 2.1 Non-bonded Interactions The part of the potential energy Unon-bonded representing non-bonded interactions between atoms is traditionally split into 1-body, 2-body, 3-body . . . terms: Unon-bonded(r N ) = X i u(ri) + X i X j>i v(ri , rj ) + . . . . (2) The u(r) term represents an externally applied potential field or the effects of the container walls; it is usually dropped for fully periodic simulations of bulk systems. Also, it is usual to concentrate on the pair potential v(ri , rj ) = v(rij ) and neglect three-body (and higher order) interactions. There is an extensive literature on the way these potentials are determined experimentally, or modelled theoretically1–4 . In some simulations of complex fluids, it is sufficient to use the simplest models that faithfully represent the essential physics. In this chapter we shall concentrate on continu￾ous, differentiable pair-potentials (although discontinuous potentials such as hard spheres 2