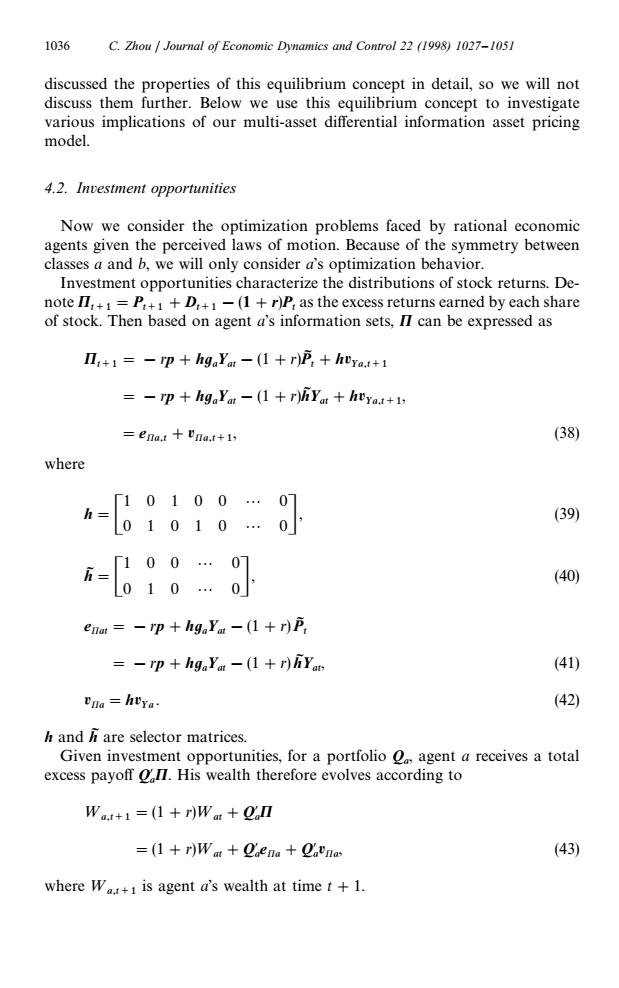
1036 C.Zhou Journal of Economic Dynamics and Control 22 (1998)1027-1051 discussed the properties of this equilibrium concept in detail,so we will not discuss them further.Below we use this equilibrium concept to investigate various implications of our multi-asset differential information asset pricing model. 4.2.Investment opportunities Now we consider the optimization problems faced by rational economic agents given the perceived laws of motion.Because of the symmetry between classes a and b,we will only consider a's optimization behavior. Investment opportunities characterize the distributions of stock returns.De- note I,+1=P+1+D+1-(1+r)P,as the excess returns earned by each share of stock.Then based on agent a's information sets,II can be expressed as Ⅱ，+1=-Tp+hgaYat-(1+rP,+ha+1 =-rp hgaYat-(1 +r)Yat heya+1 =ena.t Una.t+1, (38) where (39) (40) enat -rp hgaYat -(1 +r)P =-rp hgaYat -(1 +r)hYat (410 Una hUya. (42) h and h are selector matrices. Given investment opportunities,for a portfolio agent a receives a total excess payoff on.His wealth therefore evolves according to Wat+1=(1+r)Wam+aⅡ =(1+r）Wa+ena+a'nam (43) where Wa.+i is agent a's wealth at time t +1.discussed the properties of this equilibrium concept in detail, so we will not discuss them further. Below we use this equilibrium concept to investigate various implications of our multi-asset differential information asset pricing model. 4.2. Investment opportunities Now we consider the optimization problems faced by rational economic agents given the perceived laws of motion. Because of the symmetry between classes a and b, we will only consider a’s optimization behavior. Investment opportunities characterize the distributions of stock returns. De￾note Pt`1 "Pt`1 #Dt`1 !(1#r)Pt as the excess returns earned by each share of stock. Then based on agent a’s information sets, P can be expressed as Pt`1 "!rp#hu a Yat!(1#r)PI t #h€ Ya,t`1 "!rp#hu a Yat!(1#r)hIYat#h€ Ya,t`1 , "ePa,t #€Pa,t`1 , (38) where h"C 10100 2 0 01010 2 0D, (39) hI"C 100 2 0 010 2 0D, (40) ePat"!rp#hu a Yat!(1#r) PI t "!rp#hu a Yat!(1#r) hIYat, (41) €Pa "h€ Ya. (42) h and hI are selector matrices. Given investment opportunities, for a portfolio Qa , agent a receives a total excess payoff Q@ a P. His wealth therefore evolves according to ¼a,t`1 "(1#r)¼at#Q@ a P "(1#r)¼at#Q@ a ePa #Q@ a €Pa , (43) where ¼a,t`1 is agent a’s wealth at time t#1. 1036 C. Zhou / Journal of Economic Dynamics and Control 22 (1998) 1027–1051