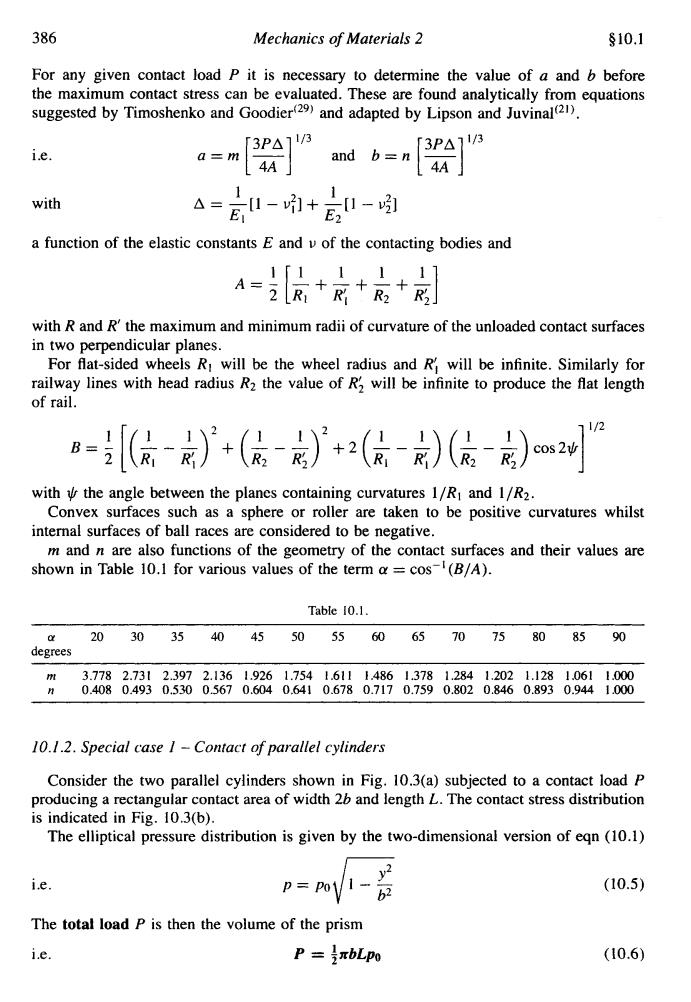
386 Mechanics of Materials 2 §10.1 For any given contact load P it is necessary to determine the value of a and b before the maximum contact stress can be evaluated.These are found analytically from equations suggested by Timoshenko and Goodier(29)and adapted by Lipson and Juvinal(21). ie. 「3PA]/3 a=m and b=n 「3PA7/3 4A 4A with △= -+-1 a function of the elastic constants E and v of the contacting bodies and ++后+ A- with R and R'the maximum and minimum radii of curvature of the unloaded contact surfaces in two perpendicular planes. For flat-sided wheels R:will be the wheel radius and R will be infinite.Similarly for railway lines with head radius R2 the value of R2 will be infinite to produce the flat length of rail. 1/2 8=(信)'+（后）+（信-）（信忘）m with the angle between the planes containing curvatures 1/RI and 1/R2. Convex surfaces such as a sphere or roller are taken to be positive curvatures whilst internal surfaces of ball races are considered to be negative. m and n are also functions of the geometry of the contact surfaces and their values are shown in Table 10.1 for various values of the term a cos-(B/A). Table 10.1. 20 3035 40 4550 5560 6570 75 80 85 90 degrees N 3.7782.7312.3972.1361.9261.7541.61114861.3781.2841.2021.1281.0611.000 0.4080.4930.5300.5670.6040.6410.6780.7170.7590.8020.8460.8930.9441000 10.1.2.Special case I-Contact of parallel cylinders Consider the two parallel cylinders shown in Fig.10.3(a)subjected to a contact load P producing a rectangular contact area of width 26 and length L.The contact stress distribution is indicated in Fig.10.3(b). The elliptical pressure distribution is given by the two-dimensional version of eqn (10.1) ie. p= -茶 (10.5) The total load P is then the volume of the prism i.e. P=πbLpo (10.6)3 86 Mechanics of Materials 2 $10.1 For any given contact load P it is necessary to determine the value of a and b before the maximum contact stress can be evaluated. These are found analytically from equations suggested by Timoshenko and G~odier'~~) and adapted by Lipson and Juvinal(2'). i.e. with 3PA and b = n [=] a function of the elastic constants E and v of the contacting bodies and 11 with R and R' the maximum and minimum radii of curvature of the unloaded contact surfaces in two perpendicular planes. For flat-sided wheels RI will be the wheel radius and R', will be infinite. Similarly for railway lines with head radius R2 the value of R; will be infinite to produce the flat length of rail. with 11/ the angle between the planes containing curvatures l/Rl and 1/R;?. internal surfaces of ball races are considered to be negative. shown in Table 10.1 for various values of the term a = cos-'(B/A). Convex surfaces such as a sphere or roller are taken to be positive curvatures whilst rn and n are also functions of the geometry of the contact surfaces and their values are Table 10.1. a 20 30 35 40 45 50 55 60 65 70 75 80 85 90 degrees m 3.778 2.731 2.397 2.136 1.926 1.754 1.611 1.486 1.378 1.284 1.202 1.128 1.061 1.OOO n 0.408 0.493 0.530 0.567 0.604 0.641 0.678 0.717 0.759 0.802 0.846 0.893 0.944 1.OOO 10.1.2. Special case I - Contact of parallel cylinders Consider the two parallel cylinders shown in Fig. 10.3(a) subjected to a contact load P producing a rectangular contact area of width 26 and length L. The contact stress distribution is indicated in Fig. 10.3(b). The elliptical pressure distribution is given by the two-dimensional version of eqn (10.1) i.e. P = Pq/l - g The total load P is then the volume of the prism i.e. P = inbLpo (10.5) (10.6)