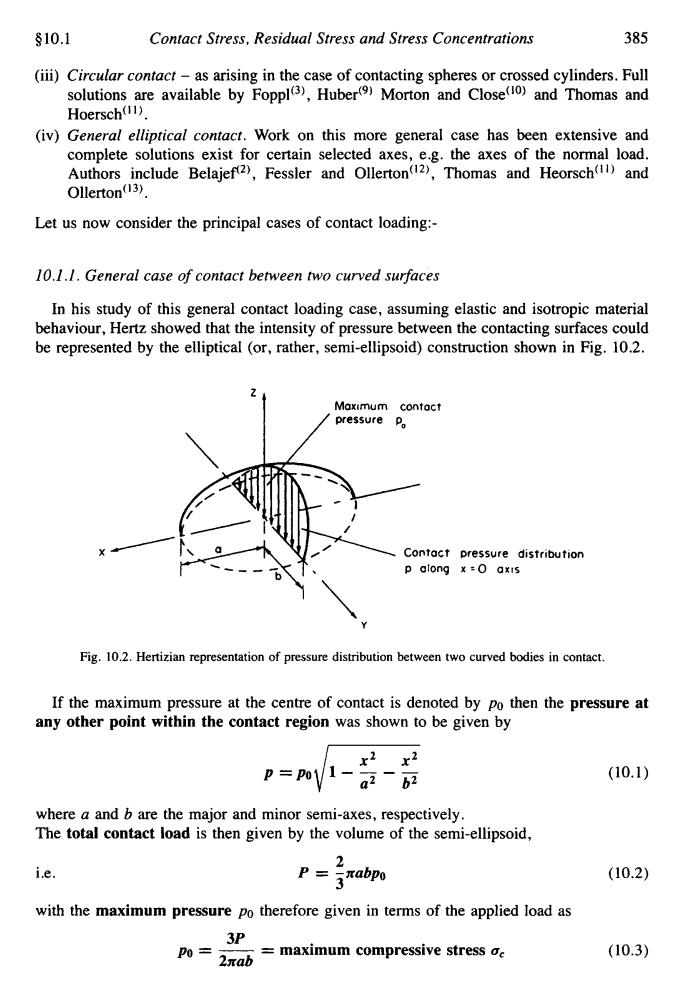
s10.1 Contact Stress,Residual Stress and Stress Concentrations 385 (iii)Circular contact-as arising in the case of contacting spheres or crossed cylinders.Full solutions are available by Foppl(3),Huber(9)Morton and Close(10)and Thomas and Hoersch(11). (iv)General elliptical contact.Work on this more general case has been extensive and complete solutions exist for certain selected axes,e.g.the axes of the normal load. Authors include Belajef(2),Fessler and Ollerton(12),Thomas and Heorsch(1)and Ollerton(13) Let us now consider the principal cases of contact loading:- 10.1.1.General case of contact between two curved surfaces In his study of this general contact loading case,assuming elastic and isotropic material behaviour,Hertz showed that the intensity of pressure between the contacting surfaces could be represented by the elliptical (or,rather,semi-ellipsoid)construction shown in Fig.10.2. Maximum contact pressure Contact pressure distribution p along x=axis Fig.10.2.Hertizian representation of pressure distribution between two curved bodies in contact. If the maximum pressure at the centre of contact is denoted by po then the pressure at any other point within the contact region was shown to be given by x2 x2 P =po/1- ai-bi (10.1) where a and b are the major and minor semi-axes,respectively. The total contact load is then given by the volume of the semi-ellipsoid, 2 i.e. P 3xabpo (10.2) with the maximum pressure po therefore given in terms of the applied load as 3P P0= maximum compressive stress oe (10.3) 2nab$10.1 Contact Stress, Residual Stress and Stress Concentrations 385 (iii) Circular contact - as arising in the case of contacting spheres or crossed cylinders. Full solutions are available by F~ppl(~), Huber") Morton and Close(") and Thomas and Hoersch(' I. (iv) General elliptical contact. Work on this more general case has been extensive and complete solutions exist for certain selected axes, e.g. the axes of the normal load. Authors include Belajef"), Fessler and Ollerton('*), Thomas and Heorsch(") and oiiert~n(~~). Let us now consider the principal cases of contact loading:- 10.1.1. General case of contact between two curved surj4aces In his study of this general contact loading case, assuming elastic and isotropic material behaviour, Hertz showed that the intensity of pressure between the contacting surfaces could be represented by the elliptical (or, rather, semi-ellipsoid) construction shown in Fig. 10.2. 2, Maximum contact COntOCt pressure distribution P along x =O OXIS Y Fig. 10.2. Hertizian representation of pressure distribution between two curved bodies in contact. If the maximum pressure at the centre of contact is denoted by po then the pressure at any other point within the contact region was shown to be given by (10.1) where a and b are the major and minor semi-axes, respectively. The total contact load is then given by the volume of the semi-ellipsoid, i.e. with the maximum pressure po therefore given in terms of the applied load as 2 3 P = -nabPo (10.2) 3P 2zab po = - = maximum compressive stress a, (10.3)