正在加载图片...
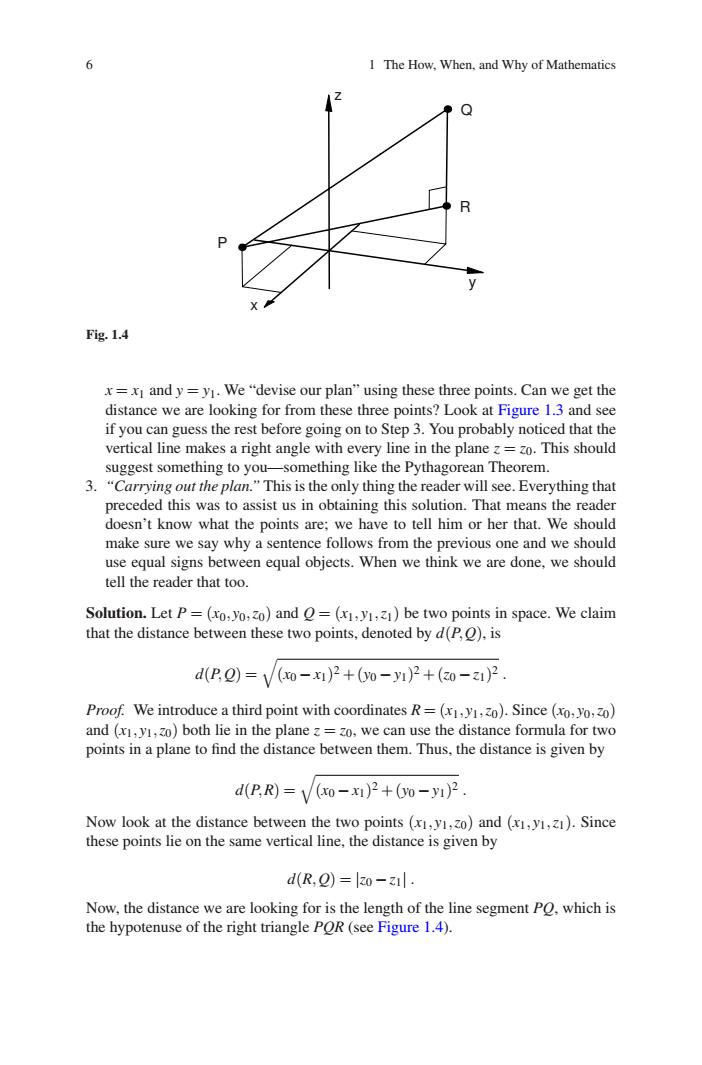
6 I The How,When,and Why of Mathematics Fig.1.4 x=x and y=y.We "devise our plan"using these three points.Can we get the distance we are looking for from these three points?Look at Figure 1.3 and see if you can guess the rest before going on to Step 3.You probably noticed that the vertical line makes a right angle with every line in the plane z=zo.This should suggest something to you-something like the Pythagorean Theorem. 3."Carrying out the plan."This is the only thing the reader will see.Everything that preceded this was to assist us in obtaining this solution.That means the reader doesn't know what the points are;we have to tell him or her that.We should make sure we say why a sentence follows from the previous one and we should use equal signs between equal objects.When we think we are done,we should tell the reader that too. Solution.Let P=(xo,yo,zo)and =(x1,y1,z1)be two points in space.We claim that the distance between these two points,denoted by d(P,O),is d(P0)=V(o-x)2+0o-yn)2+(o-z12 Proof.We introduce a third point with coordinates R=(x1,y1,z0).Since (xo,yo,zo) and (x1,y1,zo)both lie in the plane z=zo,we can use the distance formula for two points in a plane to find the distance between them.Thus,the distance is given by d(P,R)=V(xo-x1)2+(vo-yI)2 Now look at the distance between the two points (xi,y1,zo)and (xi,y1,z1).Since these points lie on the same vertical line,the distance is given by d(R,Q)=o-z1 Now,the distance we are looking for is the length of the line segment PO,which is the hypotenuse of the right triangle POR (see Figure 1.4).6 1 The How, When, and Why of Mathematics x y z P R Q Fig. 1.4 x = x1 and y = y1. We “devise our plan” using these three points. Can we get the distance we are looking for from these three points? Look at Figure 1.3 and see if you can guess the rest before going on to Step 3. You probably noticed that the vertical line makes a right angle with every line in the plane z = z0. This should suggest something to you—something like the Pythagorean Theorem. 3. “Carrying out the plan.” This is the only thing the reader will see. Everything that preceded this was to assist us in obtaining this solution. That means the reader doesn’t know what the points are; we have to tell him or her that. We should make sure we say why a sentence follows from the previous one and we should use equal signs between equal objects. When we think we are done, we should tell the reader that too. Solution. Let P = (x0, y0,z0) and Q = (x1,y1,z1) be two points in space. We claim that the distance between these two points, denoted by d(P,Q), is d(P,Q) = q (x0 −x1)2 + (y0 −y1)2 + (z0 −z1)2 . Proof. We introduce a third point with coordinates R = (x1,y1,z0). Since (x0,y0,z0) and (x1,y1,z0) both lie in the plane z = z0, we can use the distance formula for two points in a plane to find the distance between them. Thus, the distance is given by d(P,R) = q (x0 −x1)2 + (y0 −y1)2 . Now look at the distance between the two points (x1,y1,z0) and (x1,y1,z1). Since these points lie on the same vertical line, the distance is given by d(R,Q) = |z0 −z1| . Now, the distance we are looking for is the length of the line segment PQ, which is the hypotenuse of the right triangle PQR (see Figure 1.4)