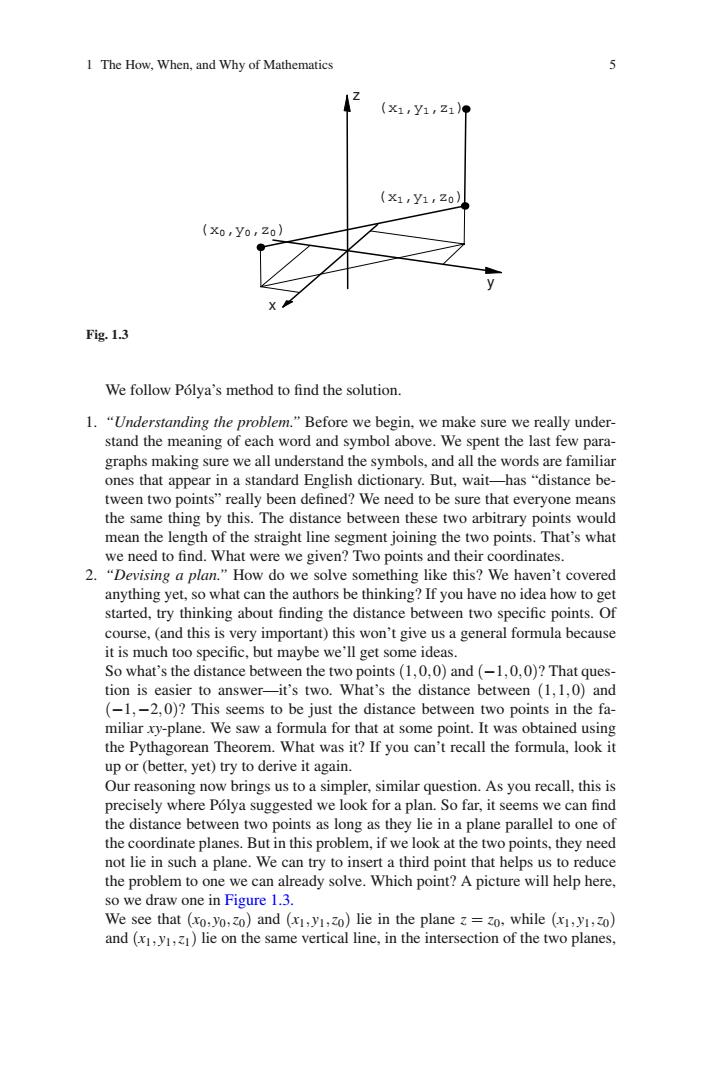
I The How,When,and Why of Mathematics 5 (X1,y1,21p （X1,y1,z0） (xo,Yo,Zo) Fig.1.3 We follow Polya's method to find the solution. 1."Understanding the problem."Before we begin,we make sure we really under- stand the meaning of each word and symbol above.We spent the last few para- graphs making sure we all understand the symbols,and all the words are familiar ones that appear in a standard English dictionary.But,wait-has"distance be- tween two points"really been defined?We need to be sure that everyone means the same thing by this.The distance between these two arbitrary points would mean the length of the straight line segment joining the two points.That's what we need to find.What were we given?Two points and their coordinates. 2."Devising a plan."How do we solve something like this?We haven't covered anything yet,so what can the authors be thinking?If you have no idea how to get started,try thinking about finding the distance between two specific points.Of course,(and this is very important)this won't give us a general formula because it is much too specific,but maybe we'll get some ideas. So what's the distance between the two points(1,0,0)and(-1,0,0)?That ques- tion is easier to answer-it's two.What's the distance between (1,1,0)and (-1,-2,0)?This seems to be just the distance between two points in the fa- miliar xy-plane.We saw a formula for that at some point.It was obtained using the Pythagorean Theorem.What was it?If you can't recall the formula,look it up or(better,yet)try to derive it again. Our reasoning now brings us to a simpler,similar question.As you recall,this is precisely where Polya suggested we look for a plan.So far,it seems we can find the distance between two points as long as they lie in a plane parallel to one of the coordinate planes.But in this problem,if we look at the two points,they need not lie in such a plane.We can try to insert a third point that helps us to reduce the problem to one we can already solve.Which point?A picture will help here, so we draw one in Figure 1.3. We see that (xo,yo,zo)and (xi,y1,zo)lie in the plane z=zo.while (x1,y1,) and (x1,y1,1)lie on the same vertical line,in the intersection of the two planes,1 The How, When, and Why of Mathematics 5 x y z (x 0 ,y 0 ,z 0 ) (x 1 ,y 1 ,z 0 ) (x 1 ,y 1 ,z 1 ) Fig. 1.3 We follow Polya’s method to find the solution. ´ 1. “Understanding the problem.” Before we begin, we make sure we really under￾stand the meaning of each word and symbol above. We spent the last few para￾graphs making sure we all understand the symbols, and all the words are familiar ones that appear in a standard English dictionary. But, wait—has “distance be￾tween two points” really been defined? We need to be sure that everyone means the same thing by this. The distance between these two arbitrary points would mean the length of the straight line segment joining the two points. That’s what we need to find. What were we given? Two points and their coordinates. 2. “Devising a plan.” How do we solve something like this? We haven’t covered anything yet, so what can the authors be thinking? If you have no idea how to get started, try thinking about finding the distance between two specific points. Of course, (and this is very important) this won’t give us a general formula because it is much too specific, but maybe we’ll get some ideas. So what’s the distance between the two points (1,0,0) and (−1,0,0)? That ques￾tion is easier to answer—it’s two. What’s the distance between (1,1,0) and (−1,−2,0)? This seems to be just the distance between two points in the fa￾miliar xy-plane. We saw a formula for that at some point. It was obtained using the Pythagorean Theorem. What was it? If you can’t recall the formula, look it up or (better, yet) try to derive it again. Our reasoning now brings us to a simpler, similar question. As you recall, this is precisely where Polya suggested we look for a plan. So far, it seems we can find ´ the distance between two points as long as they lie in a plane parallel to one of the coordinate planes. But in this problem, if we look at the two points, they need not lie in such a plane. We can try to insert a third point that helps us to reduce the problem to one we can already solve. Which point? A picture will help here, so we draw one in Figure 1.3. We see that (x0,y0,z0) and (x1,y1,z0) lie in the plane z = z0, while (x1,y1,z0) and (x1, y1,z1) lie on the same vertical line, in the intersection of the two planes