正在加载图片...
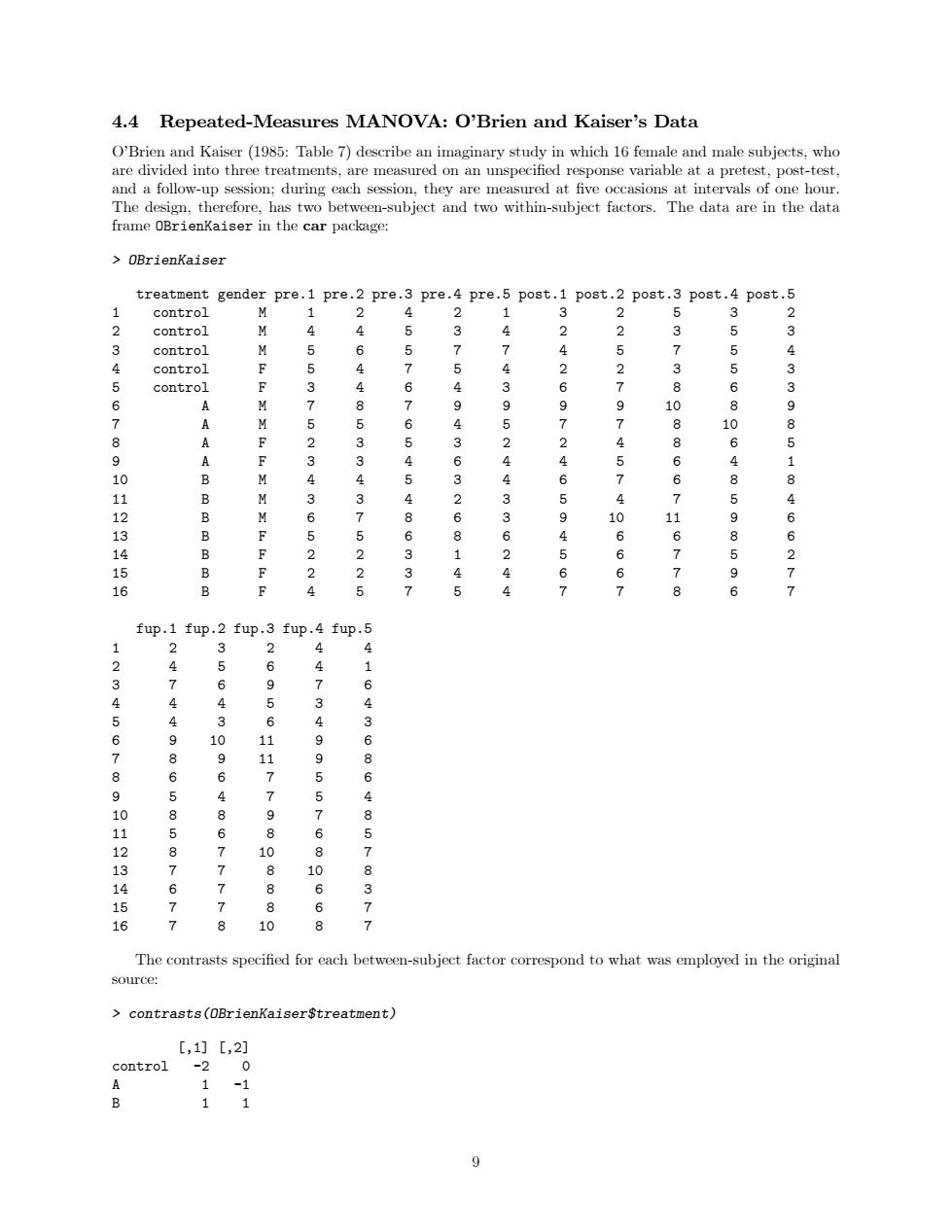
4.4 Repeated-Measures MANOVA:O'Brien and Kaiser's Data O'Brien and Kaiser (1985:Table 7)describe an imaginary study in which 16 female and male subjects,who are divided into three treatments,are measured on an unspecified response variable at a pretest,post-test, and a follow-up session:during each session,they are measured at five occasions at intervals of one hour. The design,therefore,has two between-subject and two within-subject factors.The data are in the data frame OBrienKaiser in the car package: OBrienKaiser treatment gender pre.1 pre.2 pre.3 pre.4 pre.5 post.1 post.2 post.3 post.4 post.5 1 control M 1 2 4 2 1 3 2 3 2 control M 4 4 2 3 3 3 control M 5 6 6 > 6 4 control F 5 4 57 5 P 3 5 3 5 control F 3 4 67 3 6 6 3 M 9 9 9 9 10 9 7 5 5 6 4 5 1 7 8 10 8 8 A F 2 3 6 5 9 F 3 3 6 5 6 1 10 M 4 4 5 6 6 8 8 11 12 BBBBBB 3 7 8 10 9 6 14 52 6 8 6 7 15 F 4 24 9 62 F 4 5225 6337 567 > fup.1 fup.2 fup.3 fup.4 fup.5 2 4 23456789 7 3564 4473 4 3 269561 4 10 9 9 6 6 1 5 5 4 7 8 11 5 6 980 57 16436864857 68 3 7 7 1 6 15 77 888 8377 16 0668 8 10 The contrasts specified for each between-subject factor correspond to what was employed in the original source: contrasts(OBrienKaiserStreatment) [,1][,2] control -2 0 B 1 1 94.4 Repeated-Measures MANOVA: O’Brien and Kaiser’s Data O’Brien and Kaiser (1985: Table 7) describe an imaginary study in which 16 female and male subjects, who are divided into three treatments, are measured on an unspecified response variable at a pretest, post-test, and a follow-up session; during each session, they are measured at five occasions at intervals of one hour. The design, therefore, has two between-subject and two within-subject factors. The data are in the data frame OBrienKaiser in the car package: > OBrienKaiser treatment gender pre.1 pre.2 pre.3 pre.4 pre.5 post.1 post.2 post.3 post.4 post.5 1 control M 1 2 4 2 132532 2 control M 4 4 5 3 422353 3 control M 5 6 5 7 745754 4 control F 5 4 7 5 422353 5 control F 3 4 6 4 367863 6 A M 7 8 7 9 9 9 9 10 8 9 7 A M 5 5 6 4 5 7 7 8 10 8 8 A F23532 2 4 8 6 5 9 A F33464 4 5 6 4 1 10 B M 4 4 5 3 4 6 7 6 8 8 11 B M 3 3 4 2 3 5 4 7 5 4 12 B M 6 7 8 6 3 9 10 11 9 6 13 B F 5 5 6 8 6 4 6 6 8 6 14 B F 2 2 3 1 2 5 6 7 5 2 15 B F 2 2 3 4 4 6 6 7 9 7 16 B F 4 5 7 5 4 7 7 8 6 7 fup.1 fup.2 fup.3 fup.4 fup.5 1 23244 2 45641 3 76976 4 44534 5 43643 6 9 10 11 9 6 7 8 9 11 9 8 8 66756 9 54754 10 8 8 9 7 8 11 5 6 8 6 5 12 8 7 10 8 7 13 7 7 8 10 8 14 6 7 8 6 3 15 7 7 8 6 7 16 7 8 10 8 7 The contrasts specified for each between-subject factor correspond to what was employed in the original source: > contrasts(OBrienKaiser$treatment) [,1] [,2] control -2 0 A 1 -1 B 11 9