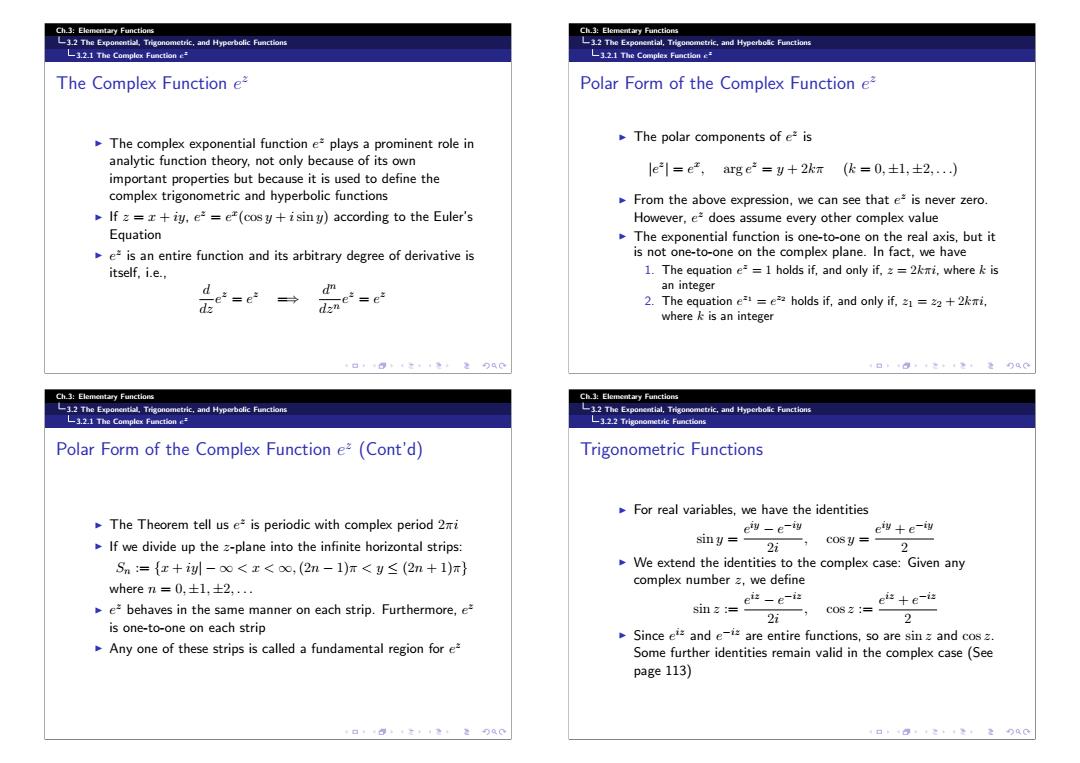
Ch.3:Elementary Functions Ch.3:Elementary Functions L3.2 The Exponential Trigonometric,and Hyperboic Functions L3.2 The Exponential,Trigonometric,and Hyperboc Functions L3.2.1 The Complex Function e= L3.2.1 The Complex Function e The Complex Function e2 Polar Form of the Complex Function e The complex exponential function es plays a prominent role in The polar components of e*is analytic function theory,not only because of its own important properties but because it is used to define the |e1=e,arge=y+2kx(k=0,±1，±2，.) complex trigonometric and hyperbolic functions From the above expression,we can see that e=is never zero. If z=x+iy,es e(cosy+isiny)according to the Euler's However,e=does assume every other complex value Equation The exponential function is one-to-one on the real axis,but it e is an entire function and its arbitrary degree of derivative is is not one-to-one on the complex plane.In fact,we have itself,i.e., 1.The equation e==1 holds if,and only if,2=2kmi,where k is an integer e=e2=→ -e2=e 2.The equation e==e*holds if,and only if,21=22+2mi, where k is an integer Ch.3:Elementary Functions Ch.3:Elementary Functions 3.2 The Exponential,Trigonometric,and Hyperbolic Functions 3.2 The Exponential,Trigonometric,and Hyperbolic Functions L3.2.1 The Complex Function e= L3.2.2 Trigonometric Functions Polar Form of the Complex Function e*(Cont'd) Trigonometric Functions For real variables,we have the identities The Theorem tell us e=is periodic with complex period 2xi eiy-e-iy eiy+e-iy If we divide up the z-plane into the infinite horizontal strips: siny=2i cosy= 2 S={x+i-o<x<o,(2n-1)m<y≤(2n+1)x} We extend the identities to the complex case:Given any where n=0,±l,±2，.. complex number z,we define eiz-e-iz imz=2—, eiste-is e*behaves in the same manner on each strip.Furthermore,e C0s2:= 2 is one-to-one on each strip Since eis and e-is are entire functions,so are sin z and cos z. Any one of these strips is called a fundamental region for es Some further identities remain valid in the complex case(See page 113)Ch.3: Elementary Functions 3.2 The Exponential, Trigonometric, and Hyperbolic Functions 3.2.1 The Complex Function ez The Complex Function ez The complex exponential function ez plays a prominent role in analytic function theory, not only because of its own important properties but because it is used to define the complex trigonometric and hyperbolic functions If z = x + iy, ez = ex(cos y + isin y) according to the Euler’s Equation ez is an entire function and its arbitrary degree of derivative is itself, i.e., d dz ez = ez =⇒ dn dzn ez = ez Ch.3: Elementary Functions 3.2 The Exponential, Trigonometric, and Hyperbolic Functions 3.2.1 The Complex Function ez Polar Form of the Complex Function ez The polar components of ez is |ez| = ex, arg ez = y + 2kπ (k = 0, ±1, ±2,...) From the above expression, we can see that ez is never zero. However, ez does assume every other complex value The exponential function is one-to-one on the real axis, but it is not one-to-one on the complex plane. In fact, we have 1. The equation ez = 1 holds if, and only if, z = 2kπi, where k is an integer 2. The equation ez1 = ez2 holds if, and only if, z1 = z2 + 2kπi, where k is an integer Ch.3: Elementary Functions 3.2 The Exponential, Trigonometric, and Hyperbolic Functions 3.2.1 The Complex Function ez Polar Form of the Complex Function ez (Cont’d) The Theorem tell us ez is periodic with complex period 2πi If we divide up the z-plane into the infinite horizontal strips: Sn := {x + iy|−∞ <x< ∞,(2n − 1)π<y ≤ (2n + 1)π} where n = 0, ±1, ±2,... ez behaves in the same manner on each strip. Furthermore, ez is one-to-one on each strip Any one of these strips is called a fundamental region for ez Ch.3: Elementary Functions 3.2 The Exponential, Trigonometric, and Hyperbolic Functions 3.2.2 Trigonometric Functions Trigonometric Functions For real variables, we have the identities sin y = eiy − e−iy 2i , cos y = eiy + e−iy 2 We extend the identities to the complex case: Given any complex number z, we define sin z := eiz − e−iz 2i , cos z := eiz + e−iz 2 Since eiz and e−iz are entire functions, so are sin z and cos z. Some further identities remain valid in the complex case (See page 113)