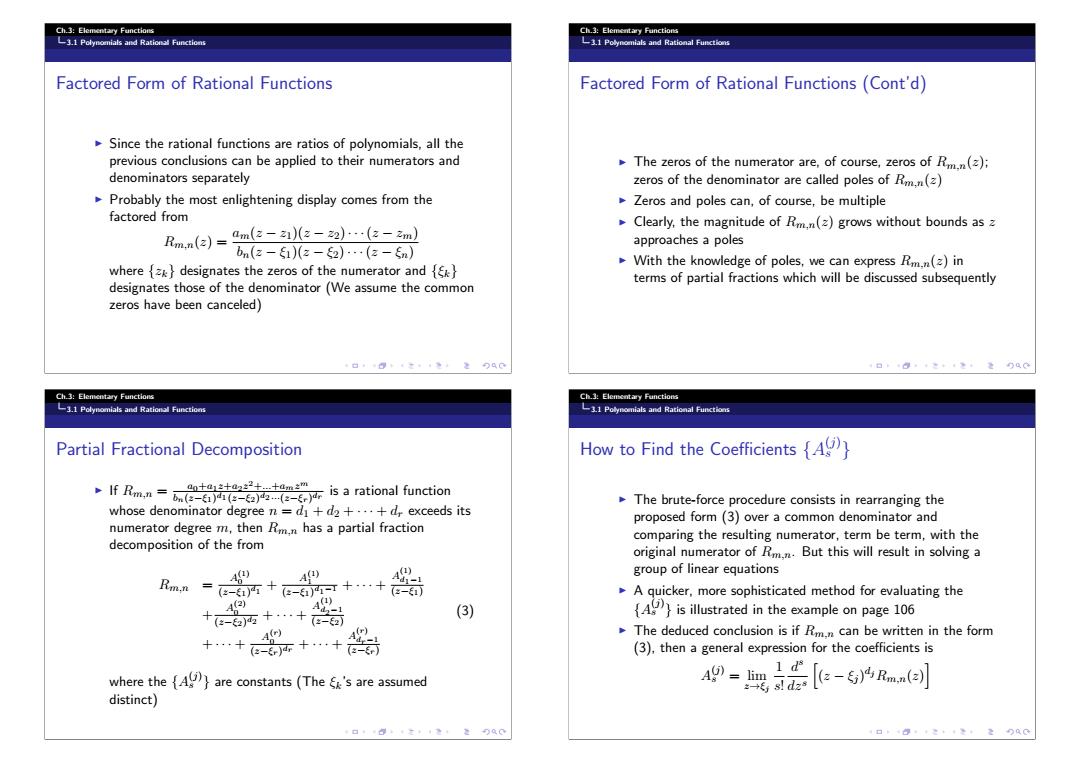
Ch.3:Elementary Functions Ch.3:Elementary Functions L3.1 Polynomials and Ration Functions L3.1 Polynomials and Rational Funsctions Factored Form of Rational Functions Factored Form of Rational Functions(Cont'd) Since the rational functions are ratios of polynomials,all the previous conclusions can be applied to their numerators and The zeros of the numerator are,of course,zeros of Rm.n(z); denominators separately zeros of the denominator are called poles of Rm.n(z) Probably the most enlightening display comes from the Zeros and poles can,of course,be multiple factored from Rmne)=m2-2-2)-e-2m Clearly,the magnitude of Rm.n(z)grows without bounds as z approaches a poles bn(z-E1)(z-2)…(z-En) where designates the zeros of the numerator and {E} With the knowledge of poles,we can express Rm.n(z)in terms of partial fractions which will be discussed subsequently designates those of the denominator(We assume the common zeros have been canceled) 白·0+之。，急，是2风C 4口0。+t+生+意0c Ch.3:Elementary Functions Ch.3:Elementary Functions 3.1 Polynomials and Rationol Functions 3.1 Polynomials and Rational Functions Partial Fractional Decomposition How to Find the Coefficients(A} a0十a1z+a2z2+..+dn2m IfR is a rational function The brute-force procedure consists in rearranging the whose denominator degree n=di+d2+...+d,exceeds its proposed form(3)over a common denominator and numerator degree m,then Rm.n has a partial fraction comparing the resulting numerator,term be term,with the decomposition of the from original numerator of Rm.n.But this will result in solving a 4 1) group of linear equations A quicker,more sophisticated method for evaluating the -1 +-a西+.…+司 (3) (A is illustrated in the example on page 106 十…十 F+…+-器 The deduced conclusion is if Rm.n can be written in the form (3).then a general expression for the coefficients is 1 ds[ where the A are constants(The 's are assumed -)Rmn(a创 A)=lim distinct) 口Ch.3: Elementary Functions 3.1 Polynomials and Rational Functions Factored Form of Rational Functions Since the rational functions are ratios of polynomials, all the previous conclusions can be applied to their numerators and denominators separately Probably the most enlightening display comes from the factored from Rm,n(z) = am(z − z1)(z − z2)···(z − zm) bn(z − ξ1)(z − ξ2)···(z − ξn) where {zk} designates the zeros of the numerator and {ξk} designates those of the denominator (We assume the common zeros have been canceled) Ch.3: Elementary Functions 3.1 Polynomials and Rational Functions Factored Form of Rational Functions (Cont’d) The zeros of the numerator are, of course, zeros of Rm,n(z); zeros of the denominator are called poles of Rm,n(z) Zeros and poles can, of course, be multiple Clearly, the magnitude of Rm,n(z) grows without bounds as z approaches a poles With the knowledge of poles, we can express Rm,n(z) in terms of partial fractions which will be discussed subsequently Ch.3: Elementary Functions 3.1 Polynomials and Rational Functions Partial Fractional Decomposition If Rm,n = a0+a1z+a2z2+...+amzm bn(z−ξ1)d1 (z−ξ2)d2 ···(z−ξr)dr is a rational function whose denominator degree n = d1 + d2 + ··· + dr exceeds its numerator degree m, then Rm,n has a partial fraction decomposition of the from Rm,n = A(1) 0 (z−ξ1)d1 + A(1) 1 (z−ξ1)d1−1 + ··· + A(1) d1−1 (z−ξ1) + A(2) 0 (z−ξ2)d2 + ··· + A(1) d2−1 (z−ξ2) + ··· + A(r) 0 (z−ξr)dr + ··· + A(r) dr−1 (z−ξr) (3) where the {A(j) s } are constants (The ξk’s are assumed distinct) Ch.3: Elementary Functions 3.1 Polynomials and Rational Functions How to Find the Coefficients {A(j) s } The brute-force procedure consists in rearranging the proposed form (3) over a common denominator and comparing the resulting numerator, term be term, with the original numerator of Rm,n. But this will result in solving a group of linear equations A quicker, more sophisticated method for evaluating the {A(j) s } is illustrated in the example on page 106 The deduced conclusion is if Rm,n can be written in the form (3), then a general expression for the coefficients is A(j) s = lim z→ξj 1 s! ds dzs (z − ξj )djRm,n(z)