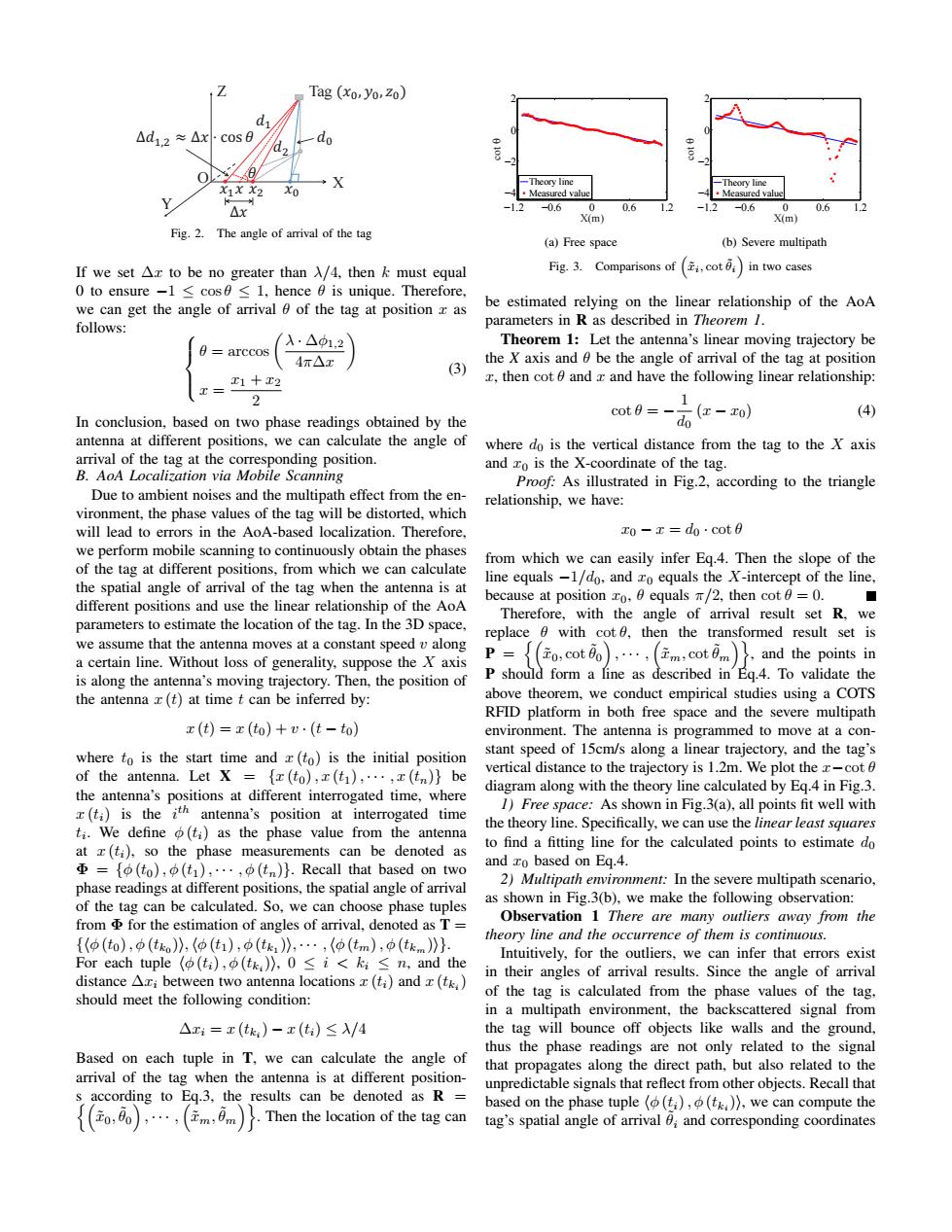
Z Tag (xo,yo,Zo) d △d1,2≈△xcos d, do 810 100 X -Theory line X1X X2 -Theory line Xo ·Measured value ·Measured value Y △x -12 -0.6 0 0.6 12 -12 -0.6 0 0.6 X(m) X(m) Fig.2.The angle of arrival of the tag (a)Free space (b)Severe multipath If we set Az to be no greater than A/4,then k must equal Fig.3. Comparisons of cot in two cases 0 to ensure -1 cos 1,hence 0 is unique.Therefore, we can get the angle of arrival of the tag at position x as be estimated relying on the linear relationship of the AoA follows: parameters in R as described in Theorem 1. 入·△1,2 Theorem 1:Let the antenna's linear moving trajectory be 0=arccos 4π△x the X axis and 0 be the angle of arrival of the tag at position (3) x1+E2 z,then cot and x and have the following linear relationship: T= 2 1 cot=- (x-x0) (4) In conclusion,based on two phase readings obtained by the do antenna at different positions,we can calculate the angle of where do is the vertical distance from the tag to the X axis arrival of the tag at the corresponding position. and zo is the X-coordinate of the tag. B.AoA Localization via Mobile Scanning Proof:As illustrated in Fig.2,according to the triangle Due to ambient noises and the multipath effect from the en- relationship,we have: vironment,the phase values of the tag will be distorted,which will lead to errors in the AoA-based localization.Therefore, x0-x=d0·cot0 we perform mobile scanning to continuously obtain the phases from which we can easily infer Eq.4.Then the slope of the of the tag at different positions,from which we can calculate the spatial angle of arrival of the tag when the antenna is at line equals-1/do,and zo equals the X-intercept of the line, because at position ro,8 equalsπ/2，then cot9=0.■ different positions and use the linear relationship of the AoA parameters to estimate the location of the tag.In the 3D space, Therefore,with the angle of arrival result set R,we replace 0 with cot,then the transformed result set is we assume that the antenna moves at a constant speed v along a certain line.Without loss of generality,suppose the X axis P={(io,coto）,…,(立m,cot Om),and the points in is along the antenna's moving trajectory.Then,the position of P should form a line as described in Eg.4.To validate the the antenna z(t)at time t can be inferred by: above theorem,we conduct empirical studies using a COTS RFID platform in both free space and the severe multipath x(t)=x(to)+v.(t-to) environment.The antenna is programmed to move at a con- where to is the start time and x(to)is the initial position stant speed of 15cm/s along a linear trajectory,and the tag's of the antenna.Let x {z(to),z(t1),...,x(tn)}be vertical distance to the trajectory is 1.2m.We plot the x-cot diagram along with the theory line calculated by Eg.4 in Fig.3. the antenna's positions at different interrogated time,where x(ti)is the ith antenna's position at interrogated time 1)Free space:As shown in Fig.3(a),all points fit well with ti.We define (ti)as the phase value from the antenna the theory line.Specifically,we can use the linear least squares to find a fitting line for the calculated points to estimate do at x(ti),so the phase measurements can be denoted as =o(to),(t),...,(n)}.Recall that based on two and zo based on Eq.4. phase readings at different positions,the spatial angle of arrival 2)Multipath environment:In the severe multipath scenario, as shown in Fig.3(b).we make the following observation: of the tag can be calculated.So,we can choose phase tuples from for the estimation of angles of arrival,denoted as T= Observation 1 There are many outliers away from the theory line and the occurrence of them is continuous. {(p(to),p(tk)》,(p(t1),p(tk)》,…,(p(tm),p(tkm)》}. Intuitively,for the outliers,we can infer that errors exist For each tuple(p(t),p(tk)》,0≤i<k≤n,and the distance Ar;between two antenna locations x(ti)and x(tk) in their angles of arrival results.Since the angle of arrival should meet the following condition: of the tag is calculated from the phase values of the tag, in a multipath environment,the backscattered signal from △x=x(tk)-x(t)≤λ/4 the tag will bounce off objects like walls and the ground, thus the phase readings are not only related to the signal Based on each tuple in T.we can calculate the angle of that propagates along the direct path,but also related to the arrival of the tag when the antenna is at different position-unpredictable signals that reflect from other objects.Recall that s according to Eq.3,the results can be denoted as R based on the phase tuple ((ti),()),we can compute the .Then the location of the tag can tag's spatial angle of arrivaland corresponding coordinatesߠ ଶݔ ݔଵݔ Tag ሺݔ଴ǡ ݕ଴ǡ ݖ଴ሻ ݀ଶ ݀ଵ ߠ •‘… ڄ ݔȟ ൎ ଵǡଶ݀ȟ ݔȟ X Y Z ଴ݔ ݀଴ O Fig. 2. The angle of arrival of the tag If we set ∆x to be no greater than λ/4, then k must equal 0 to ensure −1 ≤ cos θ ≤ 1, hence θ is unique. Therefore, we can get the angle of arrival θ of the tag at position x as follows:    θ = arccos ( λ · ∆ϕ1,2 4π∆x ) x = x1 + x2 2 (3) In conclusion, based on two phase readings obtained by the antenna at different positions, we can calculate the angle of arrival of the tag at the corresponding position. B. AoA Localization via Mobile Scanning Due to ambient noises and the multipath effect from the en￾vironment, the phase values of the tag will be distorted, which will lead to errors in the AoA-based localization. Therefore, we perform mobile scanning to continuously obtain the phases of the tag at different positions, from which we can calculate the spatial angle of arrival of the tag when the antenna is at different positions and use the linear relationship of the AoA parameters to estimate the location of the tag. In the 3D space, we assume that the antenna moves at a constant speed v along a certain line. Without loss of generality, suppose the X axis is along the antenna’s moving trajectory. Then, the position of the antenna x (t) at time t can be inferred by: x (t) = x (t0) + v · (t − t0) where t0 is the start time and x (t0) is the initial position of the antenna. Let X = {x (t0), x (t1), · · · , x (tn)} be the antenna’s positions at different interrogated time, where x (ti) is the i th antenna’s position at interrogated time ti . We define ϕ (ti) as the phase value from the antenna at x (ti), so the phase measurements can be denoted as Φ = {ϕ (t0), ϕ (t1), · · · , ϕ (tn)}. Recall that based on two phase readings at different positions, the spatial angle of arrival of the tag can be calculated. So, we can choose phase tuples from Φ for the estimation of angles of arrival, denoted as T = {⟨ϕ (t0), ϕ (tk0 )⟩,⟨ϕ (t1), ϕ (tk1 )⟩, · · · ,⟨ϕ (tm), ϕ (tkm)⟩}. For each tuple ⟨ϕ (ti), ϕ (tki )⟩, 0 ≤ i < ki ≤ n, and the distance ∆xi between two antenna locations x (ti) and x (tki ) should meet the following condition: ∆xi = x (tki ) − x (ti) ≤ λ/4 Based on each tuple in T, we can calculate the angle of arrival of the tag when the antenna is at different position￾s according to Eq.3, the results can be denoted as {( R = x˜0, ˜θ0 ) , · · · , ( x˜m, ˜θm )}. Then the location of the tag can −1.2 −0.6 0 0.6 1.2 −4 −2 0 2 X(m) cot θ Theory line Measured value (a) Free space −1.2 −0.6 0 0.6 1.2 −4 −2 0 2 X(m) cot θ Theory line Measured value (b) Severe multipath Fig. 3. Comparisons of ( x˜i, cot θ˜ i ) in two cases be estimated relying on the linear relationship of the AoA parameters in R as described in Theorem 1. Theorem 1: Let the antenna’s linear moving trajectory be the X axis and θ be the angle of arrival of the tag at position x, then cot θ and x and have the following linear relationship: cot θ = − 1 d0 (x − x0) (4) where d0 is the vertical distance from the tag to the X axis and x0 is the X-coordinate of the tag. Proof: As illustrated in Fig.2, according to the triangle relationship, we have: x0 − x = d0 · cot θ from which we can easily infer Eq.4. Then the slope of the line equals −1/d0, and x0 equals the X-intercept of the line, because at position x0, θ equals π/2, then cot θ = 0. Therefore, with the angle of arrival result set R, we replace θ with cot θ, then the transformed result set is P = {(x˜0, cot ˜θ0 ) , · · · , ( x˜m, cot ˜θm )}, and the points in P should form a line as described in Eq.4. To validate the above theorem, we conduct empirical studies using a COTS RFID platform in both free space and the severe multipath environment. The antenna is programmed to move at a con￾stant speed of 15cm/s along a linear trajectory, and the tag’s vertical distance to the trajectory is 1.2m. We plot the x−cot θ diagram along with the theory line calculated by Eq.4 in Fig.3. 1) Free space: As shown in Fig.3(a), all points fit well with the theory line. Specifically, we can use the linear least squares to find a fitting line for the calculated points to estimate d0 and x0 based on Eq.4. 2) Multipath environment: In the severe multipath scenario, as shown in Fig.3(b), we make the following observation: Observation 1 There are many outliers away from the theory line and the occurrence of them is continuous. Intuitively, for the outliers, we can infer that errors exist in their angles of arrival results. Since the angle of arrival of the tag is calculated from the phase values of the tag, in a multipath environment, the backscattered signal from the tag will bounce off objects like walls and the ground, thus the phase readings are not only related to the signal that propagates along the direct path, but also related to the unpredictable signals that reflect from other objects. Recall that based on the phase tuple ⟨ϕ (ti), ϕ (tki )⟩, we can compute the tag’s spatial angle of arrival ˜θi and corresponding coordinates