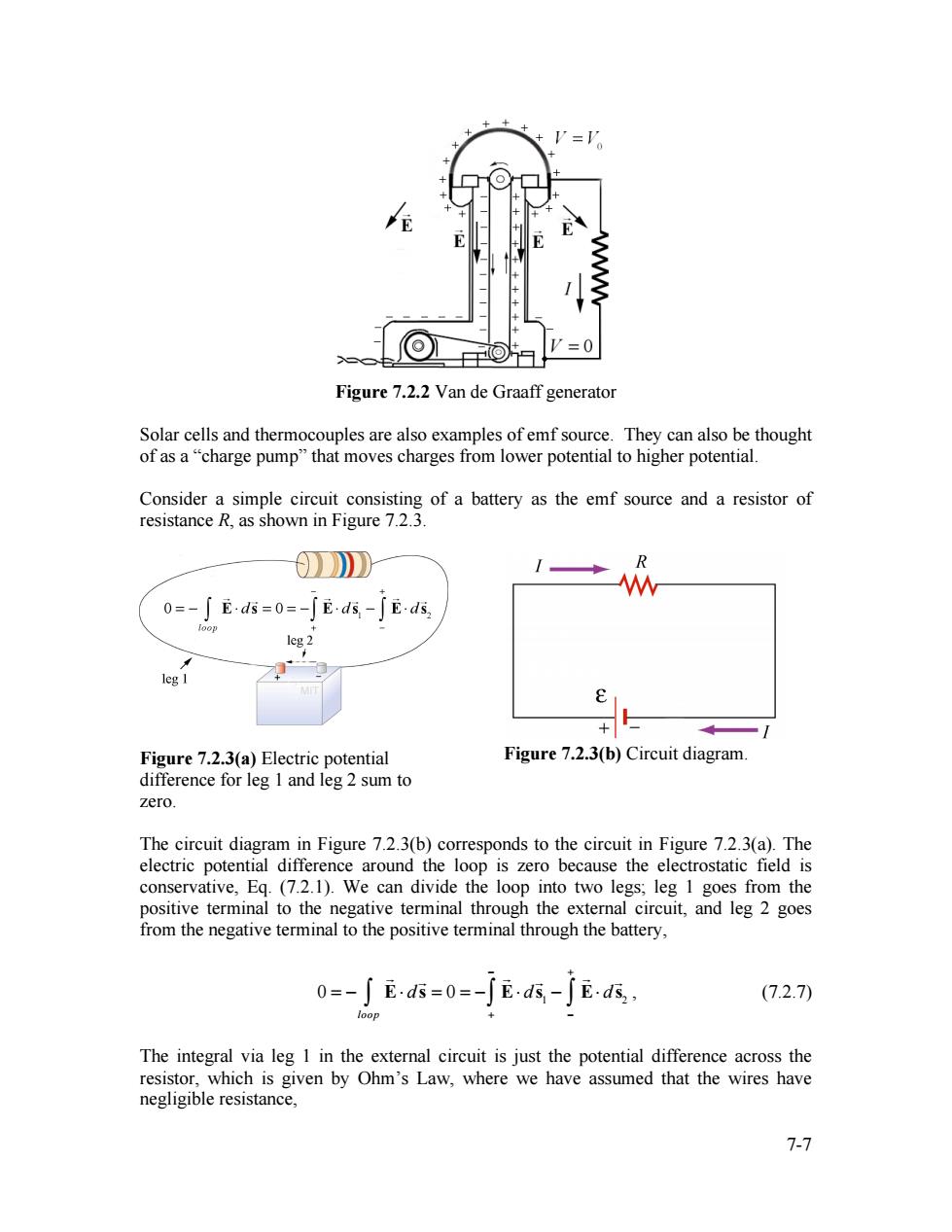
V=V V=0 Figure 7.2.2 Van de Graaff generator Solar cells and thermocouples are also examples of emf source.They can also be thought of as a"charge pump"that moves charges from lower potential to higher potential. Consider a simple circuit consisting of a battery as the emf source and a resistor of resistance R,as shown in Figure 7.2.3 D R 0=-∫Es=0=-jEs-jEs leg 2 leg 1 Figure 7.2.3(a)Electric potential Figure 7.2.3(b)Circuit diagram. difference for leg 1 and leg 2 sum to zero. The circuit diagram in Figure 7.2.3(b)corresponds to the circuit in Figure 7.2.3(a).The electric potential difference around the loop is zero because the electrostatic field is conservative,Eq.(7.2.1).We can divide the loop into two legs;leg 1 goes from the positive terminal to the negative terminal through the external circuit,and leg 2 goes from the negative terminal to the positive terminal through the battery, 0=-∫Es=0=-jEs-jEs (7.2.7) oop The integral via leg 1 in the external circuit is just the potential difference across the resistor,which is given by Ohm's Law,where we have assumed that the wires have negligible resistance, 7-77-7 Figure 7.2.2 Van de Graaff generator Solar cells and thermocouples are also examples of emf source. They can also be thought of as a “charge pump” that moves charges from lower potential to higher potential. Consider a simple circuit consisting of a battery as the emf source and a resistor of resistance R, as shown in Figure 7.2.3. Figure 7.2.3(a) Electric potential difference for leg 1 and leg 2 sum to zero. Figure 7.2.3(b) Circuit diagram. The circuit diagram in Figure 7.2.3(b) corresponds to the circuit in Figure 7.2.3(a). The electric potential difference around the loop is zero because the electrostatic field is conservative, Eq. (7.2.1). We can divide the loop into two legs; leg 1 goes from the positive terminal to the negative terminal through the external circuit, and leg 2 goes from the negative terminal to the positive terminal through the battery, 0 = −  E⋅ d  s = 0 loop ∫ = −  E⋅ d  s 1 + − ∫ −  E⋅ d  s2 − + ∫ , (7.2.7) The integral via leg 1 in the external circuit is just the potential difference across the resistor, which is given by Ohm’s Law, where we have assumed that the wires have negligible resistance