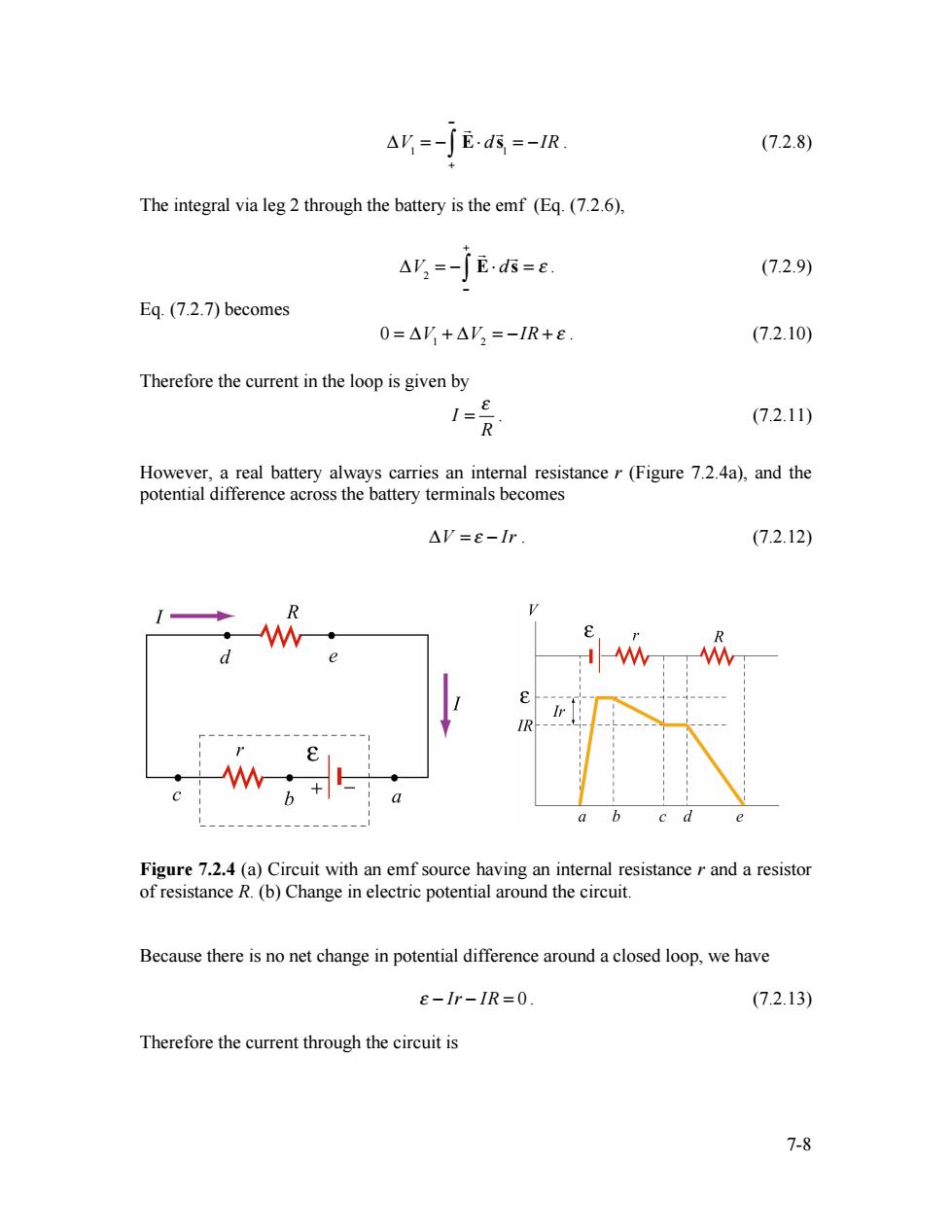
△r=-∫E.d=-lIR (7.2.8) The integral via leg 2 through the battery is the emf (Eq.(7.2.6), An--jE.di=e. (7.2.9) Eq.(7.2.7)becomes 0=△V+△V,=-IR+e. (7.2.10) Therefore the current in the loop is given by I=e (7.2.11) R However,a real battery always carries an internal resistance r(Figure 7.2.4a),and the potential difference across the battery terminals becomes △V=e-Ir. (7.2.12) d Ir IR b Figure 7.2.4 (a)Circuit with an emf source having an internal resistance r and a resistor of resistance R.(b)Change in electric potential around the circuit. Because there is no net change in potential difference around a closed loop,we have 8-Ir-IR=0. (7.2.13) Therefore the current through the circuit is 7-87-8 ΔV1 = −  E⋅ d  s 1 + − ∫ = −IR . (7.2.8) The integral via leg 2 through the battery is the emf (Eq. (7.2.6), ΔV2 = −  E⋅ d  s − + ∫ = ε . (7.2.9) Eq. (7.2.7) becomes 0 = ΔV1 + ΔV2 = −IR + ε . (7.2.10) Therefore the current in the loop is given by I R ε = . (7.2.11) However, a real battery always carries an internal resistance r (Figure 7.2.4a), and the potential difference across the battery terminals becomes ΔV = ε − Ir . (7.2.12) Figure 7.2.4 (a) Circuit with an emf source having an internal resistance r and a resistor of resistance R. (b) Change in electric potential around the circuit. Because there is no net change in potential difference around a closed loop, we have ε − Ir − IR = 0 . (7.2.13) Therefore the current through the circuit is