正在加载图片...
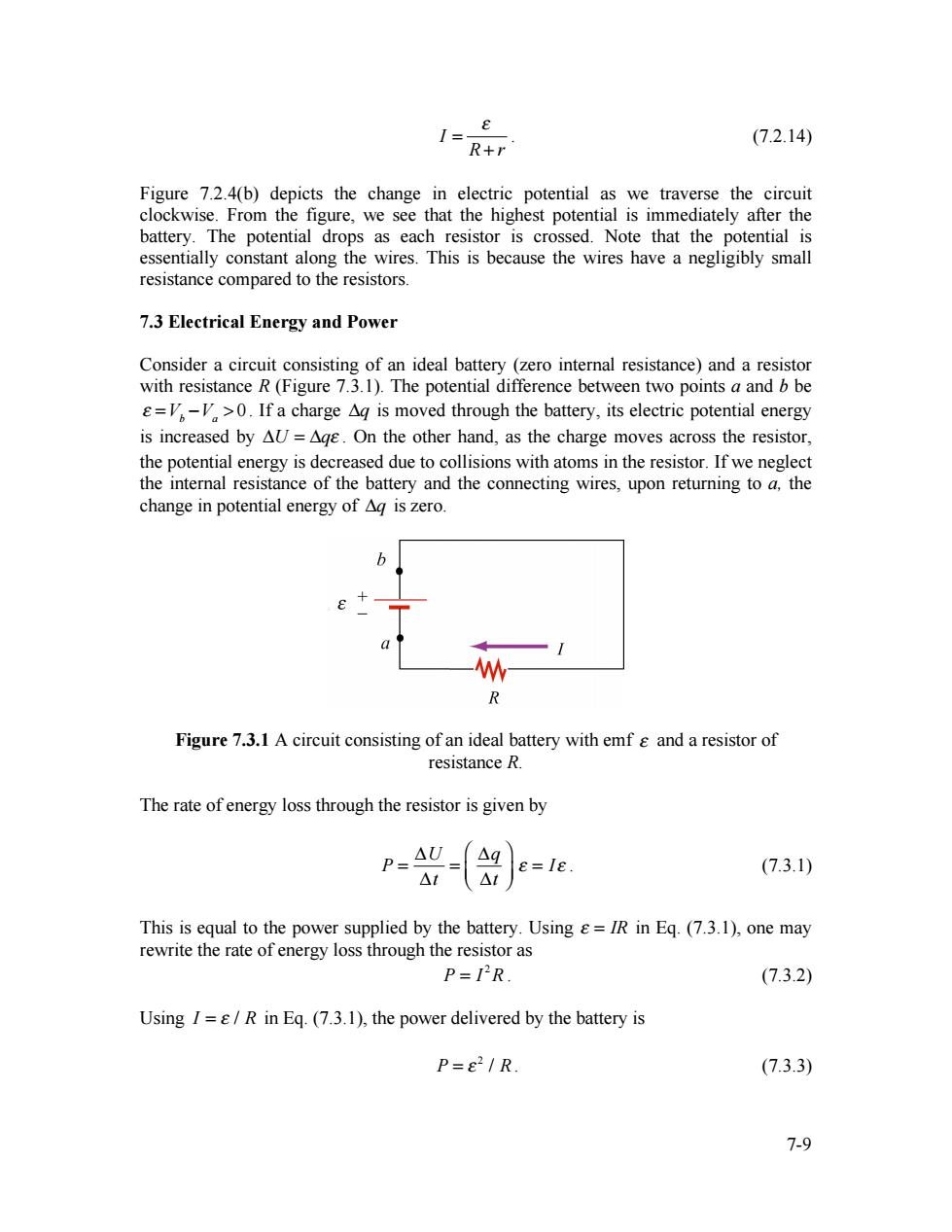
I= (7.2.14) R+r Figure 7.2.4(b)depicts the change in electric potential as we traverse the circuit clockwise.From the figure,we see that the highest potential is immediately after the battery.The potential drops as each resistor is crossed.Note that the potential is essentially constant along the wires.This is because the wires have a negligibly small resistance compared to the resistors. 7.3 Electrical Energy and Power Consider a circuit consisting of an ideal battery (zero internal resistance)and a resistor with resistance R(Figure 7.3.1).The potential difference between two points a and b be g=V-V>0.If a charge Ag is moved through the battery,its electric potential energy is increased by AU=Age.On the other hand,as the charge moves across the resistor, the potential energy is decreased due to collisions with atoms in the resistor.If we neglect the internal resistance of the battery and the connecting wires,upon returning to a,the change in potential energy of Ag is zero. R Figure 7.3.1 A circuit consisting of an ideal battery with emf g and a resistor of resistance R. The rate of energy loss through the resistor is given by P= △U △ (7.3.1) △1△1 This is equal to the power supplied by the battery.Using &=IR in Eq.(7.3.1),one may rewrite the rate of energy loss through the resistor as P=IR (7.3.2) Using I=8/R in Eq.(7.3.1),the power delivered by the battery is P=82/R (7.3.3) 7-97-9 I R r ε = + . (7.2.14) Figure 7.2.4(b) depicts the change in electric potential as we traverse the circuit clockwise. From the figure, we see that the highest potential is immediately after the battery. The potential drops as each resistor is crossed. Note that the potential is essentially constant along the wires. This is because the wires have a negligibly small resistance compared to the resistors. 7.3 Electrical Energy and Power Consider a circuit consisting of an ideal battery (zero internal resistance) and a resistor with resistance R (Figure 7.3.1). The potential difference between two points a and b be ε =Vb −Va > 0 . If a charge Δq is moved through the battery, its electric potential energy is increased by ΔU = Δqε . On the other hand, as the charge moves across the resistor, the potential energy is decreased due to collisions with atoms in the resistor. If we neglect the internal resistance of the battery and the connecting wires, upon returning to a, the change in potential energy of Δq is zero. Figure 7.3.1 A circuit consisting of an ideal battery with emf ε and a resistor of resistance R. The rate of energy loss through the resistor is given by P = ΔU Δt = Δq Δt ⎛ ⎝ ⎜ ⎞ ⎠ ⎟ ε = Iε . (7.3.1) This is equal to the power supplied by the battery. Using ε = IR in Eq. (7.3.1), one may rewrite the rate of energy loss through the resistor as P = I 2 R . (7.3.2) Using I = ε / R in Eq. (7.3.1), the power delivered by the battery is P = ε2 / R. (7.3.3)