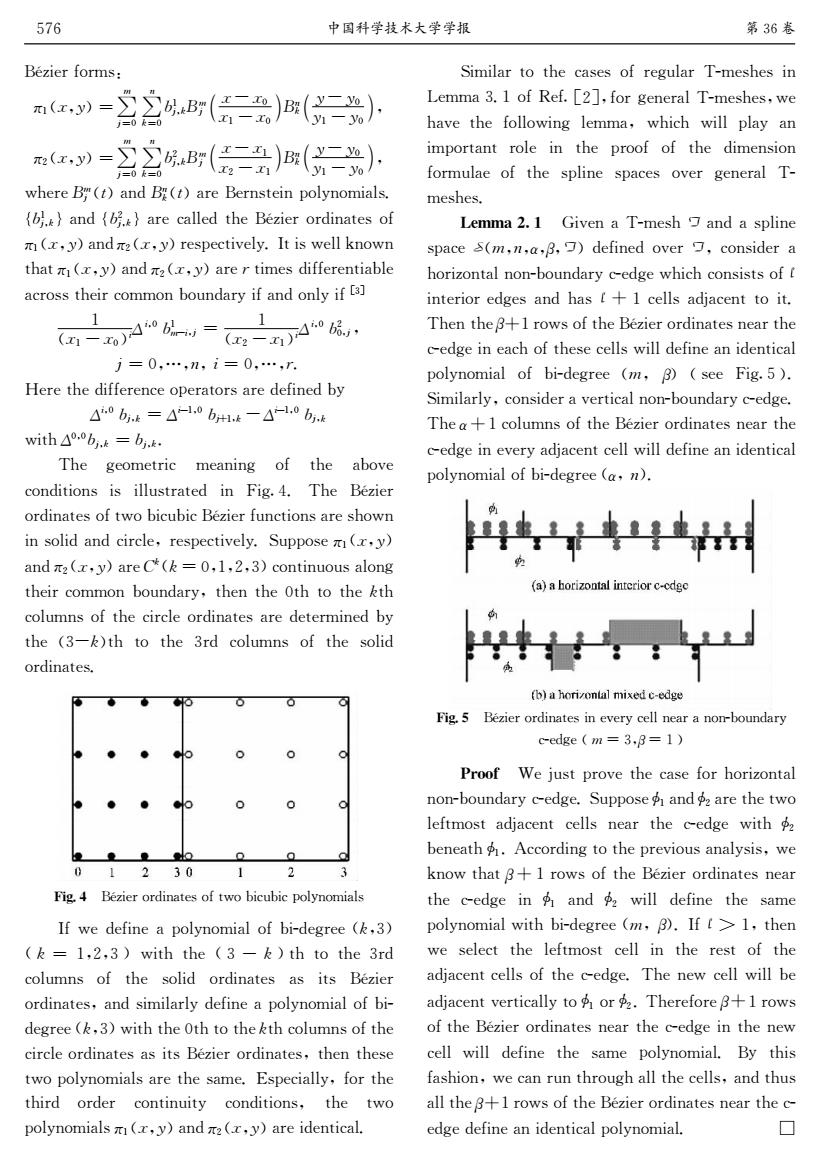
576 中回科学徒术大零学摄 第36表 Bezier forms: Similar to the caxes of regular T-meshes in Lemma 3.1 of Ref.[2],for general T-meshes,we have the following lemma.which will play an -含2r信（货 important role in the proof of the dimension formulae of the spline spaces over general T- where B(t)and B()are Bernstein polynomials. meshes. {以，.】and{.}are called the Bezier ordinates of Lemma 2.I Given a T-mesh and a spline m(r.y)and(r.y)respertively.It is well known space s(m,i,a,3,)defined over了，consider a that(.c,y)and (c,y)are r times differentiable horizontal non-boundary cedge which consists of I across their commo boundary if and ony if interior edges and has !+I cells adjacent to it. -n20n4成· Then the +1 rows of the Bezier ordinates near the edge in each of these cells will define an identical j=0,…,,了=0，…， polynomial of bi-degree (m.see Fig.5). Here the difference operators are defined by Similarly,consider a vertical notrboundary cedge. 4b4=△-10644-△-10bc The a+1 columns of the Bezier ordinates near the with△0.h.t=hk cedge in every adjacent cell will define an identical The geometric meaning of the above polynomial of bi-degree (a,n). conditions is illustrated in Fig.4.The Bezier ordinates of two birubic Bezier functions are shown in solid and circle,respectively.Suppose m.y) and (c.y)are C(-0.1.2,3)continuous along 件m their common boundary,then the Oth to the kth columns of the circle ordinates are determined by the (3-)th to the 3rd columns of the solid ordinates. b)a hrmyuntal mived c-edpe Fig.5 Bezier ordntes in every cvll meur s nourboundary c-edge (-3.8-1) Proof We just prove the case for horizontal non-boundary c-edge.Suppose$andf:are the two leftmost adjacent cells near the eedge with beneath According to the previous analysis,we 01 1301 know that a+1 rows of the Bezier ordinates near Fig 4 Birier ordirates of two bicuhic polynommials the c-edge in and will define the same If we define a polynomial of hi-degree (,3) polynomial with bi-degree (m.B).If 1,then (1,2.3 with the (3-)th to the 3rd we select the leftmost cell in the rest of the columns of the solid ordinates as its Bezier adjacent cells of the cedge.The new cell will be ordinates,and similarly define a polynomial of bi- adjacent vertically to or.Therefore 8+1 rows degree (.3)with the Oth to the tth columns of the of the Bezier ordinates near the cedge in the new circle ordinates as its Bezier ordinates,then these cell will define the same polynomial.By this two polynomials are the same.Especially.for the fashion,we can nm through all the cells,and thus third order continuity conditions,the two all the +1 rows of the Bezier ordinates near the e polynomials(r.y)and ga(r,y)are identical edge define an identical polypomial. ▣NPQ02.O$.IC! %S">#2$;% ’ @;)% ( A;) :S @#AB’ @ >C>) ">S C>)$B( A 2C2) "2S C2)$# %(">#2$;% ’ @;)% ( A;) :( @#AB’ @ >C>S ">( C>S$B( A 2C2) "2S C2)$# K>2.2B’ @ "&$?,JB( A"&$?.2N2.,C/20,G$%U,$I0?%C& %:S @#A&?,J%:( @#A&?.21?%%2J/>2NPQ02.$.J0,?/2C$O %S">#2$?,J%(">#2$.2CG21/0H2%U&3/0CK2%%[,$K, />?/%S">#2$?,J%(">#2$?.2%/0I2CJ0OO2.2,/0?M%2 ?1.$CC/>20.1$II$,M$+,J?.U0O?,J$,%U0O’!( S ">S C>)$-&-#):S ’C-#@ ; S ">( C>S$-&-#):( )#@# @;)#)#(#-;)#)#%< :2.2/>2J0OO2.2,12$G2.?/$.C?.2J2O0,2JMU &-#):@#A ;&-CS#):@DS#A C&-CS#):@#A K0/>&)#) :@#A ;:@#A< F>2 @2$I2/.01 I2?,0,@ $O />2 ?M$H2 1$,J0/0$,C0C0%%+C/.?/2J 0, \0@YT& F>2 NPQ02. $.J0,?/2C$O/K$M01+M01NPQ02.O+,1/0$,C?.2C>$K, 0,C$%0J?,J10.1%2#.2CG21/0H2%U&W+GG$C2%S">#2$ ?,J%(">#2$?.28A"A;)#S#(#!$1$,/0,+$+C?%$,@ />20.1$II$,M$+,J?.U#/>2,/>2)/>/$/>2A/> 1$%+I,C$O/>210.1%2$.J0,?/2C?.2J2/2.I0,2JMU />2 "!cA$/>/$/>2!.J 1$%+I,C $O/>2C$%0J $.J0,?/2C& D"/GI NPQ02.$.J0,?/2C$O/K$M01+M01G$%U,$I0?%C 3OK2J2O0,2?G$%U,$I0?%$OM06J2@.22"A#!$ "A ; S#(#!$K0/>/>2 "!CA$/>/$/>2!.J 1$%+I,C $O />2 C$%0J $.J0,?/2C ?C 0/C NPQ02. $.J0,?/2C#?,JC0I0%?.%UJ2O0,2?G$%U,$I0?%$OM06 J2@.22"A#!$K0/>/>2)/>/$/>2A/>1$%+I,C$O/>2 10.1%2$.J0,?/2C?C0/CNPQ02.$.J0,?/2C#/>2,/>2C2 /K$G$%U,$I0?%C?.2/>2C?I2&BCG210?%%U#O$./>2 />0.J $.J2. 1$,/0,+0/U 1$,J0/0$,C# />2 /K$ G$%U,$I0?%C%S">#2$?,J%(">#2$?.20J2,/01?%& W0I0%?./$/>21?C2C$O.2@+%?.F6I2C>2C0, D2II?!&S$OX2OY’((#O$.@2,2.?%F6I2C>2C#K2 >?H2/>2O$%%$K0,@%2II?#K>01> K0%%G%?U?, 0IG$./?,/.$%20, />2 G.$$O $O/>2 J0I2,C0$, O$.I+%?2$O/>2CG%0,2CG?12C $H2. @2,2.?% F6 I2C>2C& ;$##+@JF <0H2,?F6I2C>!?,J?CG%0,2 CG?12#"’#(#"###!$J2O0,2J$H2.!#1$,C0J2.? >$.0Q$,/?%,$,6M$+,J?.U162J@2K>01>1$,C0C/C$O% 0,/2.0$.2J@2C?,J>?C%DS12%%C?JA?12,//$0/& F>2,/>2#DS.$KC$O/>2NPQ02.$.J0,?/2C,2?./>2 162J@20,2?1>$O/>2C212%%CK0%%J2O0,2?,0J2,/01?% G$%U,$I0?%$O M06J2@.22 "’##$ "C22 \0@Y5$& W0I0%?.%U#1$,C0J2.?H2./01?%,$,6M$+,J?.U162J@2& F>2"DS1$%+I,C$O/>2NPQ02.$.J0,?/2C,2?./>2 162J@20,2H2.U?JA?12,/12%%K0%%J2O0,2?,0J2,/01?% G$%U,$I0?%$OM06J2@.22""#($< D"/GK NPQ02.$.J0,?/2C0,2H2.U12%%,2?.?,$,6M$+,J?.U 162J@2"’ ;!##;S$ L.’’( L2A+C/G.$H2/>21?C2O$.>$.0Q$,/?% ,$,6M$+,J?.U162J@2&W+GG$C2$S?,J$(?.2/>2/K$ %2O/I$C/?JA?12,/12%%C,2?./>2162J@2 K0/>$( M2,2?/>$S<-11$.J0,@/$/>2G.2H0$+C?,?%UC0C#K2 [,$K/>?/#DS.$KC$O/>2NPQ02.$.J0,?/2C,2?. />2162J@20,$S ?,J$( K0%% J2O0,2 />2 C?I2 G$%U,$I0?%K0/>M06J2@.22"’##$<3O%&S#/>2, K2C2%21//>2%2O/I$C/12%%0,/>2.2C/$O/>2 ?JA?12,/12%%C$O/>2162J@2&F>2,2K12%%K0%%M2 ?JA?12,/H2./01?%%U/$$S$.$(<F>2.2O$.2#DS.$KC $O/>2NPQ02.$.J0,?/2C,2?./>2162J@20,/>2,2K 12%% K0%%J2O0,2/>2 C?I2 G$%U,$I0?%&NU />0C O?C>0$,#K21?,.+,/>.$+@>?%%/>212%%C#?,J/>+C ?%%/>2#DS.$KC$O/>2NPQ02.$.J0,?/2C,2?./>216 2J@2J2O0,2?,0J2,/01?%G$%U,$I0?%& ’ 57" CDEFGHIFFJ K!"L