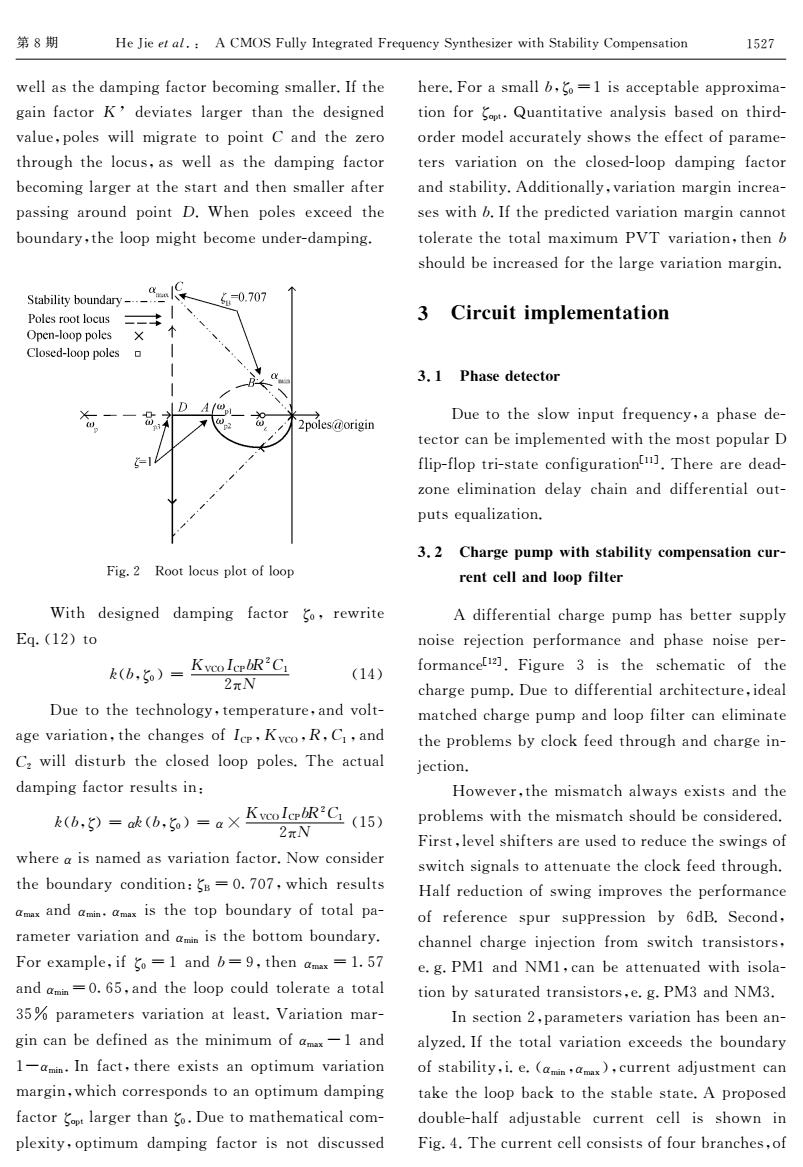
第8期 He Jie et al.A CMOS Fully Integrated Frequency Synthesizer with Stability Compensation 1527 well as the damping factor becoming smaller.If the here.For a small b,o=1 is acceptable approxima- gain factor K'deviates larger than the designed tion for Quantitative analysis based on third- value,poles will migrate to point C and the zero order model accurately shows the effect of parame- through the locus,as well as the damping factor ters variation on the closed-loop damping factor becoming larger at the start and then smaller after and stability.Additionally,variation margin increa- passing around point D.When poles exceed the ses with b.If the predicted variation margin cannot boundary,the loop might become under-damping. tolerate the total maximum PVT variation,then b should be increased for the large variation margin. Stability boundary- -0.707 Poles root locus 3 Circuit implementation Open-loop poles X Closed-loop poles 3.1 Phase detector Due to the slow input frequency,a phase de- 2polesaorigin tector can be implemented with the most popular D flip-flop tri-state configuration There are dead- zone elimination delay chain and differential out- puts equalization. 3.2 Charge pump with stability compensation cur- Fig.2 Root locus plot of loop rent cell and loop filter With designed damping factor to,rewrite A differential charge pump has better supply Eq.(12)to noise rejection performance and phase noise per- k(b,5o)= Kvco ICpbR2C (14) formance[iz.Figure 3 is the schematic of the 2πN charge pump.Due to differential architecture,ideal Due to the technology,temperature,and volt- matched charge pump and loop filter can eliminate age variation,the changes of Icp,Kvco,R,C,and the problems by clock feed through and charge in- Ca will disturb the closed loop poles.The actual jection. damping factor results in: However,the mismatch always exists and the k(b,0=k(b,a)=a×KveoleRC（a5) problems with the mismatch should be considered. 2πN First,level shifters are used to reduce the swings of where a is named as variation factor.Now consider switch signals to attenuate the clock feed through. the boundary condition:8=0.707,which results Half reduction of swing improves the performance amax and amin.amax is the top boundary of total pa- of reference spur suppression by 6dB.Second, rameter variation and amin is the bottom boundary. channel charge injection from switch transistors, For example,if $o=1 and b=9,then amax =1.57 e.g.PMI and NM1,can be attenuated with isola- and amin=0.65,and the loop could tolerate a total tion by saturated transistors,e.g.PM3 and NM3. 35%parameters variation at least.Variation mar- In section 2,parameters variation has been an- gin can be defined as the minimum of amax-1 and alyzed.If the total variation exceeds the boundary 1-amin.In fact,there exists an optimum variation of stability,i.e.(amin,amax),current adjustment can margin,which corresponds to an optimum damping take the loop back to the stable state.A proposed factor opt larger than o.Due to mathematical com- double-half adjustable current cell is shown in plexity,optimum damping factor is not discussed Fig.4.The current cell consists of four branches,of第&期 I=.8=9=+>S( *)AW,UN99L(?F=JD7F=RUD=^N=?:L,L?FG=<8]=D@8FG,F7K898FL)E6M=?<7F8E? @=997<FG=R76M8?JH7:FEDK=:E68?J<6799=D>(HFG= J78?H7:FEDN*R=X87F=<97DJ=DFG7?FG=R=<8J?=R X79N=$ME9=<@89968JD7F=FEME8?FA 7?RFG=]=DE FGDENJGFG=9E:N<$7<@=997<FG=R76M8?JH7:FED K=:E68?J97DJ=D7FFG=<F7DF7?RFG=?<6799=D7HF=D M7<<8?J7DEN?R ME8?F:>SG=? ME9=<=\:==RFG= KEN?R7DL$FG=9EEM68JGFK=:E6=N?R=D0R76M8?J> U8J>! ZEEF9E:N<M9EFEH9EEM S8FG R=<8J?=R R76M8?J H7:FED +"$D=@D8F= ’^>%$!&FE L%H$+"&V NV)WT)-HB! A$ !(G %$2& 1N=FEFG=F=:G?E9EJL$F=6M=D7FND=$7?RXE9F0 7J=X7D87F8E?$FG=:G7?J=<EHT)-$NV)W$B$A$$7?R A! @899R8<FNDKFG=:9E<=R9EEMME9=<>/G=7:FN79 R76M8?JH7:FEDD=<N9F<8?( L%H$+&V)L%H$+"&V)[NV)WT)-HB! A$ !(G %$5& @G=D=)8<?76=R7<X7D87F8E?H7:FED>+E@:E?<8R=D FG=KEN?R7DL:E?R8F8E?(+B g"‘3"3$@G8:GD=<N9F< )67\7?R)68?>)67\8<FG=FEMKEN?R7DLEHFEF79M70 D76=F=DX7D87F8E?7?R)68?8<FG=KEFFE6KEN?R7DL> UED=\76M9=$8H+" g$7?RHg%$FG=?)67\ g$‘53 7?R)68?g"‘#5$7?RFG=9EEM:EN9RFE9=D7F=7FEF79 45h M7D76=F=D<X7D87F8E?7F9=7<F>V7D87F8E? 67D0 J8?:7?K=R=H8?=R7<FG=68?86N6EH)67\_$7?R $_)68?>(?H7:F$FG=D==\8<F<7?EMF86N6 X7D87F8E? 67DJ8?$@G8:G:EDD=<ME?R<FE7?EMF86N6R76M8?J H7:FED+EMF97DJ=DFG7?+">1N=FE67FG=67F8:79:E60 M9=\8FL$EMF86N6 R76M8?JH7:FED8<?EFR8<:N<<=R G=D=>UED7<6799H$+"g$8<7::=MF7K9=7MMDE\8670 F8E?HED+EMF>[N7?F8F7F8X=7?79L<8<K7<=RE?FG8DR0 EDR=D6ER=97::ND7F=9L<GE@<FG==HH=:FEHM7D76=0 F=D<X7D87F8E? E?FG=:9E<=R09EEM R76M8?JH7:FED 7?R<F7K898FL>*RR8F8E?799L$X7D87F8E?67DJ8?8?:D=70 <=<@8FGH>(HFG=MD=R8:F=RX7D87F8E?67DJ8?:7??EF FE9=D7F=FG=FEF7967\86N6 -V/X7D87F8E?$FG=?H <GEN9RK=8?:D=7<=RHEDFG=97DJ=X7D87F8E?67DJ8?> T 0JAN<J?JRD:@R@G?C?J9G TYE BHC>@L@?@N?9A 1N=FEFG=<9E@8?MNFHD=^N=?:L$7MG7<=R=0 F=:FED:7?K=86M9=6=?F=R@8FGFG=6E<FMEMN97D1 H98M0H9EMFD80<F7F=:E?H8JND7F8E?-$$. >/G=D=7D=R=7R0 ]E?==9868?7F8E?R=97L:G78?7?RR8HH=D=?F879ENF0 MNF<=^N798]7F8E?> TY+ 0HCA=@D<RDPJ?H>?CKJ:J?IN9RD@G>C?J9GN<AW A@G?N@::CGL:99DMJ:?@A *R8HH=D=?F879:G7DJ=MN6MG7<K=FF=D<NMM9L ?E8<=D=;=:F8E?M=DHED67?:=7?RMG7<=?E8<=M=D0 HED67?:=-$!. >U8JND= 4 8< FG= <:G=67F8: EH FG= :G7DJ=MN6M>1N=FER8HH=D=?F8797D:G8F=:FND=$8R=79 67F:G=R:G7DJ=MN6M7?R9EEMH89F=D:7?=9868?7F= FG=MDEK9=6<KL:9E:aH==RFGDENJG7?R:G7DJ=8?0 ;=:F8E?> IE@=X=D$FG=68<67F:G79@7L<=\8<F<7?RFG= MDEK9=6<@8FGFG=68<67F:G<GEN9RK=:E?<8R=D=R> U8D<F$9=X=9<G8HF=D<7D=N<=RFED=RN:=FG=<@8?J<EH <@8F:G<8J?79<FE7FF=?N7F=FG=:9E:aH==RFGDENJG> I79HD=RN:F8E?EH<@8?J86MDEX=<FG=M=DHED67?:= EHD=H=D=?:= <MND <NMMD=<<8E? KL #RB>,=:E?R$ :G7??=9:G7DJ=8?;=:F8E?HDE6 <@8F:GFD7?<8<FED<$ =>J>-A$7?R+A$$:7?K=7FF=?N7F=R@8FG8<E970 F8E?KL<7FND7F=RFD7?<8<FED<$=>J>-A47?R+A4> (?<=:F8E?!$M7D76=F=D<X7D87F8E?G7<K==?7?0 79L]=R>(HFG=FEF79X7D87F8E?=\:==R<FG=KEN?R7DL EH<F7K898FL$8>=>%)68?$)67\&$:NDD=?F7R;N<F6=?F:7? F7a=FG=9EEMK7:aFEFG=<F7K9=<F7F=>*MDEME<=R RENK9=0G79H7R;N<F7K9= :NDD=?F :=998< <GE@? 8? U8J>2>/G=:NDD=?F:=99:E?<8<F<EHHENDKD7?:G=<$EH $5!3