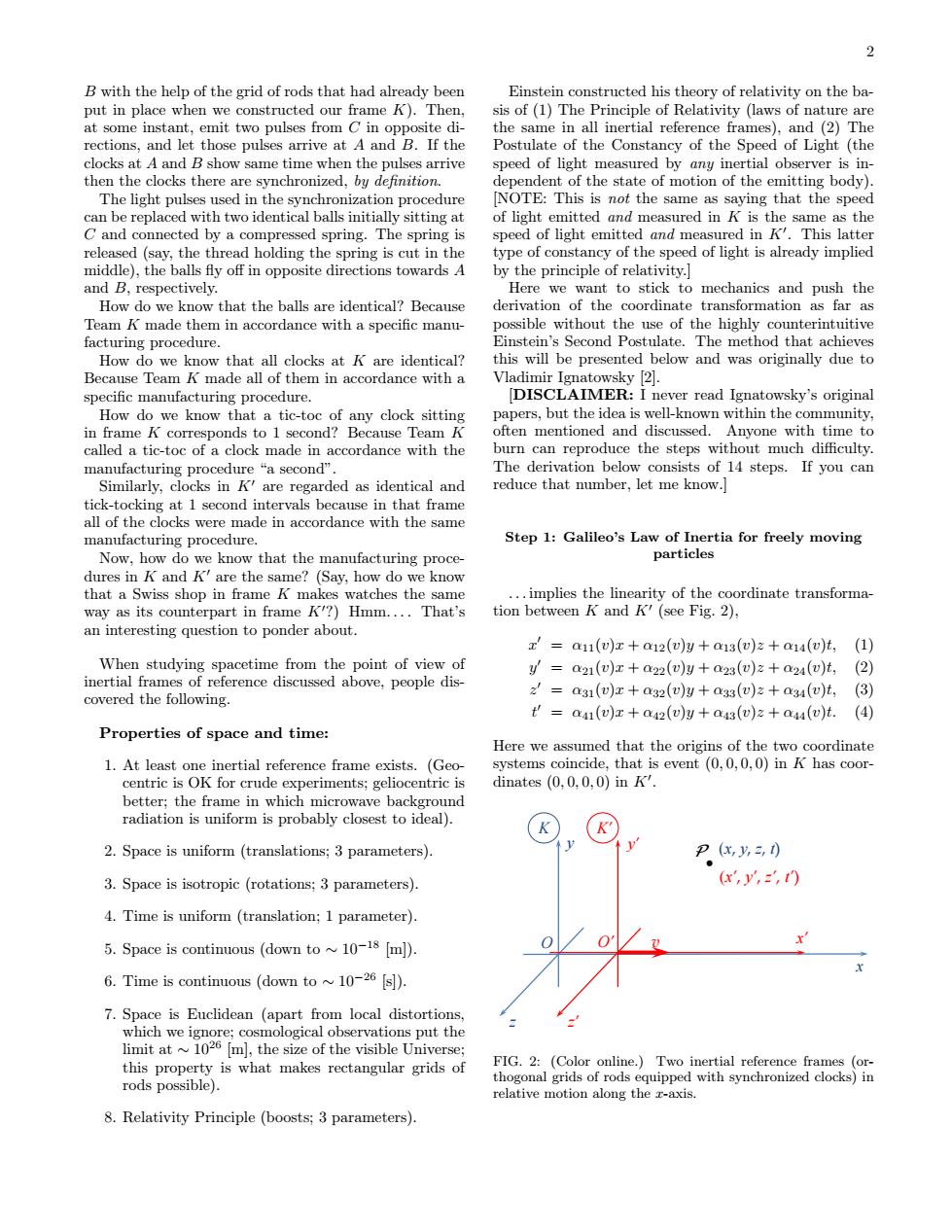
2 B with the help of the grid of rods that had already been Einstein constructed his theory of relativity on the ba- put in place when we constructed our frame K).Then, sis of(1)The Principle of Relativity (laws of nature are at some instant,emit two pulses from C in opposite di- the same in all inertial reference frames).and (2)The rections,and let those pulses arrive at A and B.If the Postulate of the Constancy of the Speed of Light (the clocks at A and B show same time when the pulses arrive speed of light measured by any inertial observer is in- then the clocks there are synchronized,by definition. dependent of the state of motion of the emitting body). The light pulses used in the synchronization procedure NOTE:This is not the same as saying that the speed can be replaced with two identical balls initially sitting at of light emitted and measured in K is the same as the C and connected by a compressed spring.The spring is speed of light emitted and measured in K'.This latter released(say,the thread holding the spring is cut in the type of constancy of the speed of light is already implied middle),the balls fly off in opposite directions towards A by the principle of relativity. and B,respectively. Here we want to stick to mechanics and push the How do we know that the balls are identical?Because derivation of the coordinate transformation as far as Team K made them in accordance with a specific manu- possible without the use of the highly counterintuitive facturing procedure. Einstein's Second Postulate.The method that achieves How do we know that all clocks at K are identical? this will be presented below and was originally due to Because Team K made all of them in accordance with a Vladimir Ignatowsky [2. specific manufacturing procedure. DISCLAIMER:I never read Ignatowsky's original How do we know that a tic-toc of any clock sitting papers,but the idea is well-known within the community, in frame K corresponds to 1 second?Because Team K often mentioned and discussed.Anyone with time to called a tic-toc of a clock made in accordance with the burn can reproduce the steps without much difficulty. manufacturing procedure "a second" The derivation below consists of 14 steps.If you can Similarly,clocks in K'are regarded as identical and reduce that number,let me know. tick-tocking at 1 second intervals because in that frame all of the clocks were made in accordance with the same manufacturing procedure. Step 1:Galileo's Law of Inertia for freely moving Now,how do we know that the manufacturing proce- particles dures in K and K'are the same?(Say,how do we know that a Swiss shop in frame k makes watches the same ..implies the linearity of the coordinate transforma- way as its counterpart in frame K?)Hmm....That's tion between K and K'(see Fig.2), an interesting question to ponder about. x'=a11(v)z+a12(v)y+a13(v)z+a14(v)t,(1) When studying spacetime from the point of view of =a21(v)z+a22()y+a23()z+a24(v)t,(2) inertial frames of reference discussed above,people dis- covered the following. 2=a31(v)z+a32(v)y+a33(v)z+a31(v)t,(3) t=a41(v)z+a42(v)y+a43()z+a44(v)t.(4) Properties of space and time: Here we assumed that the origins of the two coordinate 1.At least one inertial reference frame exists.(Geo- systems coincide,that is event(0,0,0,0)in K has coor- centric is OK for crude experiments;geliocentric is dinates (0,0,0,0)in K'. better:the frame in which microwave background radiation is uniform is probably closest to ideal). 2.Space is uniform (translations;3 parameters). P(x,y三，) 3.Space is isotropic (rotations;3 parameters). (x',y,,1门 4.Time is uniform (translation;1 parameter). 5.Space is continuous (down to ~10-18 [m]). 6.Time is continuous (down to ~10-26 [s]) 7.Space is Euclidean (apart from local distortions, which we ignore:cosmological observations put the limit at~1026 [m],the size of the visible Universe; this property is what makes rectangular grids of FIG.2:(Color online.)Two inertial reference frames (or- rods possible) thogonal grids of rods equipped with synchronized clocks)in relative motion along the z-axis. 8.Relativity Principle (boosts;3 parameters).2 B with the help of the grid of rods that had already been put in place when we constructed our frame K). Then, at some instant, emit two pulses from C in opposite di￾rections, and let those pulses arrive at A and B. If the clocks at A and B show same time when the pulses arrive then the clocks there are synchronized, by definition. The light pulses used in the synchronization procedure can be replaced with two identical balls initially sitting at C and connected by a compressed spring. The spring is released (say, the thread holding the spring is cut in the middle), the balls fly off in opposite directions towards A and B, respectively. How do we know that the balls are identical? Because Team K made them in accordance with a specific manu￾facturing procedure. How do we know that all clocks at K are identical? Because Team K made all of them in accordance with a specific manufacturing procedure. How do we know that a tic-toc of any clock sitting in frame K corresponds to 1 second? Because Team K called a tic-toc of a clock made in accordance with the manufacturing procedure “a second”. Similarly, clocks in K0 are regarded as identical and tick-tocking at 1 second intervals because in that frame all of the clocks were made in accordance with the same manufacturing procedure. Now, how do we know that the manufacturing proce￾dures in K and K0 are the same? (Say, how do we know that a Swiss shop in frame K makes watches the same way as its counterpart in frame K0 ?) Hmm. . . . That’s an interesting question to ponder about. When studying spacetime from the point of view of inertial frames of reference discussed above, people dis￾covered the following. Properties of space and time: 1. At least one inertial reference frame exists. (Geo￾centric is OK for crude experiments; geliocentric is better; the frame in which microwave background radiation is uniform is probably closest to ideal). 2. Space is uniform (translations; 3 parameters). 3. Space is isotropic (rotations; 3 parameters). 4. Time is uniform (translation; 1 parameter). 5. Space is continuous (down to ∼ 10−18 [m]). 6. Time is continuous (down to ∼ 10−26 [s]). 7. Space is Euclidean (apart from local distortions, which we ignore; cosmological observations put the limit at ∼ 1026 [m], the size of the visible Universe; this property is what makes rectangular grids of rods possible). 8. Relativity Principle (boosts; 3 parameters). Einstein constructed his theory of relativity on the ba￾sis of (1) The Principle of Relativity (laws of nature are the same in all inertial reference frames), and (2) The Postulate of the Constancy of the Speed of Light (the speed of light measured by any inertial observer is in￾dependent of the state of motion of the emitting body). [NOTE: This is not the same as saying that the speed of light emitted and measured in K is the same as the speed of light emitted and measured in K0 . This latter type of constancy of the speed of light is already implied by the principle of relativity.] Here we want to stick to mechanics and push the derivation of the coordinate transformation as far as possible without the use of the highly counterintuitive Einstein’s Second Postulate. The method that achieves this will be presented below and was originally due to Vladimir Ignatowsky [2]. [DISCLAIMER: I never read Ignatowsky’s original papers, but the idea is well-known within the community, often mentioned and discussed. Anyone with time to burn can reproduce the steps without much difficulty. The derivation below consists of 14 steps. If you can reduce that number, let me know.] Step 1: Galileo’s Law of Inertia for freely moving particles . . . implies the linearity of the coordinate transforma￾tion between K and K0 (see Fig. 2), x 0 = α11(v)x + α12(v)y + α13(v)z + α14(v)t, (1) y 0 = α21(v)x + α22(v)y + α23(v)z + α24(v)t, (2) z 0 = α31(v)x + α32(v)y + α33(v)z + α34(v)t, (3) t 0 = α41(v)x + α42(v)y + α43(v)z + α44(v)t. (4) Here we assumed that the origins of the two coordinate systems coincide, that is event (0, 0, 0, 0) in K has coor￾dinates (0, 0, 0, 0) in K0 . K K’ (x ’ , y ’ , z ’ , t’) P (x, y, z, t) O O’ v x ’ y ’ z ’ y x z FIG. 2: (Color online.) Two inertial reference frames (or￾thogonal grids of rods equipped with synchronized clocks) in relative motion along the x-axis