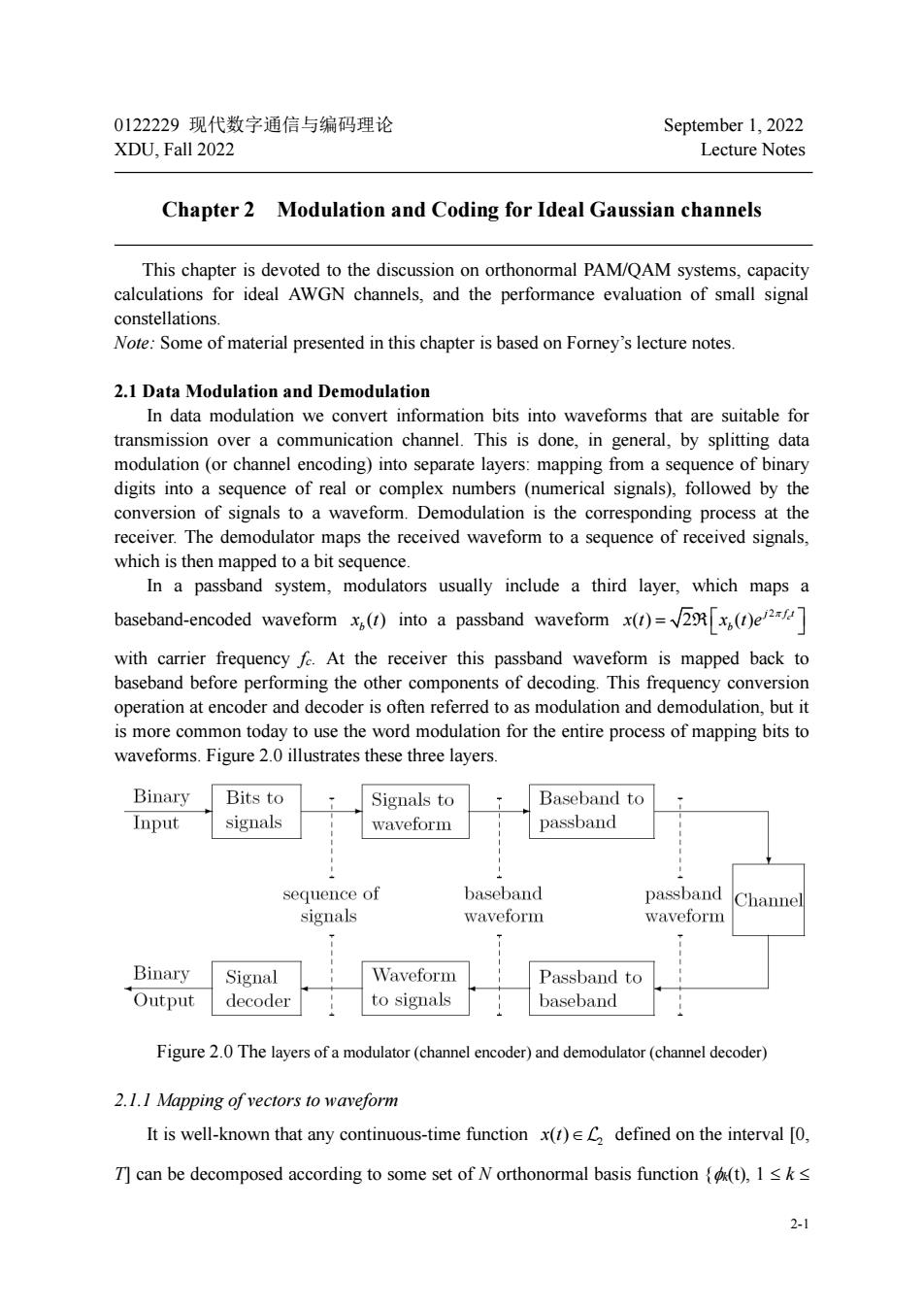
0122229现代数字通信与编码理论 September 1,2022 XDU,Fall 2022 Lecture Notes Chapter 2 Modulation and Coding for Ideal Gaussian channels This chapter is devoted to the discussion on orthonormal PAM/QAM systems,capacity calculations for ideal AWGN channels,and the performance evaluation of small signa constellations Note:Some of material presented in this chapter is based on Forney's lecture notes. 2.1 Data modulation and Demodulation In data modulation we c onvert information bits into waveforms that are suitable for transmission over a communication channel. This is done,in general,by splitting data modulation(or channel encoding)into separate layers:mapping from a sequence of binary digits into a sequence of real or complex numbers (numerical signals),followed by the conversion of signals to a waveform.Demodulation is the corresponding process at the ece maps the received waveform to a sequence of received signals In a passband system,modulators usually include a third layer,which maps a baseband-encoded waveform()into a passband wavefor(( with carrier frequencyf.At the receiver this passband waveform is mapped back to aseband ning the other components of decoding.This frequency conversion operation at encoder and decoder is often referred to as modulation and demodulation,but it is more common today to use the word modulation for the entire process of mapping bits to waveforms.Figure 2.0 illustrates these three layers. Binary Bits to Signals to Baseband to Input signals waveform passband of baseband pas an Channel waveform Binary Signal Waveform Passband to Output decoder to signals baseband Figure 2.0 The layers of a modulator (channel encoder)and demodulator (channel decoder) 2.1.1 Mapping of vectors to waveform It is well-known that any continuous-time function x()C defined on the interval [0. T]can be decomposed according to some set of N orthonormal basis function (t),Isks 2-12-1 0122229 现代数字通信与编码理论 September 1, 2022 XDU, Fall 2022 Lecture Notes Chapter 2 Modulation and Coding for Ideal Gaussian channels This chapter is devoted to the discussion on orthonormal PAM/QAM systems, capacity calculations for ideal AWGN channels, and the performance evaluation of small signal constellations. Note: Some of material presented in this chapter is based on Forney’s lecture notes. 2.1 Data Modulation and Demodulation In data modulation we convert information bits into waveforms that are suitable for transmission over a communication channel. This is done, in general, by splitting data modulation (or channel encoding) into separate layers: mapping from a sequence of binary digits into a sequence of real or complex numbers (numerical signals), followed by the conversion of signals to a waveform. Demodulation is the corresponding process at the receiver. The demodulator maps the received waveform to a sequence of received signals, which is then mapped to a bit sequence. In a passband system, modulators usually include a third layer, which maps a baseband-encoded waveform () b x t into a passband waveform 2 ( ) 2 ( ) c j f t b x t x t e  =   R   with carrier frequency fc. At the receiver this passband waveform is mapped back to baseband before performing the other components of decoding. This frequency conversion operation at encoder and decoder is often referred to as modulation and demodulation, but it is more common today to use the word modulation for the entire process of mapping bits to waveforms. Figure 2.0 illustrates these three layers. Figure 2.0 The layers of a modulator (channel encoder) and demodulator (channel decoder) 2.1.1 Mapping of vectors to waveform It is well-known that any continuous-time function 2 x t( ) defined on the interval [0, T] can be decomposed according to some set of N orthonormal basis function {k(t), 1  k 