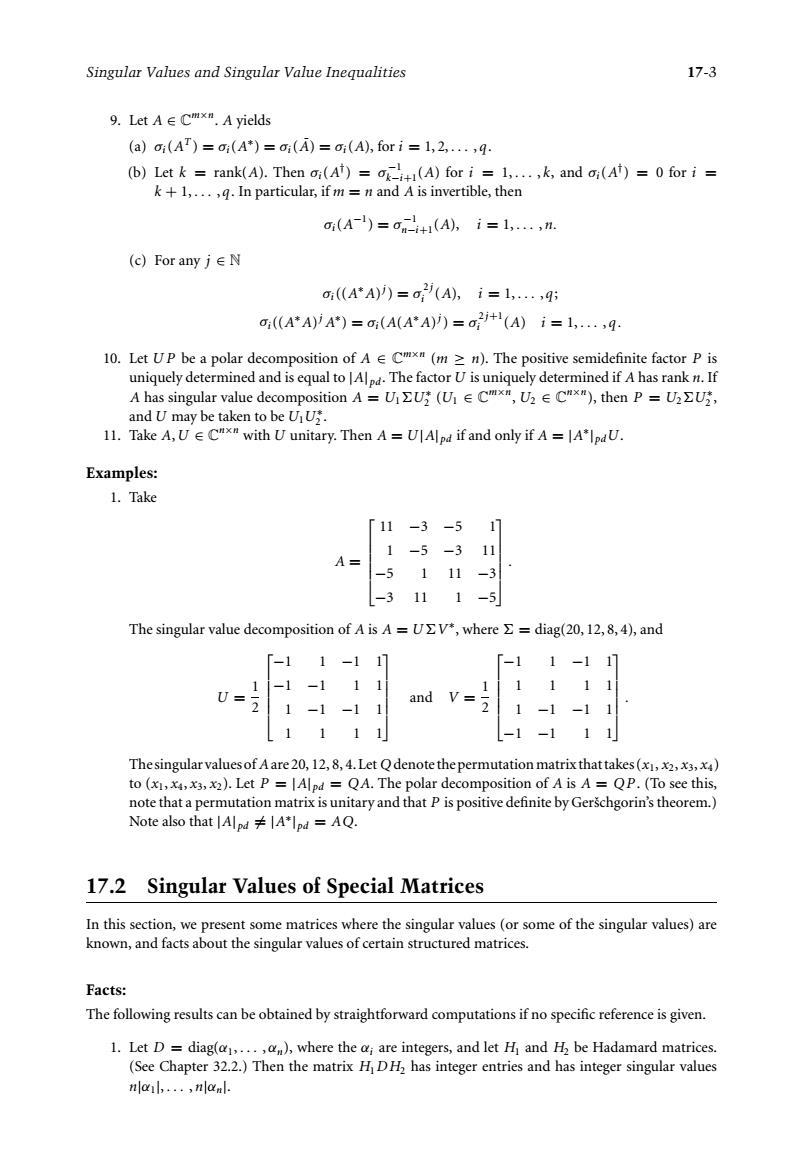
Singular Values and Singular Value Inequalities 17-3 9.LctA∈Cmx".A yields (a)a(AT)=(A')=0(A=a(A),fori=1,2,,9. 0(A)=0+(A),i=1,...,n (c)For any jeN G:(A°A))=a2(A,i=1,,q: G,(A*AyA)=(A(A*A)=+'(A）i=1,q: 10.Let UP be a sition of A∈Cmx(G sitive semidefinite factor Pi value s equal to )The pos ned if a ha ingu ion U:)P 11.Take A,with Uu nitary.Then A=UlAlp if and only if A =A'lpU. Examples: 1.Take 11-3-5 1-5-311 -5111-3 [-3111-5 The singular value decomposition of A is A=UV,where=diag(20,12,8,4),and 1-1 1111 -1 Thesingular valuesof Aare 20,12,8,4.Let Qdenote thepermutation matrix that takes() to(x,2).Let P=Alpd =QA.The polar decomposition of A is A=QP.(To see this note that a permutation matrix is unitary and that P is positive definite by Gerschgorin's theorem.) Note also that IAldA*lpd =AQ. 17.2 Singular Values of Special Matrices In this section,we resent some matrices where the singular values(or some of the singular values)are known,and facts about the singular values of certain structured matrices. Facts: The following results can be obtained by straightforward computations if no specific reference is given. 1.Let D=diag(),where the are integers,and let H and H be Hadamard matrices. (See Chapter 32.2.)Then the matrix HDH has integer entries and has integer singular values lal,…,nlca Singular Values and Singular Value Inequalities 17-3 9. Let A ∈ Cm×n. A yields (a) σi(AT ) = σi(A∗) = σi(A¯) = σi(A), for i = 1, 2, ... , q. (b) Let k = rank(A). Then σi(A†) = σ −1 k−i+1(A) for i = 1, ... , k, and σi(A†) = 0 for i = k + 1, ... , q. In particular, if m = n and A is invertible, then σi(A−1 ) = σ −1 n−i+1(A), i = 1, ... , n. (c) For any j ∈ N σi((A∗A)j ) = σ2 j i (A), i = 1, ... , q; σi((A∗A)j A∗) = σi(A(A∗A)j ) = σ2 j+1 i (A) i = 1, ... , q. 10. Let U P be a polar decomposition of A ∈ Cm×n (m ≥ n). The positive semidefinite factor P is uniquely determined and is equal to |A|pd . The factor U is uniquely determined if A has rank n. If A has singular value decomposition A = U1U∗ 2 (U1 ∈ Cm×n, U2 ∈ Cn×n), then P = U2U∗ 2 , and U may be taken to be U1U∗ 2 . 11. Take A,U ∈ Cn×n with U unitary. Then A = U|A|pd if and only if A = |A∗|pdU. Examples: 1. Take A = ⎡ ⎢ ⎢ ⎢ ⎢ ⎣ 11 −3 −5 1 1 −5 −3 11 −5 1 11 −3 −3 11 1 −5 ⎤ ⎥ ⎥ ⎥ ⎥ ⎦ . The singular value decomposition of A is A = UV∗, where = diag(20, 12, 8, 4), and U = 1 2 ⎡ ⎢ ⎢ ⎢ ⎢ ⎣ −1 1 −1 1 −1 −1 11 1 −1 −1 1 1 1 11 ⎤ ⎥ ⎥ ⎥ ⎥ ⎦ and V = 1 2 ⎡ ⎢ ⎢ ⎢ ⎢ ⎣ −1 1 −1 1 1 1 11 1 −1 −1 1 −1 −1 11 ⎤ ⎥ ⎥ ⎥ ⎥ ⎦ . The singular values of Aare 20, 12, 8, 4. Let Q denote the permutation matrix that takes (x1, x2, x3, x4) to (x1, x4, x3, x2). Let P = |A|pd = Q A. The polar decomposition of A is A = Q P . (To see this, note that a permutation matrix is unitary and that P is positive definite by Gerschgorin’s theorem.) ˇ Note also that |A|pd = |A∗|pd = AQ. 17.2 Singular Values of Special Matrices In this section, we present some matrices where the singular values (or some of the singular values) are known, and facts about the singular values of certain structured matrices. Facts: The following results can be obtained by straightforward computations if no specific reference is given. 1. Let D = diag(α1, ... , αn), where the αi are integers, and let H1 and H2 be Hadamard matrices. (See Chapter 32.2.) Then the matrix H1D H2 has integer entries and has integer singular values n|α1|, ... , n|αn|.