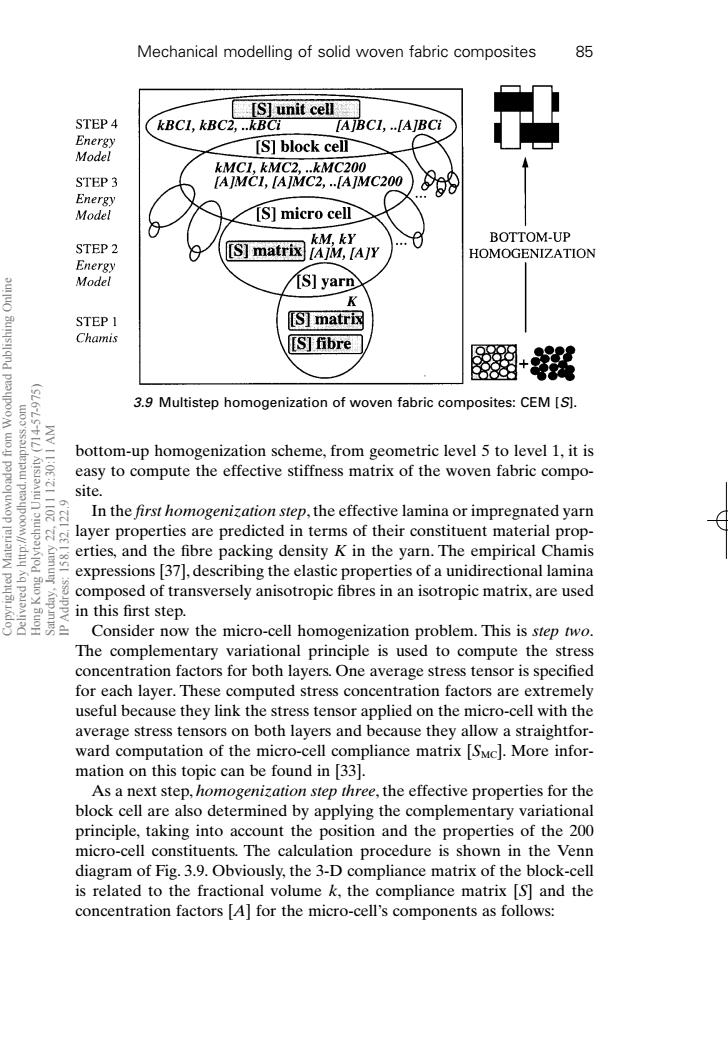
Mechanical modelling of solid woven fabric composites 85 [S]unit cell STEP4 kBCI,kBC2,..kBCi TAJBCI,..[AJBCi Energy [S]block cell Model kMCI,kMC2,..kMC200 STEP3 [AJMCI,[AJMC2,..[AJMC200 9g8 Energy Model [S]micro cell kM,kY BOTTOM-UP STEP 2 9 S]matri☒AM,[aJY HOMOGENIZATION Energy Model [S]yarn STEP 1 [S]matrix Chamis [S]fibre 3.9 Multistep homogenization of woven fabric composites:CEM [S]. WV IL:OE bottom-up homogenization scheme,from geometric level 5 to level 1,it is easy to compute the effective stiffness matrix of the woven fabric compo- 210 site. In the first homogenization step,the effective lamina or impregnated yarn layer properties are predicted in terms of their constituent material prop- erties,and the fibre packing density K in the yarn.The empirical Chamis expressions [37],describing the elastic properties of a unidirectional lamina composed of transversely anisotropic fibres in an isotropic matrix,are used in this first step. Consider now the micro-cell homogenization problem.This is step two. The complementary variational principle is used to compute the stress concentration factors for both layers.One average stress tensor is specified for each layer.These computed stress concentration factors are extremely useful because they link the stress tensor applied on the micro-cell with the average stress tensors on both layers and because they allow a straightfor- ward computation of the micro-cell compliance matrix [SMc].More infor- mation on this topic can be found in [33]. As a next step,homogenization step three,the effective properties for the block cell are also determined by applying the complementary variational principle,taking into account the position and the properties of the 200 micro-cell constituents.The calculation procedure is shown in the Venn diagram of Fig.3.9.Obviously,the 3-D compliance matrix of the block-cell is related to the fractional volume k,the compliance matrix [S]and the concentration factors [A]for the micro-cell's components as follows:bottom-up homogenization scheme, from geometric level 5 to level 1, it is easy to compute the effective stiffness matrix of the woven fabric compo￾site. In the first homogenization step, the effective lamina or impregnated yarn layer properties are predicted in terms of their constituent material prop￾erties, and the fibre packing density K in the yarn. The empirical Chamis expressions [37], describing the elastic properties of a unidirectional lamina composed of transversely anisotropic fibres in an isotropic matrix, are used in this first step. Consider now the micro-cell homogenization problem. This is step two. The complementary variational principle is used to compute the stress concentration factors for both layers. One average stress tensor is specified for each layer. These computed stress concentration factors are extremely useful because they link the stress tensor applied on the micro-cell with the average stress tensors on both layers and because they allow a straightfor￾ward computation of the micro-cell compliance matrix [SMC]. More infor￾mation on this topic can be found in [33]. As a next step, homogenization step three, the effective properties for the block cell are also determined by applying the complementary variational principle, taking into account the position and the properties of the 200 micro-cell constituents. The calculation procedure is shown in the Venn diagram of Fig. 3.9. Obviously, the 3-D compliance matrix of the block-cell is related to the fractional volume k, the compliance matrix [S] and the concentration factors [A] for the micro-cell’s components as follows: Mechanical modelling of solid woven fabric composites 85 3.9 Multistep homogenization of woven fabric composites: CEM [S]. RIC3 7/10/99 7:37 PM Page 85 Copyrighted Material downloaded from Woodhead Publishing Online Delivered by http://woodhead.metapress.com Hong Kong Polytechnic University (714-57-975) Saturday, January 22, 2011 12:30:11 AM IP Address: 158.132.122.9