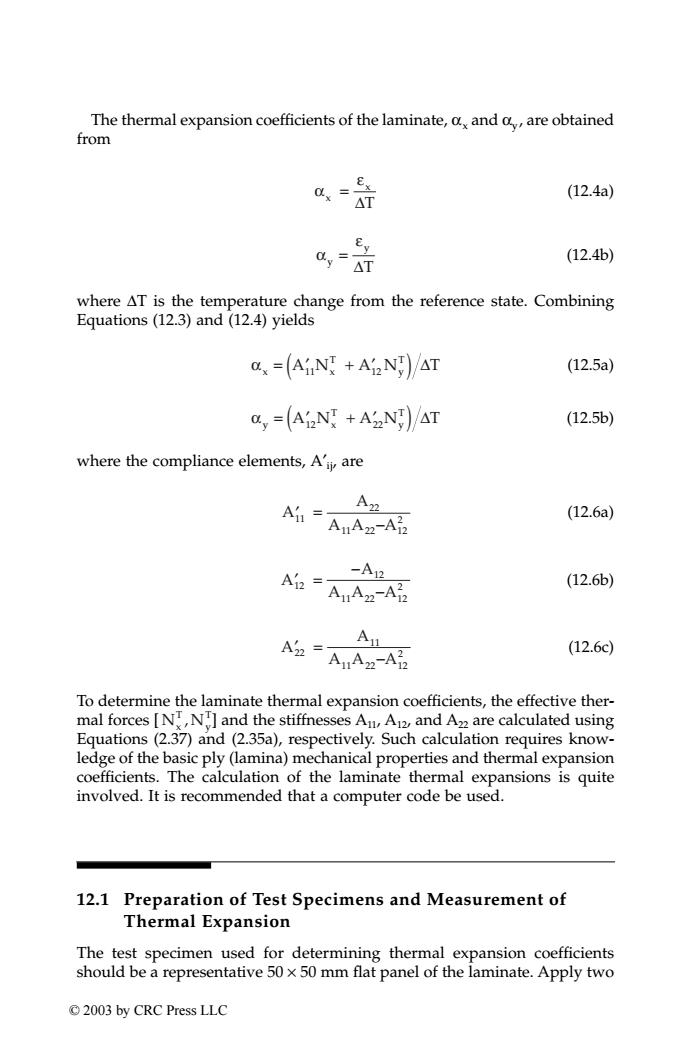
The thermal expansion coefficients of the laminate,and d,are obtained from 0x= Ex (12.4a) △T dy=AT (12.4b) where AT is the temperature change from the reference state.Combining Equations (12.3)and (12.4)yields =(AiN:+AiNy)/AT (12.5a) y=(AiNT +ANT)/AT (12.5b) where the compliance elements,Air are Ai =AuA2-A品 (12.6a) -A12 An =AuAz-Ais (12.6b) A A2-AuA-Ai (12.6c) To determine the laminate thermal expansion coefficients,the effective ther- mal forces [NT,NT]and the stiffnesses An,A,and Az are calculated using Equations (2.37)and (2.35a),respectively.Such calculation requires know- ledge of the basic ply (lamina)mechanical properties and thermal expansion coefficients.The calculation of the laminate thermal expansions is quite involved.It is recommended that a computer code be used. 12.1 Preparation of Test Specimens and Measurement of Thermal Expansion The test specimen used for determining thermal expansion coefficients should be a representative 50 x 50 mm flat panel of the laminate.Apply two ©2003 by CRC Press LLCThe thermal expansion coefficients of the laminate, αx and αy, are obtained from (12.4a) (12.4b) where ∆T is the temperature change from the reference state. Combining Equations (12.3) and (12.4) yields (12.5a) (12.5b) where the compliance elements, A′ij, are (12.6a) (12.6b) (12.6c) To determine the laminate thermal expansion coefficients, the effective ther￾mal forces [ ] and the stiffnesses A11, A12, and A22 are calculated using Equations (2.37) and (2.35a), respectively. Such calculation requires know￾ledge of the basic ply (lamina) mechanical properties and thermal expansion coefficients. The calculation of the laminate thermal expansions is quite involved. It is recommended that a computer code be used. 12.1 Preparation of Test Specimens and Measurement of Thermal Expansion The test specimen used for determining thermal expansion coefficients should be a representative 50 × 50 mm flat panel of the laminate. Apply two α ε x x T = ∆ α ε y y T = ∆ αx x T y T = ( ) AN A N T ′ + ′ 11 12 ∆ αy x T y T = ( ) AN AN T ′ + ′ 12 22 ∆ ′ = − A A AA A 11 22 11 22 12 2 ′ = − − A A AA A 12 12 11 22 12 2 ′ = − A A AA A 22 11 11 22 12 2 N Nx T y T , TX001_ch12_Frame Page 164 Saturday, September 21, 2002 5:05 AM © 2003 by CRC Press LLC