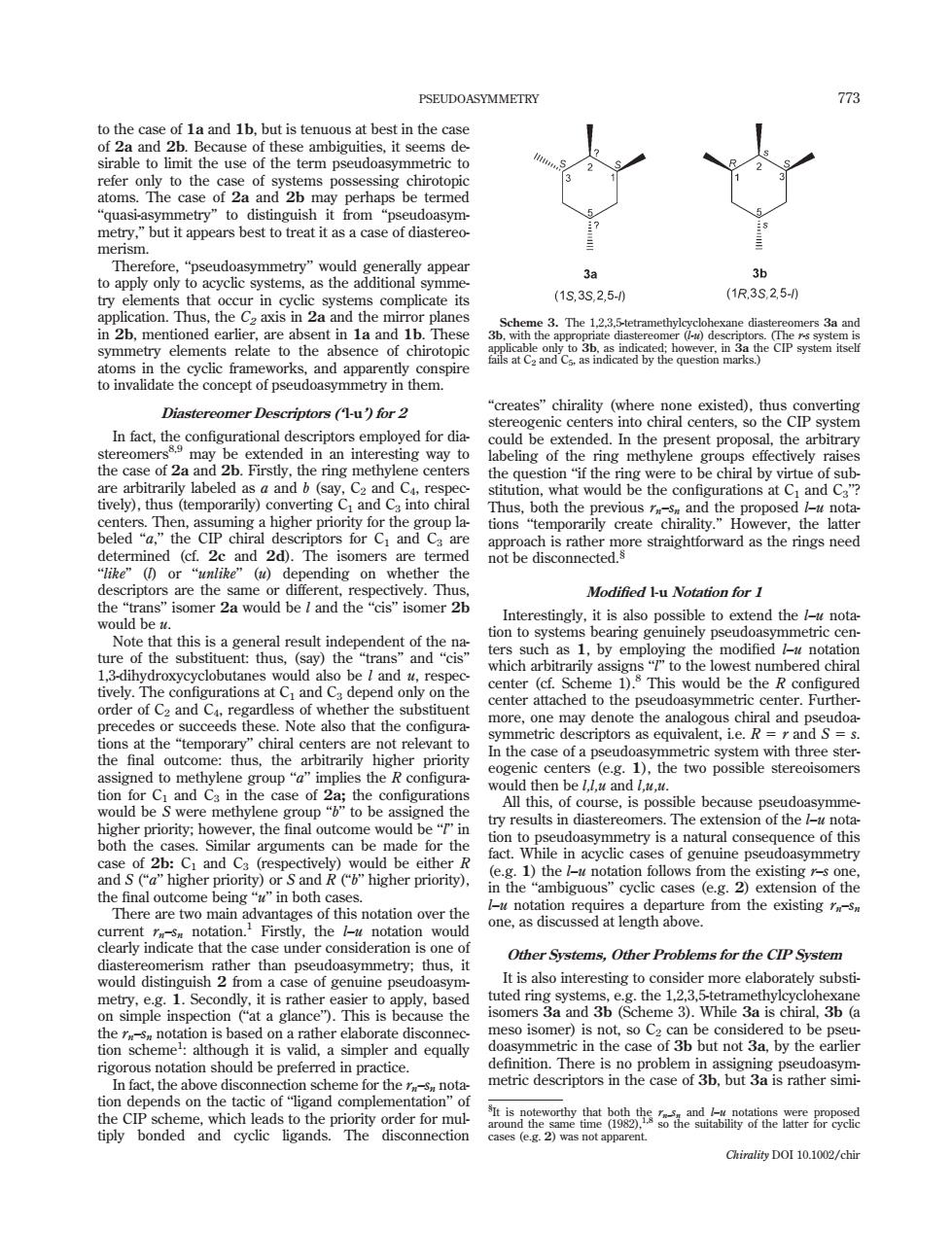
PSEUDOASYMMETRY 77 of 2a to distinguish from"p to 15,3S.25-0 (1R3S,250 in 2b.mentioned earlier.are a in la and 1b.Thes Sch dia rs 3a an nce and a mer Descriptors(Hu for 2 In fact,theco present pro the arbit the a stion 'if the re to be chiral by vi rtue of sul into chiral e the con tions ity." The isomers are ter not be disconnected ghtforward asthe ectively.Thu Modifed Lu Natation for I be and the 2 stingly,it is also ossible to e d the not tion to systems ring Note that this is to the lo est numbered chira uld center( This would b e s of wh s ch lso that the more, oe may denote the al and pses he final outco e:thus with three ster the arily higher priority In the case of a p cud as e the ohen be e ps arguments can be metry is a naturalco e in acyc n the nbiguous”cyc beng“nbot of this r one.as discussed at length above. 9 ation Other Systems,Other Problems for the CIP System dia rathe thar thus of genuine ps ide elabora y subst t is tuted rin sys mle inspection at a glan th is n sidered to be ion scheme:although it is valid,a simpler and equally but not 3a by the earlie metric descriptors in the case of 3b.but a israther simi tion depends on the ind complen tiply bonded and nnection Chirality DOI 10.1002/chir to the case of 1a and 1b, but is tenuous at best in the case of 2a and 2b. Because of these ambiguities, it seems de￾sirable to limit the use of the term pseudoasymmetric to refer only to the case of systems possessing chirotopic atoms. The case of 2a and 2b may perhaps be termed ‘‘quasi-asymmetry’’ to distinguish it from ‘‘pseudoasym￾metry,’’ but it appears best to treat it as a case of diastereo￾merism. Therefore, ‘‘pseudoasymmetry’’ would generally appear to apply only to acyclic systems, as the additional symme￾try elements that occur in cyclic systems complicate its application. Thus, the C2 axis in 2a and the mirror planes in 2b, mentioned earlier, are absent in 1a and 1b. These symmetry elements relate to the absence of chirotopic atoms in the cyclic frameworks, and apparently conspire to invalidate the concept of pseudoasymmetry in them. Diastereomer Descriptors (‘ l-u’) for 2 In fact, the configurational descriptors employed for dia￾stereomers8,9 may be extended in an interesting way to the case of 2a and 2b. Firstly, the ring methylene centers are arbitrarily labeled as a and b (say, C2 and C4, respec￾tively), thus (temporarily) converting C1 and C3 into chiral centers. Then, assuming a higher priority for the group la￾beled ‘‘a,’’ the CIP chiral descriptors for C1 and C3 are determined (cf. 2c and 2d). The isomers are termed ‘‘like’’ (l) or ‘‘unlike’’ (u) depending on whether the descriptors are the same or different, respectively. Thus, the ‘‘trans’’ isomer 2a would be l and the ‘‘cis’’ isomer 2b would be u. Note that this is a general result independent of the na￾ture of the substituent: thus, (say) the ‘‘trans’’ and ‘‘cis’’ 1,3-dihydroxycyclobutanes would also be l and u, respec￾tively. The configurations at C1 and C3 depend only on the order of C2 and C4, regardless of whether the substituent precedes or succeeds these. Note also that the configura￾tions at the ‘‘temporary’’ chiral centers are not relevant to the final outcome: thus, the arbitrarily higher priority assigned to methylene group ‘‘a’’ implies the R configura￾tion for C1 and C3 in the case of 2a; the configurations would be S were methylene group ‘‘b’’ to be assigned the higher priority; however, the final outcome would be ‘‘l’’ in both the cases. Similar arguments can be made for the case of 2b: C1 and C3 (respectively) would be either R and S (‘‘a’’ higher priority) or S and R (‘‘b’’ higher priority), the final outcome being ‘‘u’’ in both cases. There are two main advantages of this notation over the current rn–sn notation.1 Firstly, the l–u notation would clearly indicate that the case under consideration is one of diastereomerism rather than pseudoasymmetry; thus, it would distinguish 2 from a case of genuine pseudoasym￾metry, e.g. 1. Secondly, it is rather easier to apply, based on simple inspection (‘‘at a glance’’). This is because the the rn–sn notation is based on a rather elaborate disconnec￾tion scheme1 : although it is valid, a simpler and equally rigorous notation should be preferred in practice. In fact, the above disconnection scheme for the rn–sn nota￾tion depends on the tactic of ‘‘ligand complementation’’ of the CIP scheme, which leads to the priority order for mul￾tiply bonded and cyclic ligands. The disconnection ‘‘creates’’ chirality (where none existed), thus converting stereogenic centers into chiral centers, so the CIP system could be extended. In the present proposal, the arbitrary labeling of the ring methylene groups effectively raises the question ‘‘if the ring were to be chiral by virtue of sub￾stitution, what would be the configurations at C1 and C3’’? Thus, both the previous rn–sn and the proposed l–u nota￾tions ‘‘temporarily create chirality.’’ However, the latter approach is rather more straightforward as the rings need not be disconnected.§ Modified l-u Notation for 1 Interestingly, it is also possible to extend the l–u nota￾tion to systems bearing genuinely pseudoasymmetric cen￾ters such as 1, by employing the modified l–u notation which arbitrarily assigns ‘‘l’’ to the lowest numbered chiral center (cf. Scheme 1).8 This would be the R configured center attached to the pseudoasymmetric center. Further￾more, one may denote the analogous chiral and pseudoa￾symmetric descriptors as equivalent, i.e. R 5 r and S 5 s. In the case of a pseudoasymmetric system with three ster￾eogenic centers (e.g. 1), the two possible stereoisomers would then be l,l,u and l,u,u. All this, of course, is possible because pseudoasymme￾try results in diastereomers. The extension of the l–u nota￾tion to pseudoasymmetry is a natural consequence of this fact. While in acyclic cases of genuine pseudoasymmetry (e.g. 1) the l–u notation follows from the existing r–s one, in the ‘‘ambiguous’’ cyclic cases (e.g. 2) extension of the l–u notation requires a departure from the existing rn–sn one, as discussed at length above. Other Systems, Other Problems for the CIP System It is also interesting to consider more elaborately substi￾tuted ring systems, e.g. the 1,2,3,5-tetramethylcyclohexane isomers 3a and 3b (Scheme 3). While 3a is chiral, 3b (a meso isomer) is not, so C2 can be considered to be pseu￾doasymmetric in the case of 3b but not 3a, by the earlier definition. There is no problem in assigning pseudoasym￾metric descriptors in the case of 3b, but 3a is rather simi￾Scheme 3. The 1,2,3,5-tetramethylcyclohexane diastereomers 3a and 3b, with the appropriate diastereomer (l-u) descriptors. (The r-s system is applicable only to 3b, as indicated; however, in 3a the CIP system itself fails at C2 and C5, as indicated by the question marks.) § It is noteworthy that both the rn–sn and l–u notations were proposed around the same time (1982),1,8 so the suitability of the latter for cyclic cases (e.g. 2) was not apparent. PSEUDOASYMMETRY 773 Chirality DOI 10.1002/chir