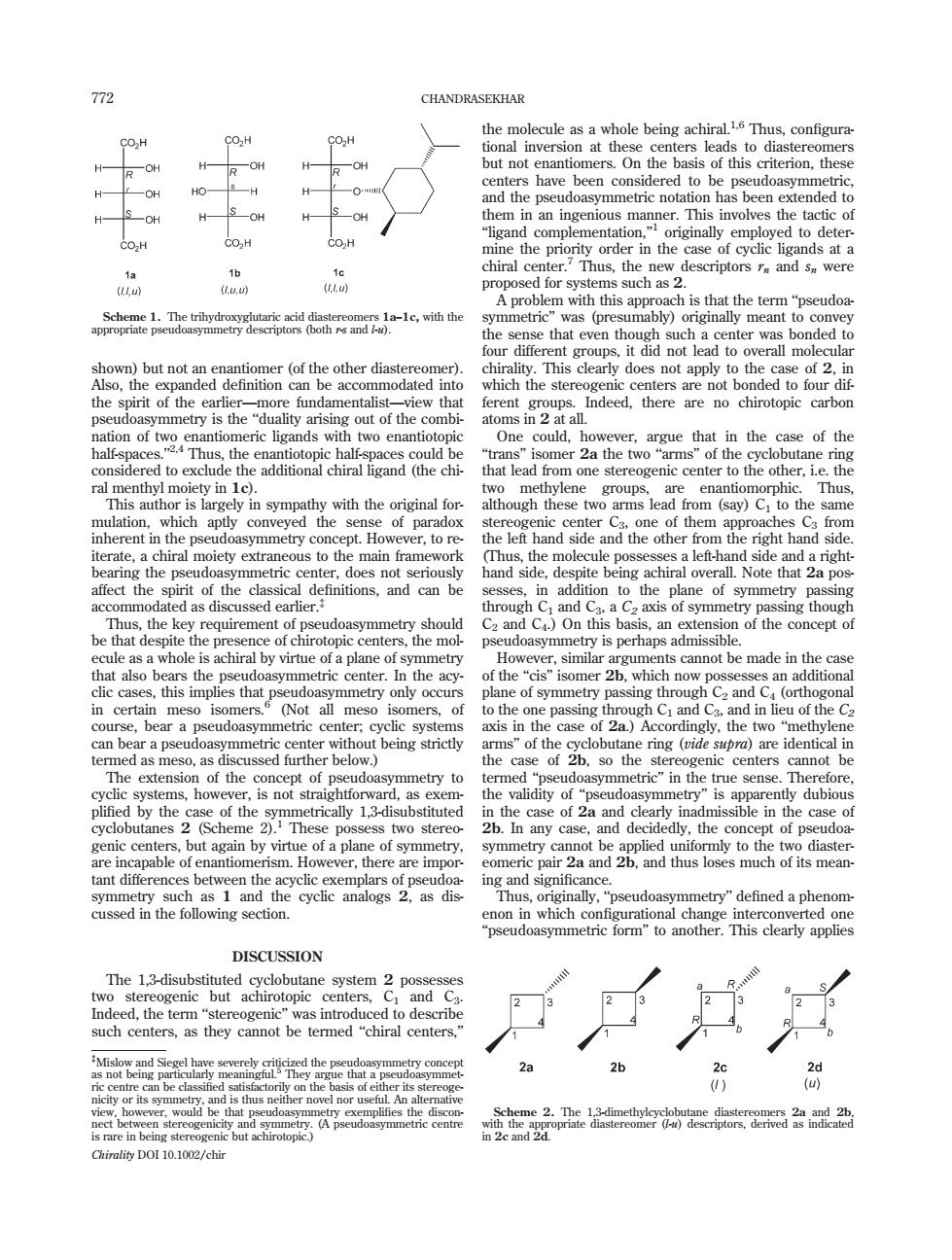
772 CHANDRASEKHAR the molecule as a whole being achiral..Thus,configura R一O s have H nd be pseudoasymmetri ner.This involves the tactic o co, chiral c rThus,the new descriptors r.and s.were ul8 ach is that the term"pseudoa stemeTchtaamramlr-1e,hhc meant to conve our diffe did not lea verall mol be accommodated into which the stereog ntersare not bonded to fou ion of ligands two enanti ne could, argue that in the case of the which atly conveyed the of paradox to the main fram (Thus,the and a right I definitions.and can be a po through C and C a Caxis of ymmetry passing though centers.the mo doasymmetry is perhaps admissible of the of on ter cyclic syste case e two ly axis in the cas of 2b.so the ster he extensio doasy etry to n the t of the y the etrically13-disubstituted 2a and rly the cas nic centers.but agan by of a plane of sy lied unifo mly to the two diaster nt enantiom sm. ever, e im d 2p.and thus loses much o of its mean pseudoasymmetric form to another.This clearly applies DISCUSSION was centers 严时芦 4 be er its stereoge er no Chirality DOI 10.1002/chirshown) but not an enantiomer (of the other diastereomer). Also, the expanded definition can be accommodated into the spirit of the earlier—more fundamentalist—view that pseudoasymmetry is the ‘‘duality arising out of the combi￾nation of two enantiomeric ligands with two enantiotopic half-spaces.’’2,4 Thus, the enantiotopic half-spaces could be considered to exclude the additional chiral ligand (the chi￾ral menthyl moiety in 1c). This author is largely in sympathy with the original for￾mulation, which aptly conveyed the sense of paradox inherent in the pseudoasymmetry concept. However, to re￾iterate, a chiral moiety extraneous to the main framework bearing the pseudoasymmetric center, does not seriously affect the spirit of the classical definitions, and can be accommodated as discussed earlier.{ Thus, the key requirement of pseudoasymmetry should be that despite the presence of chirotopic centers, the mol￾ecule as a whole is achiral by virtue of a plane of symmetry that also bears the pseudoasymmetric center. In the acy￾clic cases, this implies that pseudoasymmetry only occurs in certain meso isomers.6 (Not all meso isomers, of course, bear a pseudoasymmetric center; cyclic systems can bear a pseudoasymmetric center without being strictly termed as meso, as discussed further below.) The extension of the concept of pseudoasymmetry to cyclic systems, however, is not straightforward, as exem￾plified by the case of the symmetrically 1,3-disubstituted cyclobutanes 2 (Scheme 2).1 These possess two stereo￾genic centers, but again by virtue of a plane of symmetry, are incapable of enantiomerism. However, there are impor￾tant differences between the acyclic exemplars of pseudoa￾symmetry such as 1 and the cyclic analogs 2, as dis￾cussed in the following section. DISCUSSION The 1,3-disubstituted cyclobutane system 2 possesses two stereogenic but achirotopic centers, C1 and C3. Indeed, the term ‘‘stereogenic’’ was introduced to describe such centers, as they cannot be termed ‘‘chiral centers,’’ the molecule as a whole being achiral.1,6 Thus, configura￾tional inversion at these centers leads to diastereomers but not enantiomers. On the basis of this criterion, these centers have been considered to be pseudoasymmetric, and the pseudoasymmetric notation has been extended to them in an ingenious manner. This involves the tactic of ‘‘ligand complementation,’’1 originally employed to deter￾mine the priority order in the case of cyclic ligands at a chiral center.7 Thus, the new descriptors rn and sn were proposed for systems such as 2. A problem with this approach is that the term ‘‘pseudoa￾symmetric’’ was (presumably) originally meant to convey the sense that even though such a center was bonded to four different groups, it did not lead to overall molecular chirality. This clearly does not apply to the case of 2, in which the stereogenic centers are not bonded to four dif￾ferent groups. Indeed, there are no chirotopic carbon atoms in 2 at all. One could, however, argue that in the case of the ‘‘trans’’ isomer 2a the two ‘‘arms’’ of the cyclobutane ring that lead from one stereogenic center to the other, i.e. the two methylene groups, are enantiomorphic. Thus, although these two arms lead from (say) C1 to the same stereogenic center C3, one of them approaches C3 from the left hand side and the other from the right hand side. (Thus, the molecule possesses a left-hand side and a right￾hand side, despite being achiral overall. Note that 2a pos￾sesses, in addition to the plane of symmetry passing through C1 and C3, a C2 axis of symmetry passing though C2 and C4.) On this basis, an extension of the concept of pseudoasymmetry is perhaps admissible. However, similar arguments cannot be made in the case of the ‘‘cis’’ isomer 2b, which now possesses an additional plane of symmetry passing through C2 and C4 (orthogonal to the one passing through C1 and C3, and in lieu of the C2 axis in the case of 2a.) Accordingly, the two ‘‘methylene arms’’ of the cyclobutane ring (vide supra) are identical in the case of 2b, so the stereogenic centers cannot be termed ‘‘pseudoasymmetric’’ in the true sense. Therefore, the validity of ‘‘pseudoasymmetry’’ is apparently dubious in the case of 2a and clearly inadmissible in the case of 2b. In any case, and decidedly, the concept of pseudoa￾symmetry cannot be applied uniformly to the two diaster￾eomeric pair 2a and 2b, and thus loses much of its mean￾ing and significance. Thus, originally, ‘‘pseudoasymmetry’’ defined a phenom￾enon in which configurational change interconverted one ‘‘pseudoasymmetric form’’ to another. This clearly applies Scheme 1. The trihydroxyglutaric acid diastereomers 1a–1c, with the appropriate pseudoasymmetry descriptors (both r-s and l-u). Scheme 2. The 1,3-dimethylcyclobutane diastereomers 2a and 2b, with the appropriate diastereomer (l-u) descriptors, derived as indicated in 2c and 2d. { Mislow and Siegel have severely criticized the pseudoasymmetry concept as not being particularly meaningful.5 They argue that a pseudoasymmet￾ric centre can be classified satisfactorily on the basis of either its stereoge￾nicity or its symmetry, and is thus neither novel nor useful. An alternative view, however, would be that pseudoasymmetry exemplifies the discon￾nect between stereogenicity and symmetry. (A pseudoasymmetric centre is rare in being stereogenic but achirotopic.) 772 CHANDRASEKHAR Chirality DOI 10.1002/chir