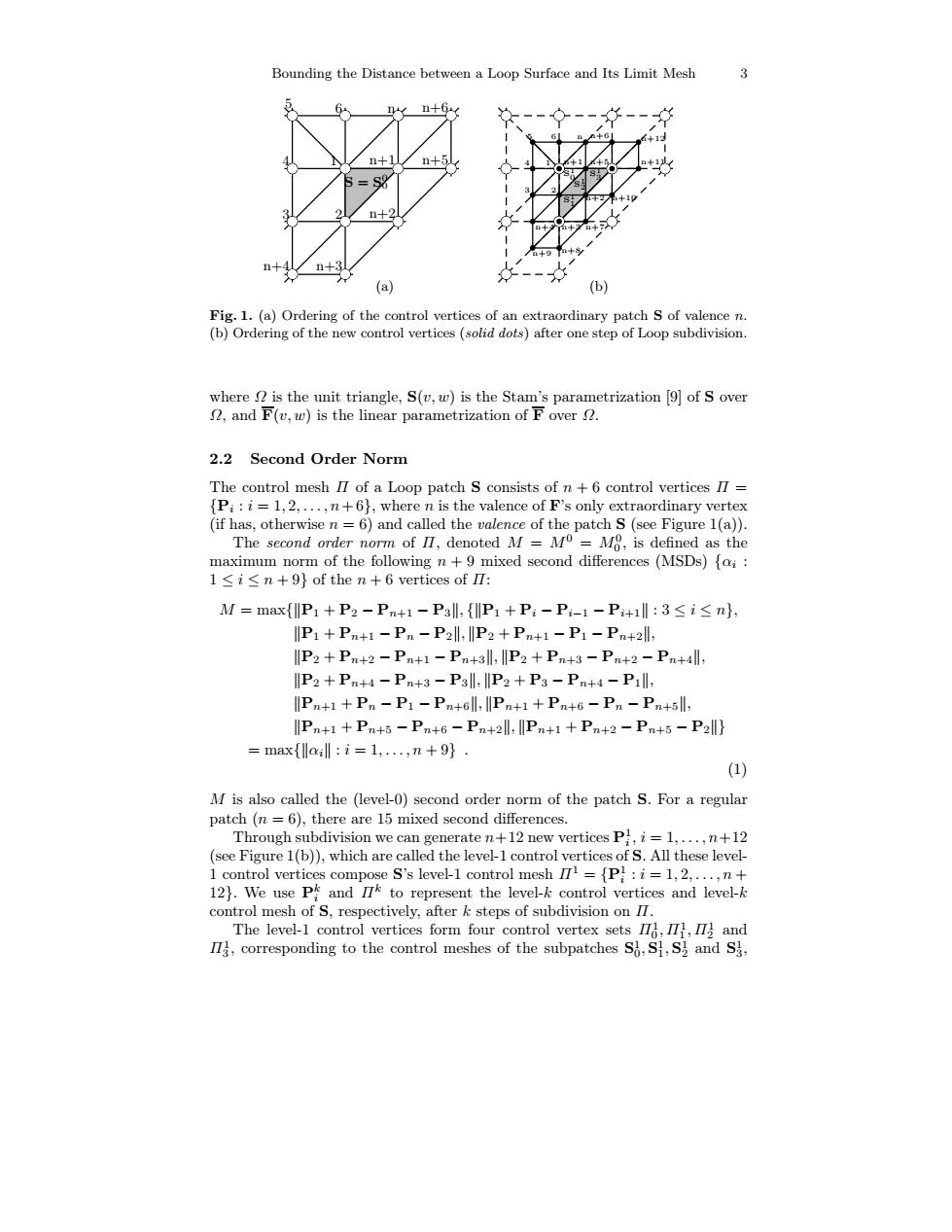
2.2 Sccond Order Norm D +Pa-P P.IP.+P2-P-P✉ e+12nmwmkaPBounding the Distance between a Loop Surface and Its Limit Mesh 3 bc bc bc bc bc bc bc bc bc bc bc bc bc 5 6 n n+6 4 1 n+1 n+5 3 2 n+2 n+4 n+3 S = S 0 0 (a) bc bc bc bc bc bc bc bc bc bc bc bc bc b b b b b b b b b b b b b b 5 6 n b b b b b n+6 n+12 4 1 n+1 n+5 n+11 3 2 n+2 n+10 n+4 n+3 n+7 n+9 n+8 S1 0 S1 1 S1 2 S1 3 (b) Fig. 1. (a) Ordering of the control vertices of an extraordinary patch S of valence n. (b) Ordering of the new control vertices (solid dots) after one step of Loop subdivision. where Ω is the unit triangle, S(v, w) is the Stam’s parametrization [9] of S over Ω, and F(v, w) is the linear parametrization of F over Ω. 2.2 Second Order Norm The control mesh Π of a Loop patch S consists of n + 6 control vertices Π = {Pi : i = 1, 2, . . . , n+ 6}, where n is the valence of F’s only extraordinary vertex (if has, otherwise n = 6) and called the valence of the patch S (see Figure 1(a)). The second order norm of Π, denoted M = M0 = M0 0 , is defined as the maximum norm of the following n + 9 mixed second differences (MSDs) {αi : 1 ≤ i ≤ n + 9} of the n + 6 vertices of Π: M = max{kP1 + P2 − Pn+1 − P3k, {kP1 + Pi − Pi−1 − Pi+1k : 3 ≤ i ≤ n}, kP1 + Pn+1 − Pn − P2k, kP2 + Pn+1 − P1 − Pn+2k, kP2 + Pn+2 − Pn+1 − Pn+3k, kP2 + Pn+3 − Pn+2 − Pn+4k, kP2 + Pn+4 − Pn+3 − P3k, kP2 + P3 − Pn+4 − P1k, kPn+1 + Pn − P1 − Pn+6k, kPn+1 + Pn+6 − Pn − Pn+5k, kPn+1 + Pn+5 − Pn+6 − Pn+2k, kPn+1 + Pn+2 − Pn+5 − P2k} = max{kαik : i = 1, . . . , n + 9} . (1) M is also called the (level-0) second order norm of the patch S. For a regular patch (n = 6), there are 15 mixed second differences. Through subdivision we can generate n+12 new vertices P1 i , i = 1, . . . , n+12 (see Figure 1(b)), which are called the level-1 control vertices of S. All these level- 1 control vertices compose S’s level-1 control mesh Π1 = {P1 i : i = 1, 2, . . . , n + 12}. We use Pk i and Πk to represent the level-k control vertices and level-k control mesh of S, respectively, after k steps of subdivision on Π. The level-1 control vertices form four control vertex sets Π1 0 , Π1 1 , Π1 2 and Π1 3 , corresponding to the control meshes of the subpatches S 1 0 , S 1 1 , S 1 2 and S 1 3