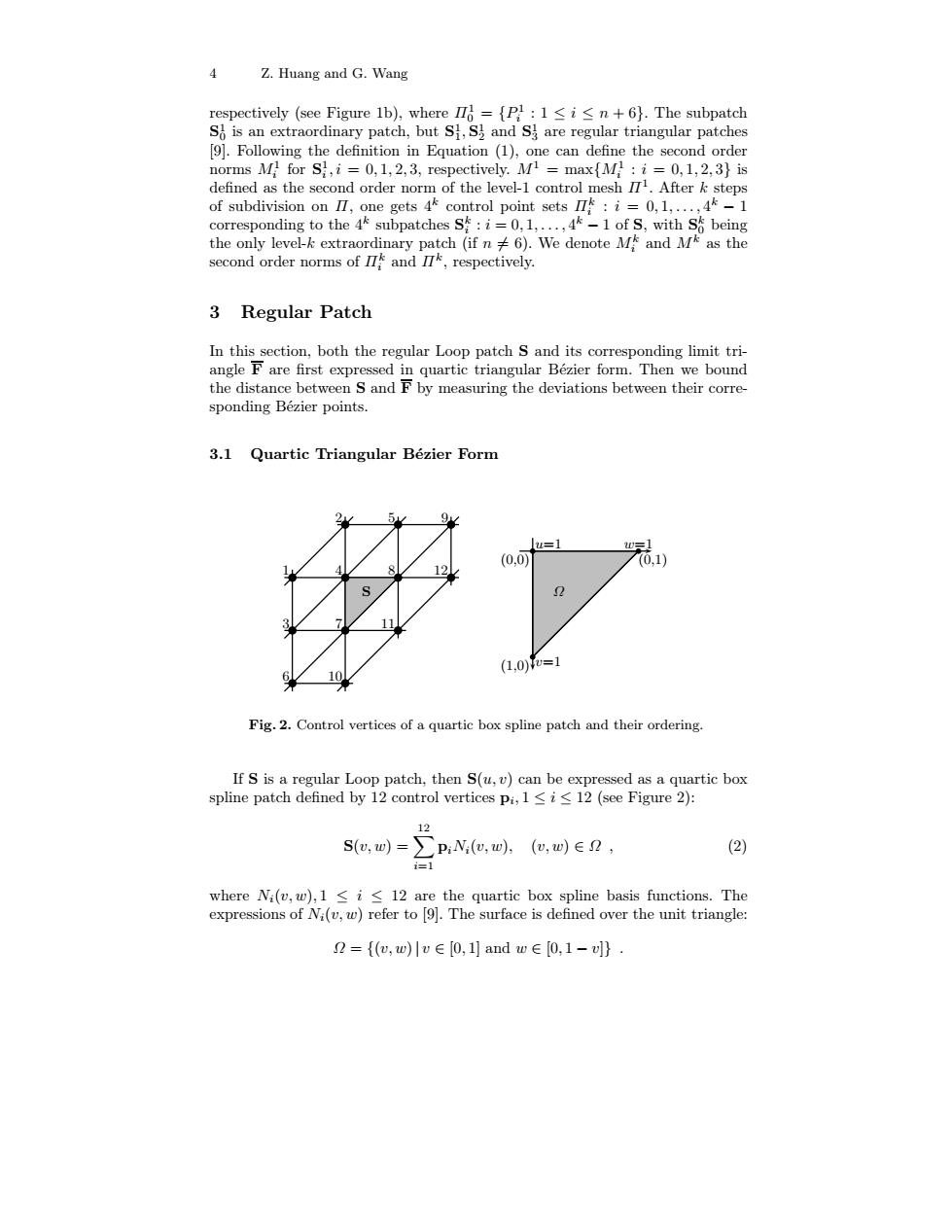
4 3 Regular Patch Quartie Triangular Form pSa物sR罗ata 2 我k ((.)v0]and 4 Z. Huang and G. Wang respectively (see Figure 1b), where Π1 0 = {P 1 i : 1 ≤ i ≤ n + 6}. The subpatch S 1 0 is an extraordinary patch, but S 1 1 , S 1 2 and S 1 3 are regular triangular patches [9]. Following the definition in Equation (1), one can define the second order norms M1 i for S 1 i , i = 0, 1, 2, 3, respectively. M1 = max{M1 i : i = 0, 1, 2, 3} is defined as the second order norm of the level-1 control mesh Π1 . After k steps of subdivision on Π, one gets 4k control point sets Πk i : i = 0, 1, . . . , 4 k − 1 corresponding to the 4k subpatches S k i : i = 0, 1, . . . , 4 k − 1 of S, with S k 0 being the only level-k extraordinary patch (if n 6= 6). We denote Mk i and Mk as the second order norms of Πk i and Πk , respectively. 3 Regular Patch In this section, both the regular Loop patch S and its corresponding limit tri￾angle F are first expressed in quartic triangular B´ezier form. Then we bound the distance between S and F by measuring the deviations between their corre￾sponding B´ezier points. 3.1 Quartic Triangular B´ezier Form b b b b b b b b b 2 5 9 b b b 1 4 8 12 3 7 11 6 10 S b b b Ω (0,0) u=1 (0,1) (1,0) w=1 v=1 Fig. 2. Control vertices of a quartic box spline patch and their ordering. If S is a regular Loop patch, then S(u, v) can be expressed as a quartic box spline patch defined by 12 control vertices pi , 1 ≤ i ≤ 12 (see Figure 2): S(v, w) = X 12 i=1 piNi(v, w), (v, w) ∈ Ω , (2) where Ni(v, w), 1 ≤ i ≤ 12 are the quartic box spline basis functions. The expressions of Ni(v, w) refer to [9]. The surface is defined over the unit triangle: Ω = {(v, w)| v ∈ [0, 1] and w ∈ [0, 1 − v]}