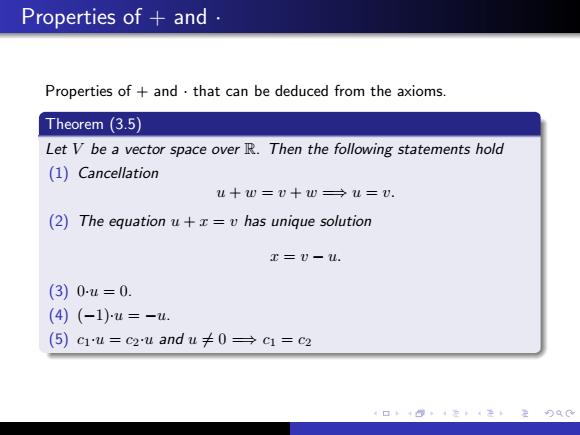
Properties of+and· Properties of+and.that can be deduced from the axioms. Theorem (3.5) Let V be a vector space over R.Then the following statements hold (1)Cancellation u十w=U+w→u=U. (2)The equation u+x=v has unique solution I=U-u. (3)0-u=0. (4)(-1)u=-u. (5)c1u=c2 u and u≠0→c1=c2 4口+心左4生主9QCProperties of + and · Properties of + and · that can be deduced from the axioms. Theorem (3.5) Let V be a vector space over R. Then the following statements hold (1) Cancellation u + w = v + w =⇒ u = v. (2) The equation u + x = v has unique solution x = v − u. (3) 0·u = 0. (4) (−1)·u = −u. (5) c1·u = c2·u and u 6= 0 =⇒ c1 = c2