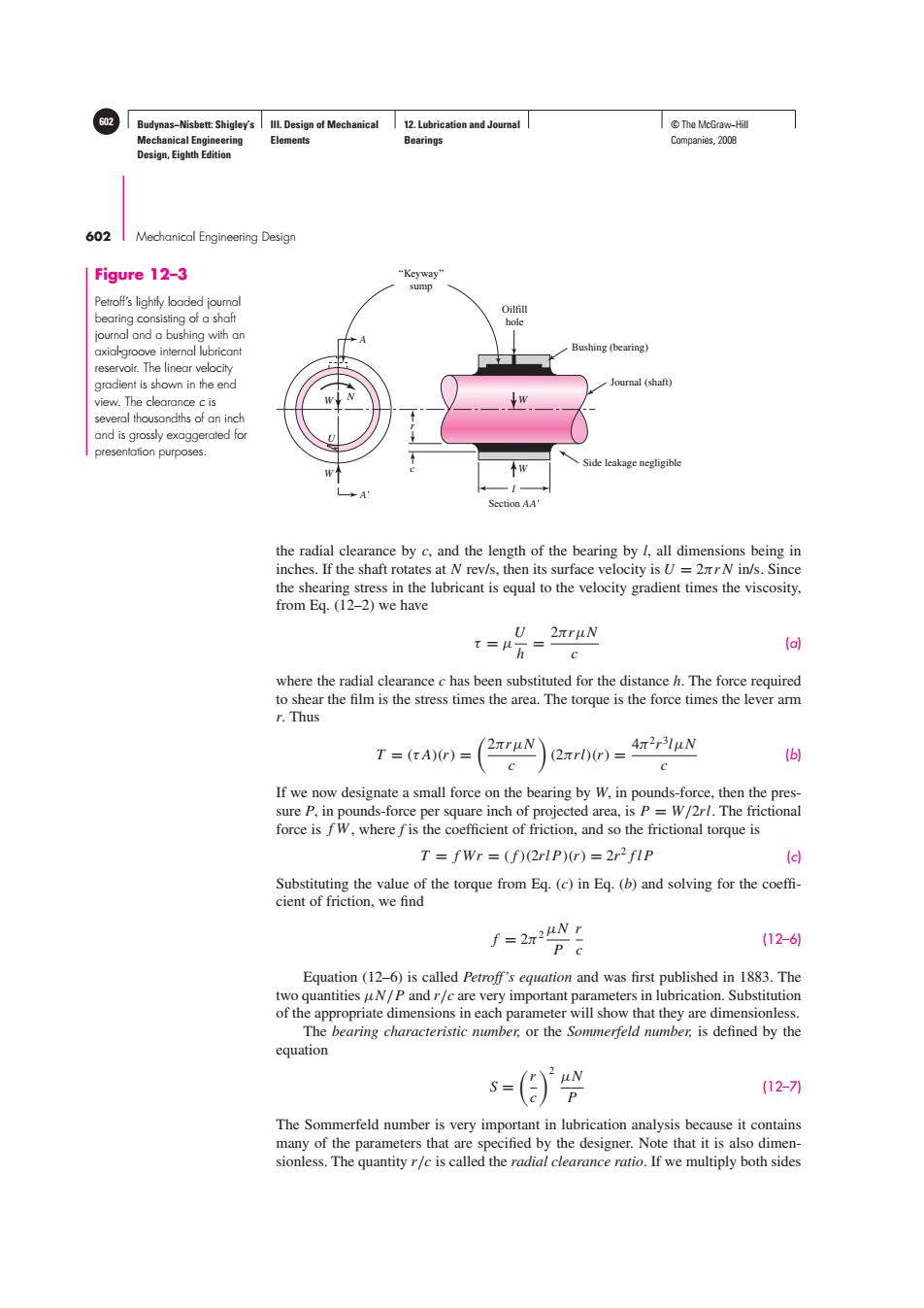
602 Budynas-Nisbett:Shigley's Ill.Design of Mechanical 12.Lubrication and Journal T©The McGraw-Hil Mechanical Engineering Elements Bearings Companies,2008 Design,Eighth Edition 602 Mechanical Engineering Design Figure 12-3 “Keyway” sump Petroff's lightly loaded journal Oilfill bearing consisting of a shaft bole journal and a bushing with an axial-groove internal lubricant Bushing(bearing） reservoir.The linear velocity gradient is shown in the end Journal (shaft) view.The clearance c is several thousandths of an inch and is grossly exaggerated for presentation purposes. w Side leakage negligible A Section AA' the radial clearance by c,and the length of the bearing by l,all dimensions being in inches.If the shaft rotates at N rev/s,then its surface velocity is U =2xrN in/s.Since the shearing stress in the lubricant is equal to the velocity gradient times the viscosity, from Eq.(12-2)we have U2πruN (a) where the radial clearance c has been substituted for the distance h.The force required to shear the film is the stress times the area.The torque is the force times the lever arm r.Thus T=(A)(r)= 2πruN 4π2r3luN (2πr)(r)= 6 c If we now designate a small force on the bearing by W,in pounds-force,then the pres- sure P,in pounds-force per square inch of projected area,is P=W/2rl.The frictional force is fW,where fis the coefficient of friction,and so the frictional torque is T=fWr=(f)(2rlP)(r)=2r2fIP (c Substituting the value of the torque from Eq.(c)in Eq.(b)and solving for the coeffi- cient of friction,we find f=272UN r P c (12-61 Equation (12-6)is called Petroff's equation and was first published in 1883.The two quantities uN/P and r/c are very important parameters in lubrication.Substitution of the appropriate dimensions in each parameter will show that they are dimensionless. The bearing characteristic number;or the Sommerfeld number,is defined by the equation s=)'w c P (12-7刀 The Sommerfeld number is very important in lubrication analysis because it contains many of the parameters that are specified by the designer.Note that it is also dimen- sionless.The quantity r/c is called the radial clearance ratio.If we multiply both sidesBudynas−Nisbett: Shigley’s Mechanical Engineering Design, Eighth Edition III. Design of Mechanical Elements 12. Lubrication and Journal Bearings 602 © The McGraw−Hill Companies, 2008 602 Mechanical Engineering Design Figure 12–3 Petroff’s lightly loaded journal bearing consisting of a shaft journal and a bushing with an axial-groove internal lubricant reservoir. The linear velocity gradient is shown in the end view. The clearance c is several thousandths of an inch and is grossly exaggerated for presentation purposes. A N A' W W r c W l Section AA' W U “Keyway” sump Oilfill hole Bushing (bearing) Journal (shaft) Side leakage negligible the radial clearance by c, and the length of the bearing by l, all dimensions being in inches. If the shaft rotates at N rev/s, then its surface velocity is U = 2πr N in/s. Since the shearing stress in the lubricant is equal to the velocity gradient times the viscosity, from Eq. (12–2) we have τ = μ U h = 2πrμN c (a) where the radial clearance c has been substituted for the distance h. The force required to shear the film is the stress times the area. The torque is the force times the lever arm r. Thus T = (τ A)(r) = 2πrμN c (2πrl)(r) = 4π2r 3lμN c (b) If we now designate a small force on the bearing by W, in pounds-force, then the pres￾sure P, in pounds-force per square inch of projected area, is P = W/2rl. The frictional force is f W , where f is the coefficient of friction, and so the frictional torque is T = f Wr = ( f )(2rlP)(r) = 2r 2 flP (c) Substituting the value of the torque from Eq. (c) in Eq. (b) and solving for the coeffi- cient of friction, we find f = 2π2μN P r c (12–6) Equation (12–6) is called Petroff’s equation and was first published in 1883. The two quantities μN/P and r/c are very important parameters in lubrication. Substitution of the appropriate dimensions in each parameter will show that they are dimensionless. The bearing characteristic number, or the Sommerfeld number, is defined by the equation S = r c 2 μN P (12–7) The Sommerfeld number is very important in lubrication analysis because it contains many of the parameters that are specified by the designer. Note that it is also dimen￾sionless. The quantity r/c is called the radial clearance ratio. If we multiply both sides