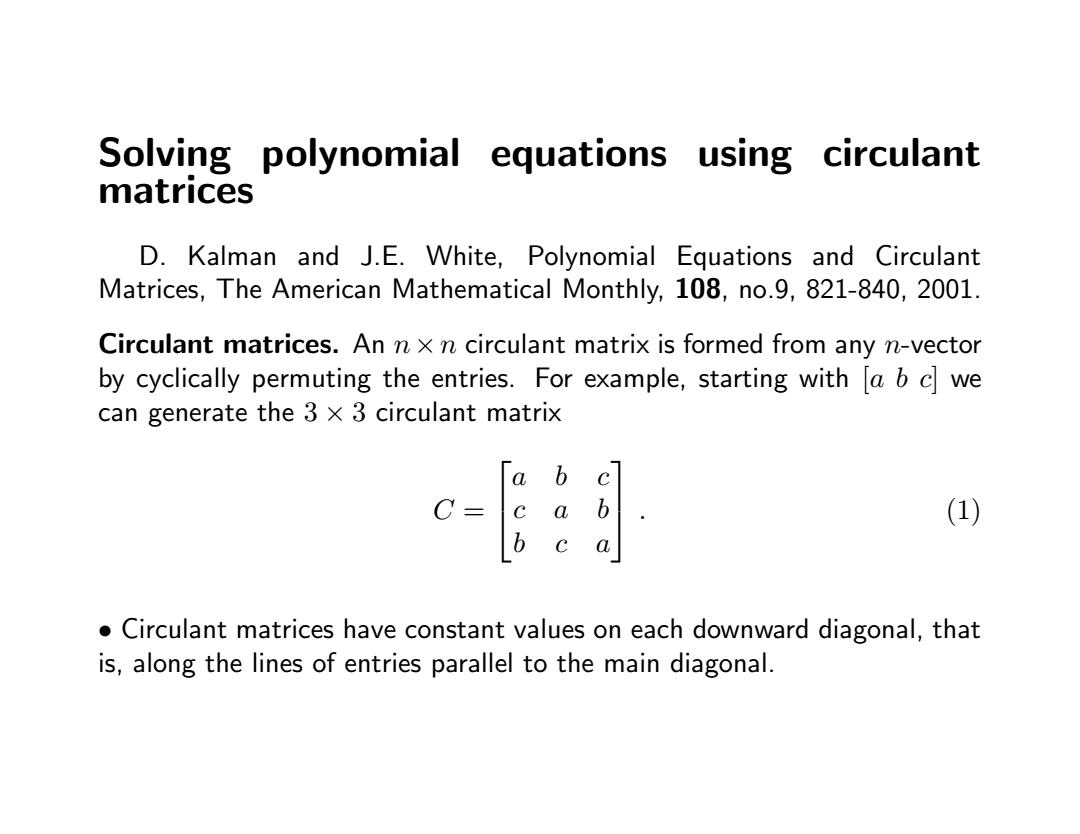
Solving polynomial equations using circulant matrices D.Kalman and J.E.White,Polynomial Equations and Circulant Matrices,The American Mathematical Monthly,108,no.9,821-840,2001. Circulant matrices.An n x n circulant matrix is formed from any n-vector by cyclically permuting the entries.For example,starting with a b c we can generate the 3 x 3 circulant matrix (a b C= a b (1) 0 Circulant matrices have constant values on each downward diagonal,that is,along the lines of entries parallel to the main diagonal.Solving polynomial equations using circulant matrices D. Kalman and J.E. White, Polynomial Equations and Circulant Matrices, The American Mathematical Monthly, 108, no.9, 821-840, 2001. Circulant matrices. An n×n circulant matrix is formed from any n-vector by cyclically permuting the entries. For example, starting with [a b c] we can generate the 3 × 3 circulant matrix C =   a b c c a b b c a   . (1) • Circulant matrices have constant values on each downward diagonal, that is, along the lines of entries parallel to the main diagonal