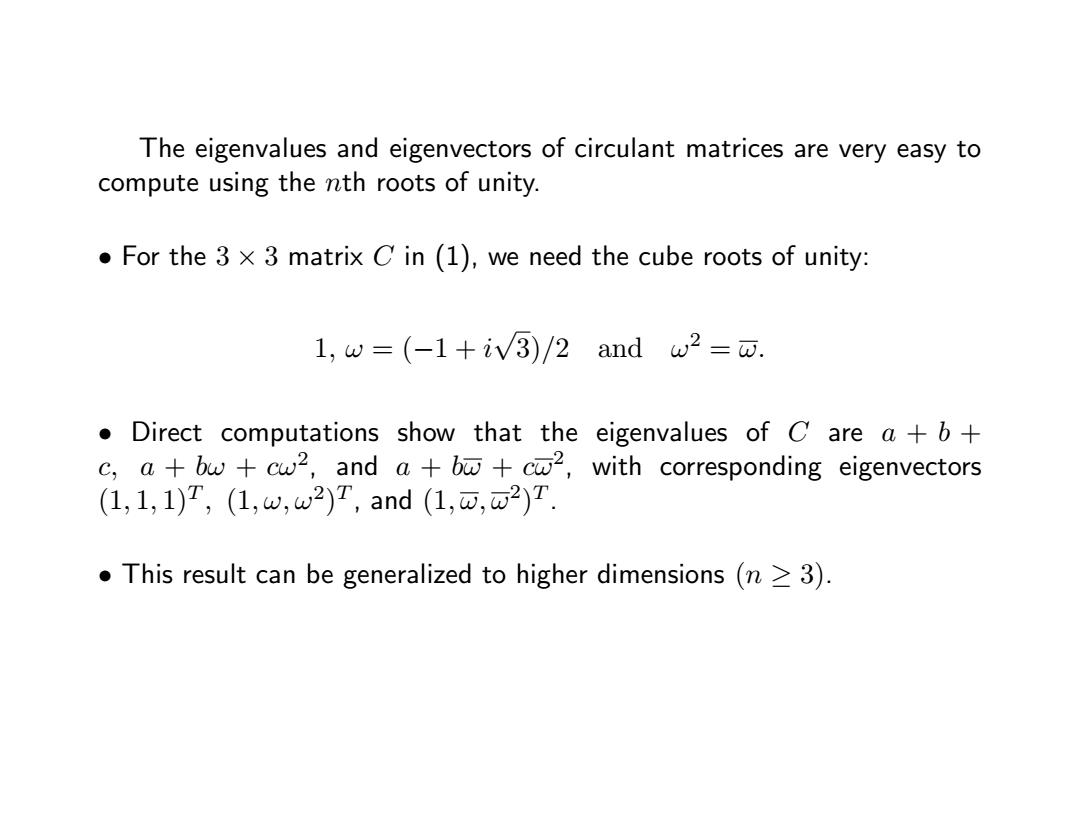
The eigenvalues and eigenvectors of circulant matrices are very easy to compute using the nth roots of unity. For the 3 x 3 matrix C in (1),we need the cube roots of unity: 1,w=(-1+iv3)/2andw2=o. Direct computations show that the eigenvalues of C are a+b+ c,a+bw +cw2,and a+bw+cw2,with corresponding eigenvectors (1,1,1)T,(1,w,w2)T,and(1,⑦，2)T. This result can be generalized to higher dimensions (n>3).The eigenvalues and eigenvectors of circulant matrices are very easy to compute using the nth roots of unity. • For the 3 × 3 matrix C in (1), we need the cube roots of unity: 1, ω = (−1 + i √ 3)/2 and ω 2 = ω. • Direct computations show that the eigenvalues of C are a + b + c, a + bω + cω2 , and a + bω + cω 2 , with corresponding eigenvectors (1, 1, 1)T , (1, ω, ω2 ) T , and (1, ω, ω 2 ) T . • This result can be generalized to higher dimensions (n ≥ 3)