正在加载图片...
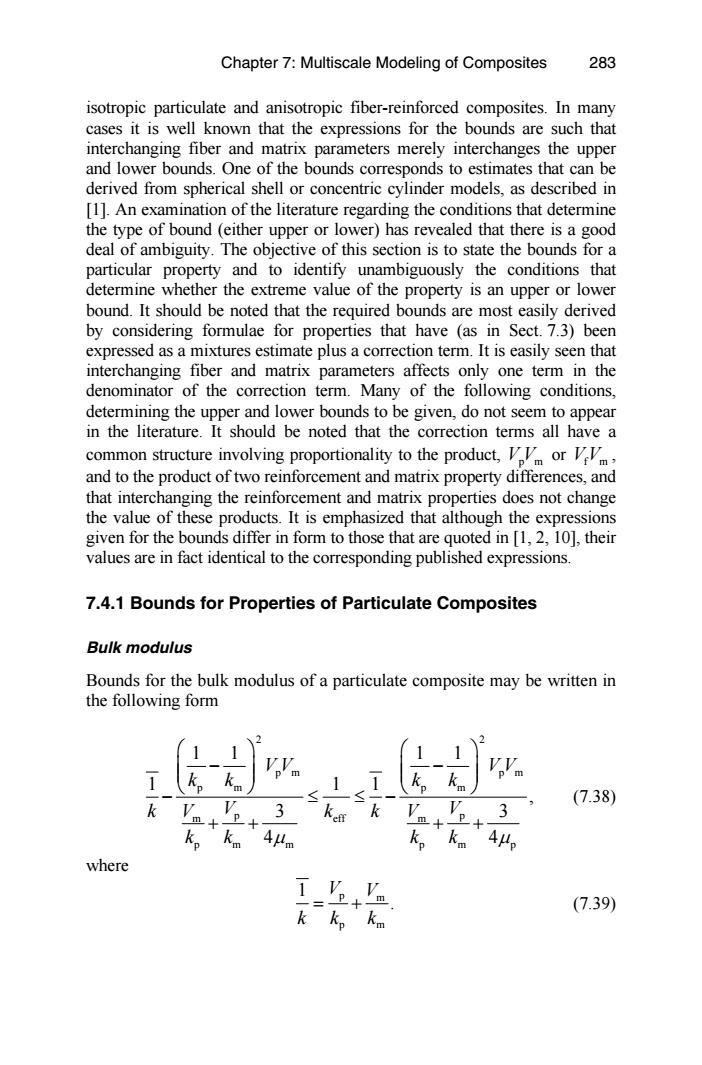
Chapter 7:Multiscale Modeling of Composites 283 isotropic particulate and anisotropic fiber-reinforced composites.In many cases it is well known that the expressions for the bounds are such that interchanging fiber and matrix parameters merely interchanges the upper and lower bounds.One of the bounds corresponds to estimates that can be derived from spherical shell or concentric cylinder models,as described in [1].An examination of the literature regarding the conditions that determine the type of bound (either upper or lower)has revealed that there is a good deal of ambiguity.The objective of this section is to state the bounds for a particular property and to identify unambiguously the conditions that determine whether the extreme value of the property is an upper or lower bound.It should be noted that the required bounds are most easily derived by considering formulae for properties that have (as in Sect.7.3)been expressed as a mixtures estimate plus a correction term.It is easily seen that interchanging fiber and matrix parameters affects only one term in the denominator of the correction term.Many of the following conditions, determining the upper and lower bounds to be given,do not seem to appear in the literature.It should be noted that the correction terms all have a common structure involving proportionality to the product,orV and to the product of two reinforcement and matrix property differences,and that interchanging the reinforcement and matrix properties does not change the value of these products.It is emphasized that although the expressions given for the bounds differ in form to those that are quoted in [1,2,10],their values are in fact identical to the corresponding published expressions. 7.4.1 Bounds for Properties of Particulate Composites Bulk modulus Bounds for the bulk modulus of a particulate composite may be written in the following form ik。k (7.38) k V 3 k。 44m k 4μ where I V V (7.39)7.4.1 Bounds for Properties of Particulate Composites Bulk modulus Bounds for the bulk modulus of a particulate composite may be written in the following form 2 2 p m p m p m p m m m p p eff pm m pm p 1 1 1 1 1 1 1 , 3 3 4 4 V V V V k k k k k k V V V V k k k µ µ k k ⎛⎞ ⎛⎞ ⎜⎟ ⎜⎟ − − ⎝⎠ ⎝⎠ − ≤ ≤ − + + + + (7.38) where p m p m 1 . V V kk k = + (7.39) Chapter 7: Multiscale Modeling of Composites isotropic particulate and anisotropic fiber-reinforced composites. In many cases it is well known that the expressions for the bounds are such that interchanging fiber and matrix parameters merely interchanges the upper and lower bounds. One of the bounds corresponds to estimates that can be derived from spherical shell or concentric cylinder models, as described in [1]. An examination of the literature regarding the conditions that determine the type of bound (either upper or lower) has revealed that there is a good deal of ambiguity. The objective of this section is to state the bounds for a particular property and to identify unambiguously the conditions that determine whether the extreme value of the property is an upper or lower bound. It should be noted that the required bounds are most easily derived by considering formulae for properties that have (as in Sect. 7.3) been expressed as a mixtures estimate plus a correction term. It is easily seen that interchanging fiber and matrix parameters affects only one term in the denominator of the correction term. Many of the following conditions, determining the upper and lower bounds to be given, do not seem to appear in the literature. It should be noted that the correction terms all have a common structure involving proportionality to the product, V Vp m or V Vf m , and to the product of two reinforcement and matrix property differences, and that interchanging the reinforcement and matrix properties does not change the value of these products. It is emphasized that although the expressions given for the bounds differ in form to those that are quoted in [1, 2, 10], their values are in fact identical to the corresponding published expressions. 283