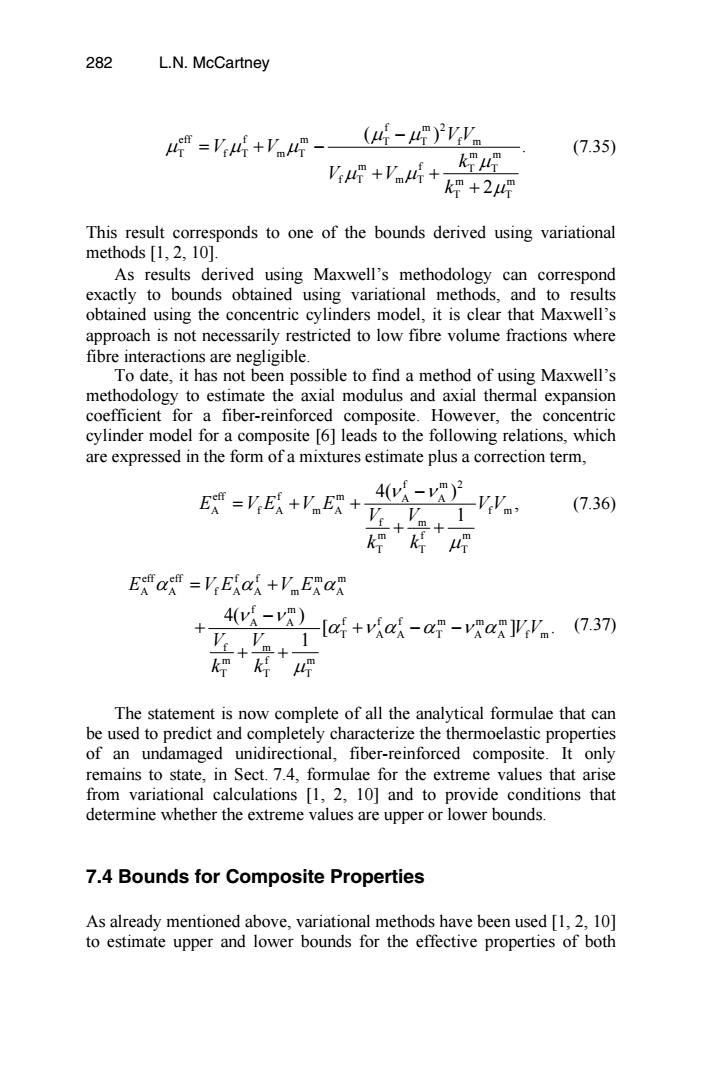
282 L.N.McCartney 4=+4- (4-4)V (7.35) ++9 "+2 This result corresponds to one of the bounds derived using variational methods [1,2,10]. As results derived using Maxwell's methodology can correspond exactly to bounds obtained using variational methods,and to results obtained using the concentric cylinders model,it is clear that Maxwell's approach is not necessarily restricted to low fibre volume fractions where fibre interactions are negligible. To date,it has not been possible to find a method of using Maxwell's methodology to estimate the axial modulus and axial thermal expansion coefficient for a fiber-reinforced composite.However,the concentric cylinder model for a composite [6]leads to the following relations,which are expressed in the form of a mixtures estimate plus a correction term, EA=VEA+VER+ 4vs-v3-VV (7.36) 十 EAM aA =VEXCA+VEROR G+以ad-ag-吹a以.an + k味 The statement is now complete of all the analytical formulae that can be used to predict and completely characterize the thermoelastic properties of an undamaged unidirectional,fiber-reinforced composite.It only remains to state,in Sect.7.4,formulae for the extreme values that arise from variational calculations [1,2,10]and to provide conditions that determine whether the extreme values are upper or lower bounds. 7.4 Bounds for Composite Properties As already mentioned above,variational methods have been used [1,2,10] to estimate upper and lower bounds for the effective properties of bothf m2 eff f m T T fm T f T mT m m m f T T f T mT m m T T ( ) . 2 V V V V k V V k µ µ µ µµ µ µ µ µ − =+ − + + + (7.35) This result corresponds to one of the bounds derived using variational methods [1, 2, 10]. To date, it has not been possible to find a method of using Maxwell’s methodology to estimate the axial modulus and axial thermal expansion coefficient for a fiber-reinforced composite. However, the concentric cylinder model for a composite [6] leads to the following relations, which are expressed in the form of a mixtures estimate plus a correction term, f m2 eff f m A A A f A mA f m f m mf m TTT 4( ) , 1 E VE V E V V V V k k ν ν µ − =+ + + + (7.36) eff eff f f m m A A f AA mA A f m A A f f f m mm T AA T A A fm f m mf m TTT 4( ) [ ] . 1 E VE V E V V V V k k α αα ν ν α να α να µ = + − + + − − + + (7.37) The statement is now complete of all the analytical formulae that can be used to predict and completely characterize the thermoelastic properties of an undamaged unidirectional, fiber-reinforced composite. It only remains to state, in Sect. 7.4, formulae for the extreme values that arise from variational calculations [1, 2, 10] and to provide conditions that determine whether the extreme values are upper or lower bounds. 7.4 Bounds for Composite Properties As already mentioned above, variational methods have been used [1, 2, 10] to estimate upper and lower bounds for the effective properties of both L.N. McCartney As results derived using Maxwell’s methodology can correspond exactly to bounds obtained using variational methods, and to results obtained using the concentric cylinders model, it is clear that Maxwell’s approach is not necessarily restricted to low fibre volume fractions where fibre interactions are negligible. 282