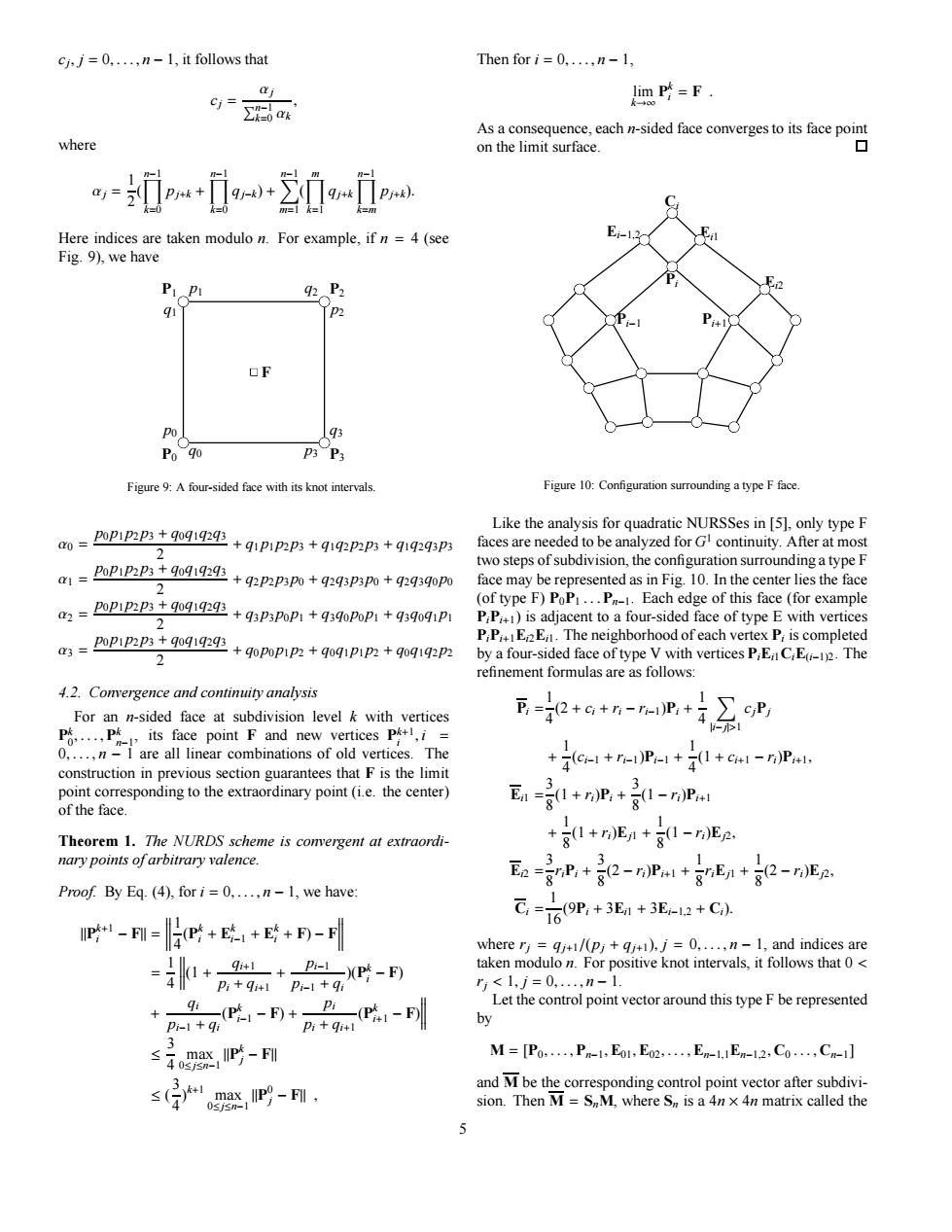
Then fo. 9-m where ieahd6eomegsoisacep g=0p-jwr2e-ie… eod For cample.( dine a type Fto -n4n2,ppM+An+M -P产Pa+AP+nP片+I9R d.2.Comerge -2+6+--+9 +C+1-n of ue ee 工-3+np+2-njP4 ++E+-re Froof By Ed.()or-1.we have 瓦aP+2-nP1+En+2-nEe C=p,+3北+3a+C I--r+,+时+)-r -1+n+- M=...EE. Te光eon物m cj , j = 0, . . . , n − 1, it follows that cj = αj Pn−1 k=0 αk , where αj = 1 2 ( Yn−1 k=0 pj+k + Yn−1 k=0 qj−k) + Xn−1 m=1 ( Ym k=1 qj+k Yn−1 k=m pj+k). Here indices are taken modulo n. For example, if n = 4 (see Fig. 9), we have bc bc bc bc rsF P0 p0 q0 P1 p1 q1 P2 p2 q2 P3 p3 q3 Figure 9: A four-sided face with its knot intervals. α0 = p0 p1 p2 p3 + q0q1q2q3 2 + q1 p1 p2 p3 + q1q2 p2 p3 + q1q2q3 p3 α1 = p0 p1 p2 p3 + q0q1q2q3 2 + q2 p2 p3 p0 + q2q3 p3 p0 + q2q3q0 p0 α2 = p0 p1 p2 p3 + q0q1q2q3 2 + q3 p3 p0 p1 + q3q0 p0 p1 + q3q0q1 p1 α3 = p0 p1 p2 p3 + q0q1q2q3 2 + q0 p0 p1 p2 + q0q1 p1 p2 + q0q1q2 p2 4.2. Convergence and continuity analysis For an n-sided face at subdivision level k with vertices P k 0 , . . . , P k n−1 , its face point F and new vertices P k+1 i , i = 0, . . . , n − 1 are all linear combinations of old vertices. The construction in previous section guarantees that F is the limit point corresponding to the extraordinary point (i.e. the center) of the face. Theorem 1. The NURDS scheme is convergent at extraordi￾nary points of arbitrary valence. Proof. By Eq. (4), for i = 0, . . . , n − 1, we have: kP k+1 i − Fk = 1 4 (P k i + E k i−1 + E k i + F) − F = 1 4 (1 + qi+1 pi + qi+1 + pi−1 pi−1 + qi )(P k i − F) + qi pi−1 + qi (P k i−1 − F) + pi pi + qi+1 (P k i+1 − F) ≤ 3 4 max 0≤j≤n−1 kP k j − Fk ≤ ( 3 4 ) k+1 max 0≤j≤n−1 kP 0 j − Fk , Then for i = 0, . . . , n − 1, lim k→∞ P k i = F . As a consequence, each n-sided face converges to its face point on the limit surface. Ci Ei−1,2 Ei1 Ei2 Pi Pi−1 Pi+1 bc bc bc bc bc bc bc bc bc bc bc bc bc bc bc bc bc bc bc bc Figure 10: Configuration surrounding a type F face. Like the analysis for quadratic NURSSes in [5], only type F faces are needed to be analyzed for G 1 continuity. After at most two steps of subdivision, the configuration surrounding a type F face may be represented as in Fig. 10. In the center lies the face (of type F) P0P1 . . .Pn−1. Each edge of this face (for example PiPi+1) is adjacent to a four-sided face of type E with vertices PiPi+1Ei2Ei1. The neighborhood of each vertex Pi is completed by a four-sided face of type V with vertices PiEi1CiE(i−1)2. The refinement formulas are as follows: Pi = 1 4 (2 + ci + ri − ri−1)Pi + 1 4 X |i−j|>1 cjPj + 1 4 (ci−1 + ri−1)Pi−1 + 1 4 (1 + ci+1 − ri)Pi+1, Ei1 = 3 8 (1 + ri)Pi + 3 8 (1 − ri)Pi+1 + 1 8 (1 + ri)Ej1 + 1 8 (1 − ri)Ej2, Ei2 = 3 8 riPi + 3 8 (2 − ri)Pi+1 + 1 8 riEj1 + 1 8 (2 − ri)Ej2, Ci = 1 16 (9Pi + 3Ei1 + 3Ei−1,2 + Ci). where rj = qj+1/(pj + qj+1), j = 0, . . . , n − 1, and indices are taken modulo n. For positive knot intervals, it follows that 0 < rj < 1, j = 0, . . . , n − 1. Let the control point vector around this type F be represented by M = [P0, . . . , Pn−1, E01, E02, . . . , En−1,1En−1,2, C0 . . . , Cn−1] and M be the corresponding control point vector after subdivi￾sion. Then M = SnM, where Sn is a 4n × 4n matrix called the 5