正在加载图片...
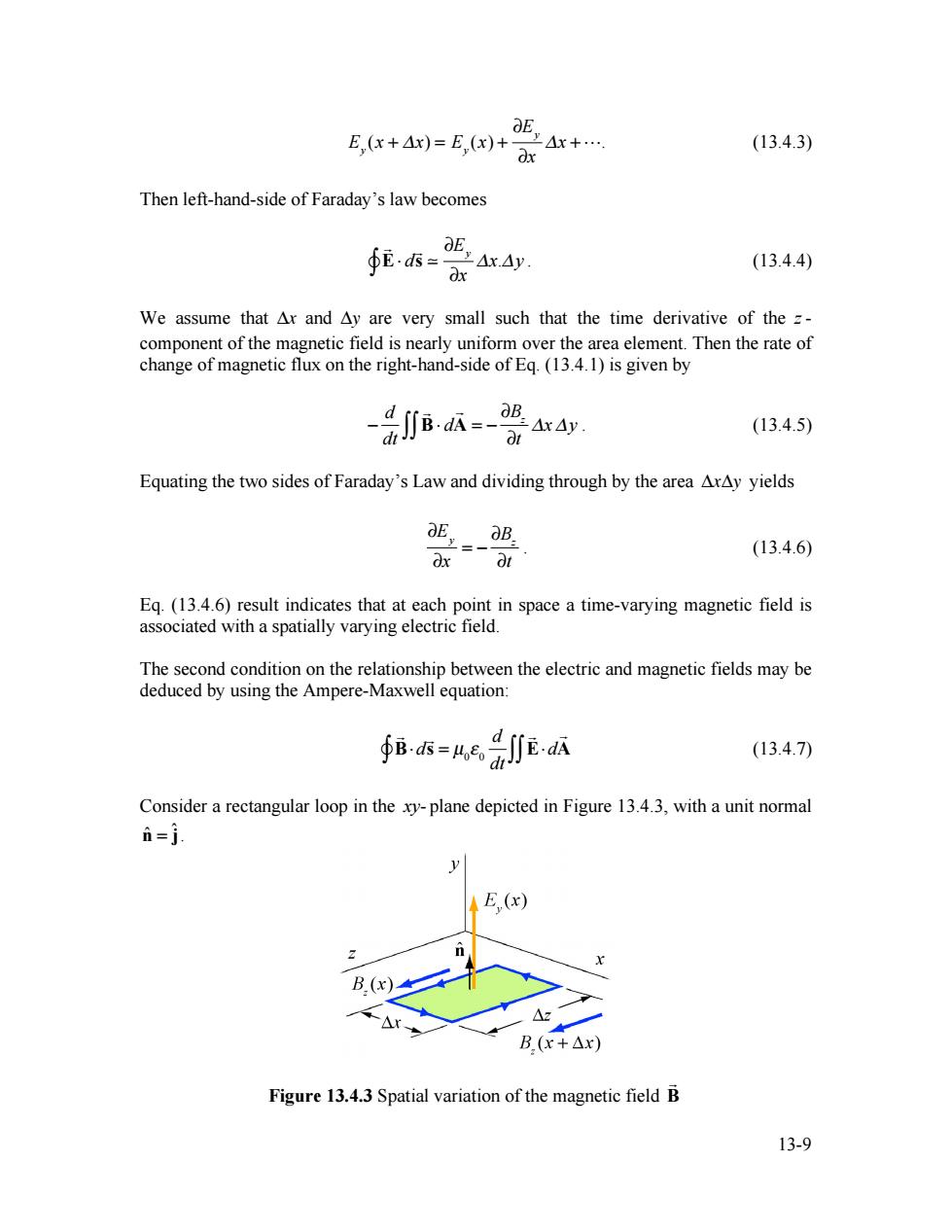
aEAx+… E.(x+Ax)=E,(x)+x (13.4.3) Then left-hand-side of Faraday's law becomes ∮Es= E2△xAy (13.4.4) Ox We assume that Ar and Ay are very small such that the time derivative of the = component of the magnetic field is nearly uniform over the area element.Then the rate of change of magnetic flux on the right-hand-side of Eq.(13.4.1)is given by s=- B△x△y, (13.4.5) Equating the two sides of Faraday's Law and dividing through by the area AxAy yields (13.4.6) Eq.(13.4.6)result indicates that at each point in space a time-varying magnetic field is associated with a spatially varying electric field. The second condition on the relationship between the electric and magnetic fields may be deduced by using the Ampere-Maxwell equation: B西=4气Ea (13.4.7) Consider a rectangular loop in the xy-plane depicted in Figure 13.4.3,with a unit normal n=j. E,(x) B.(x) △X 42 B(x+△x) Figure 13.4.3 Spatial variation of the magnetic field B 13-913-9 E y (x + !x) = E y (x) + "E y "x !x +!. (13.4.3) Then left-hand-side of Faraday’s law becomes ! E! d ! s "" # #E y #x $x.$y . (13.4.4) We assume that !x and !y are very small such that the time derivative of the z - component of the magnetic field is nearly uniform over the area element. Then the rate of change of magnetic flux on the right-hand-side of Eq. (13.4.1) is given by ! d dt ! B" d ! ## A = ! $Bz $t %x %y . (13.4.5) Equating the two sides of Faraday’s Law and dividing through by the area !x!y yields !E y !x = " !Bz !t . (13.4.6) Eq. (13.4.6) result indicates that at each point in space a time-varying magnetic field is associated with a spatially varying electric field. The second condition on the relationship between the electric and magnetic fields may be deduced by using the Ampere-Maxwell equation: ! B!d ! s = µ0 " 0 d dt "# ! E!d ! ## A (13.4.7) Consider a rectangular loop in the xy- plane depicted in Figure 13.4.3, with a unit normal ˆ nˆ = j. Figure 13.4.3 Spatial variation of the magnetic field B !