正在加载图片...
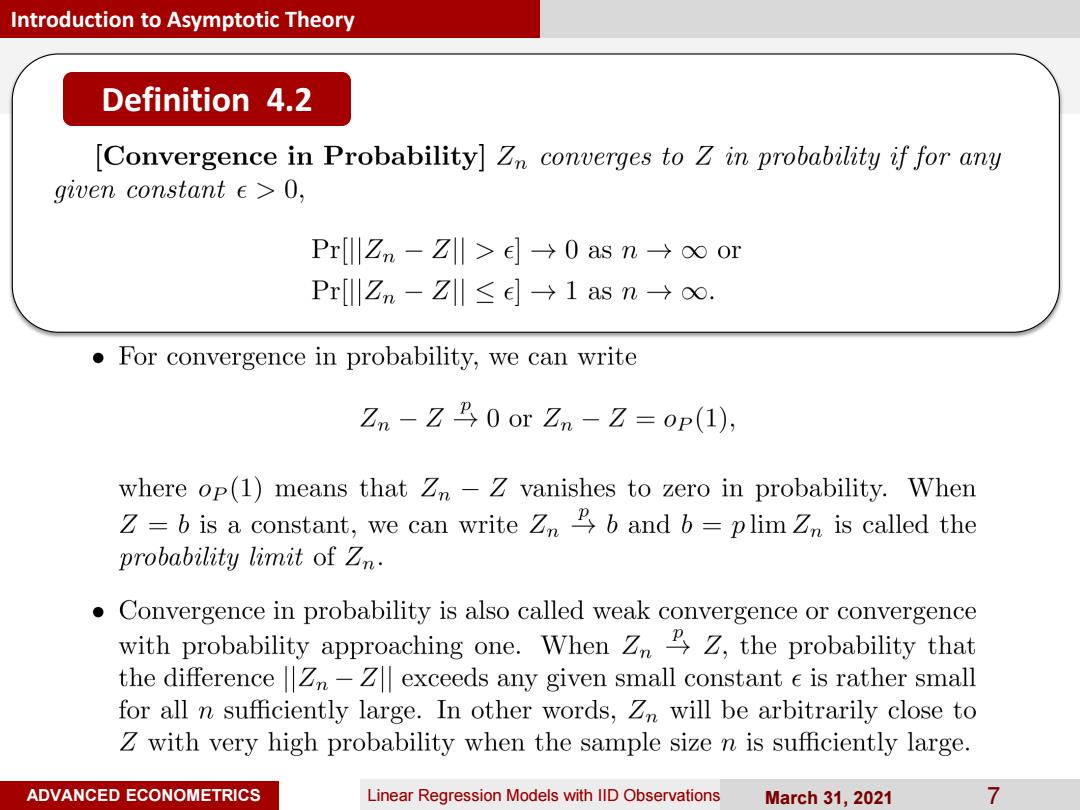
Introduction to Asymptotic Theory Definition 4.2 [Convergence in Probability]Zn converges to Z in probability if for any given constant e>0, Pr[Zm-Z>e→0asn→oor Pr[lZn-Z≤d→1asn→oo. For convergence in probability,we can write Zn-Z0 or Zn -Z=op(1), where op(1)means that Zn-Z vanishes to zero in probability.When Z=b is a constant,we can write Zn2b and b=plim Zn is called the probability limit of Zn. Convergence in probability is also called weak convergence or convergence with probability approaching one.When Zn2,the probability that the differencen-exceeds any given small constant e is rather small for all n sufficiently large.In other words,Zn will be arbitrarily close to Z with very high probability when the sample size n is sufficiently large. ADVANCED ECONOMETRICS Linear Regression Models with IID Observations March 31,2021 7ADVANCED ECONOMETRICS Linear Regression Models with IID Observations March 31, 2021 7 Introduction to Asymptotic Theory Definition 4.2