
中国科学院数学与系统科学研究院 Academy of Mathematics and Systems Science Chinese Academy of Sciences Linear Regression Models with IID Observations Professor Yongmiao Hong March 2021
Professor Yongmiao Hong March 2021 Linear Regression Models with IID Observations

CONTENTS 4.1 Introduction to Asymptotic Theory 4.2 Framework and Assumptions 4.3 Consistency of OLS 4.4 Asymptotic Normality of OLS 4.5 Asymptotic Variance Estimation 4.6 Hypothesis Testing 4.7 Testing for Conditional Homoskedasticity 4.8 Conclusion ADVANCED ECONOMETRICS Linear Regression Models with IID Observations March 31,2021 2
ADVANCED ECONOMETRICS Linear Regression Models with IID Observations March 31, 2021 2 4.1 Introduction to Asymptotic Theory 4.2 Framework and Assumptions 4.3 Consistency of OLS 4.4 Asymptotic Normality of OLS 4.5 Asymptotic Variance Estimation 4.6 Hypothesis Testing 4.7 Testing for Conditional Homoskedasticity 4.8 Conclusion CONTENTS

Introduction to Asymptotic Theory The assumptions of classical linear regression models are rather strong. For example,the conditional normality condition on e is crucial to obtain the finite sample distributions of the OLS,GLS and related test statistics, but it has been documented that most economic and financial data have heavy tails. An interesting question is whether the estimators and tests which are based on the same principles as before still make sense in this more general setting. In particular,what happens to the OLS estimator,the t-and F-tests if any of the following assumptions fails? strict exogeneity E(etX)=0; normality (eX~N(0,021); conditional homoskedasticity (var(etX)=g2); auto uncorrelatedness (cov(et,esX)=0 for ts). ADVANCED ECONOMETRICS Linear Regression Models with IID Observations March 31,2021 3
ADVANCED ECONOMETRICS Linear Regression Models with IID Observations March 31, 2021 3 Introduction to Asymptotic Theory

Introduction to Asymptotic Theory We can pose the question how estimators and test statistics would behave when the number of observations increases without limit.This is called asymptotic analysis.For more systematic introduction of asymptotic the- ory,see,for example,White (1994,2001)and Davidson (1994). To assess how close the OLS estimator B is to the true parameter value Bo and to derive its asymptotic distribution (after suitable normalization),we briefly review some important convergence concepts and limit theorems. ADVANCED ECONOMETRICS Linear Regression Models with IID Observations March 31,2021 4
ADVANCED ECONOMETRICS Linear Regression Models with IID Observations March 31, 2021 4 Introduction to Asymptotic Theory
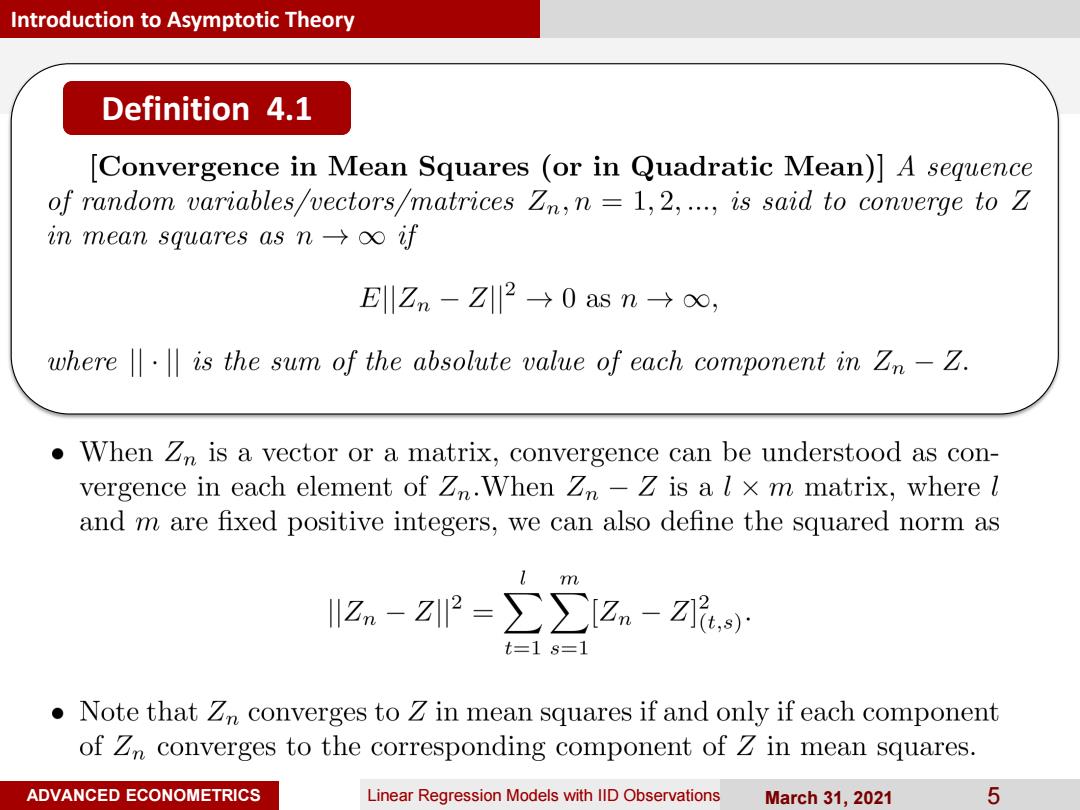
Introduction to Asymptotic Theory Definition 4.1 [Convergence in Mean Squares (or in Quadratic Mean)]A sequence of random variables/vectors/matrices Zn,n=1,2,...,is said to converge to Z in mean squares as n->oo if ElZm-Z2→0asn→o, where is the sum of the absolute value of each component in Zn -Z. When Zn is a vector or a matrix,convergence can be understood as con- vergence in each element of Zn.When Zn-Z is a l x m matrix,where l and m are fixed positive integers,we can also define the squared norm as Z-Z2=∑∑Zm-☑ t=1s=1 Note that 2n converges to 2 in mean squares if and only if each component of Zn converges to the corresponding component of Z in mean squares. ADVANCED ECONOMETRICS Linear Regression Models with IID Observations March 31,2021 5
ADVANCED ECONOMETRICS Linear Regression Models with IID Observations March 31, 2021 5 Introduction to Asymptotic Theory Definition 4.1
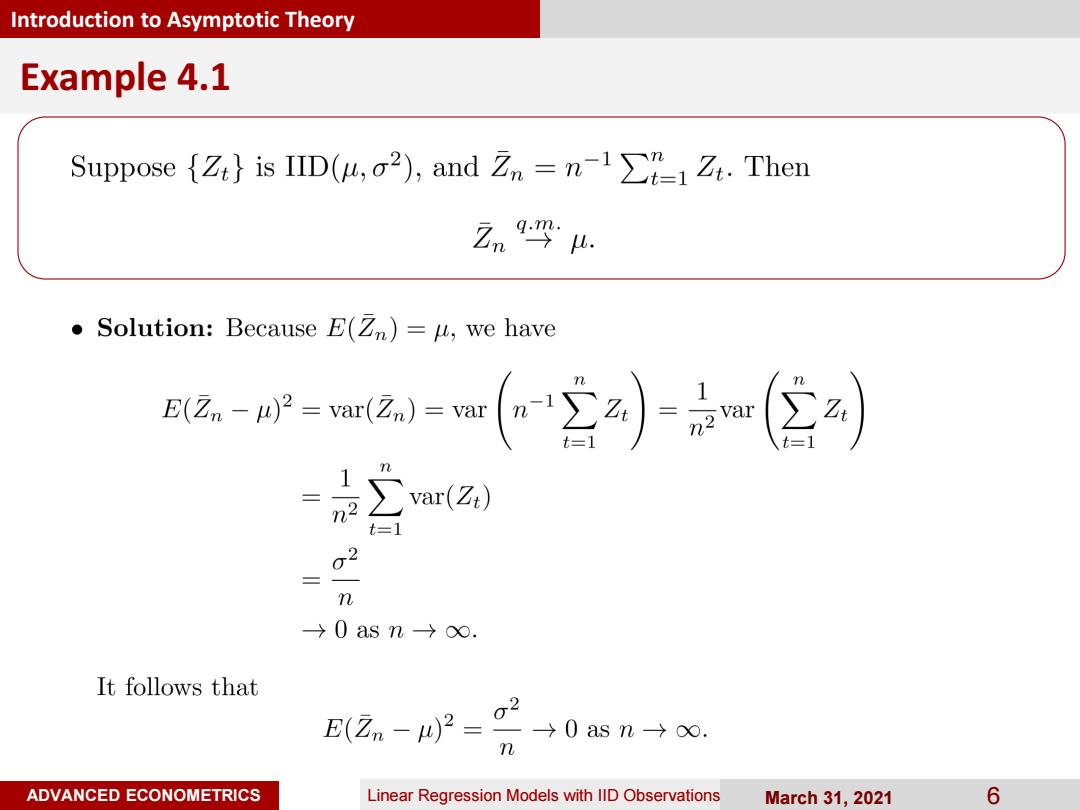
Introduction to Asymptotic Theory Example 4.1 Suppose {is IID(u,2),and Zn=n-1Z.Then 7n9y4. Solution:Because E(Zn)=u,we have 以原-w以-(空4-京店 1 var(Zt) t=1 n -→0asn→o. It follows that E2,-P2=g2→0asn→c m ADVANCED ECONOMETRICS Linear Regression Models with IID Observations March 31,2021 6
ADVANCED ECONOMETRICS Linear Regression Models with IID Observations March 31, 2021 6 Introduction to Asymptotic Theory Example 4.1
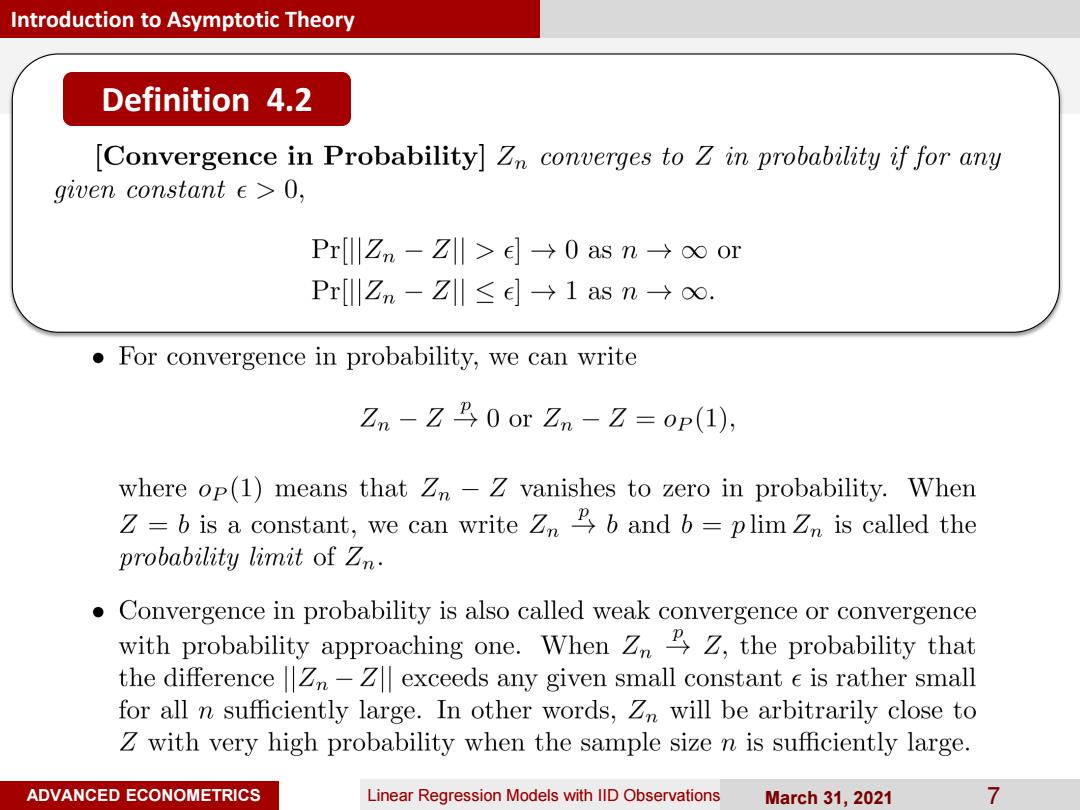
Introduction to Asymptotic Theory Definition 4.2 [Convergence in Probability]Zn converges to Z in probability if for any given constant e>0, Pr[Zm-Z>e→0asn→oor Pr[lZn-Z≤d→1asn→oo. For convergence in probability,we can write Zn-Z0 or Zn -Z=op(1), where op(1)means that Zn-Z vanishes to zero in probability.When Z=b is a constant,we can write Zn2b and b=plim Zn is called the probability limit of Zn. Convergence in probability is also called weak convergence or convergence with probability approaching one.When Zn2,the probability that the differencen-exceeds any given small constant e is rather small for all n sufficiently large.In other words,Zn will be arbitrarily close to Z with very high probability when the sample size n is sufficiently large. ADVANCED ECONOMETRICS Linear Regression Models with IID Observations March 31,2021 7
ADVANCED ECONOMETRICS Linear Regression Models with IID Observations March 31, 2021 7 Introduction to Asymptotic Theory Definition 4.2
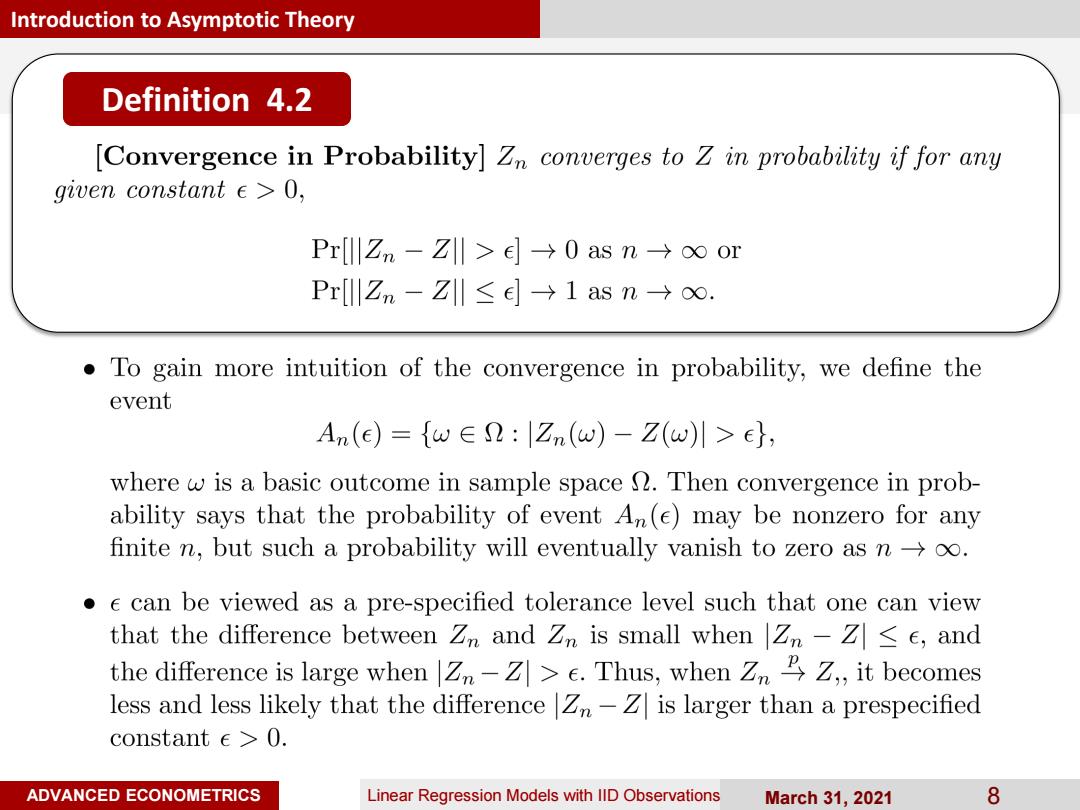
Introduction to Asymptotic Theory Definition 4.2 [Convergence in Probability]Zn converges to Z in probability if for any given constant e>0, Pr[Zm-Z>e→0asn→oor Pr[lZm-Z≤e→1asn→o. To gain more intuition of the convergence in probability,we define the event An(e)={w∈2:|Zn(w)-Z(w)川>e}, where w is a basic outcome in sample space n.Then convergence in prob- ability says that the probability of event An(e)may be nonzero for any finite n,but such a probability will eventually vanish to zero as n->oo. e can be viewed as a pre-specified tolerance level such that one can view that the difference between Zn and Zn is small when Zn-Ze.Thus,when ZnBZ,,it becomes less and less likely that the difference Zn-is larger than a prespecified constant e>0. ADVANCED ECONOMETRICS Linear Regression Models with IID Observations March 31,2021 8
ADVANCED ECONOMETRICS Linear Regression Models with IID Observations March 31, 2021 8 Introduction to Asymptotic Theory Definition 4.2
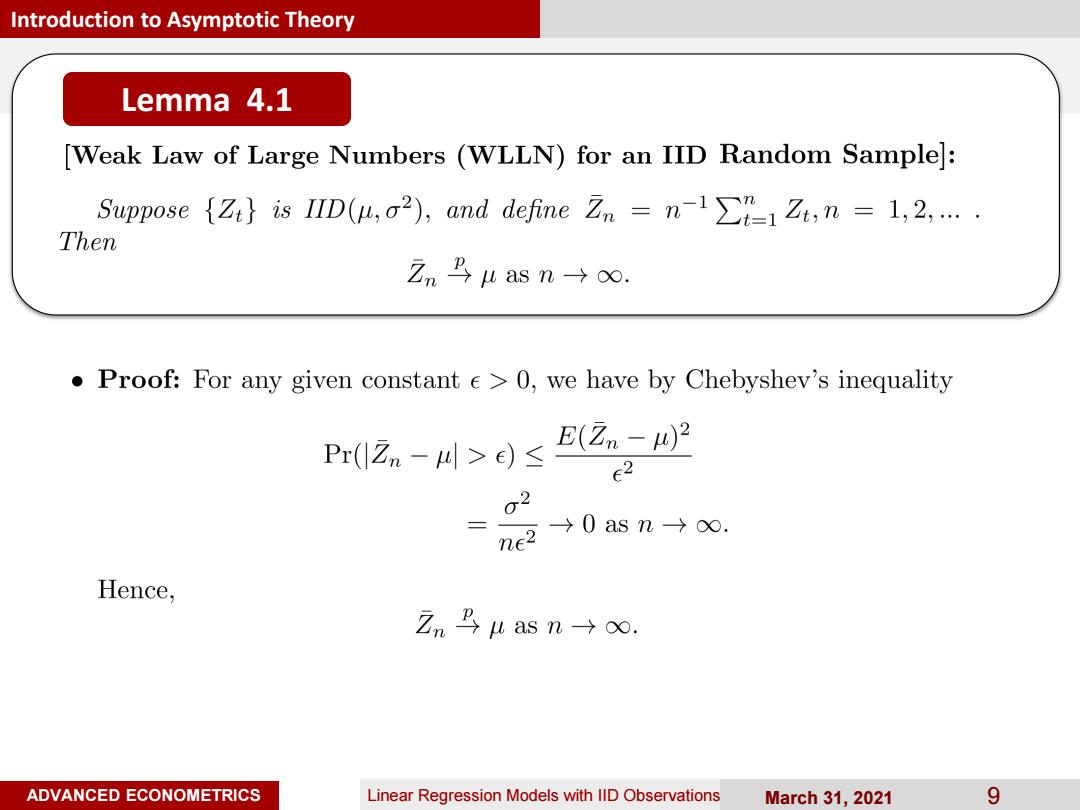
Introduction to Asymptotic Theory Lemma 4.1 [Weak Law of Large Numbers (WLLN)for an IID Random Sample]: Suppose (Z}is IID(u,2),and define Zn =nZ,n =1,2..... Then ZmB4asn→o. Proof:For any given constant e>0,we have by Chebyshev's inequality Pr1Z-川>)≤E2-四2 E2 03 ne2→0asno. Hence, Zn B u as n→o. ADVANCED ECONOMETRICS Linear Regression Models with IID Observations March 31,2021 9
ADVANCED ECONOMETRICS Linear Regression Models with IID Observations March 31, 2021 9 Introduction to Asymptotic Theory Lemma 4.1

Introduction to Asymptotic Theory Example 4.2 Buy and Hold Trading Strategy and Economic Interpretation of WLLN In finance,there is a popular trading strategy called buy-and-hold trading strategy.An investor buys a stock at some day and then hold it for a long time period before he sells it out.This is called a buy-and-hold trading strategy. How is the average return of this trading strategy? Suppose Zt is the return of the stock on period t,and the returns over different time periods are IID(u,o2).Also assume the investor holds the stock for a total of n period.Then the average return over each time period is the sample mean t=1 When the number n of holding periods is large,we have =E(Z) as n->oo.Thus,the average return of the buy-and-hold trading strategy is approximately equal to u when n is sufficiently large. ADVANCED ECONOMETRICS Linear Regression Models with IID Observations March 31,2021 10
ADVANCED ECONOMETRICS Linear Regression Models with IID Observations March 31, 2021 10 Introduction to Asymptotic Theory Example 4.2 Buy and Hold Trading Strategy and Economic Interpretation of WLLN