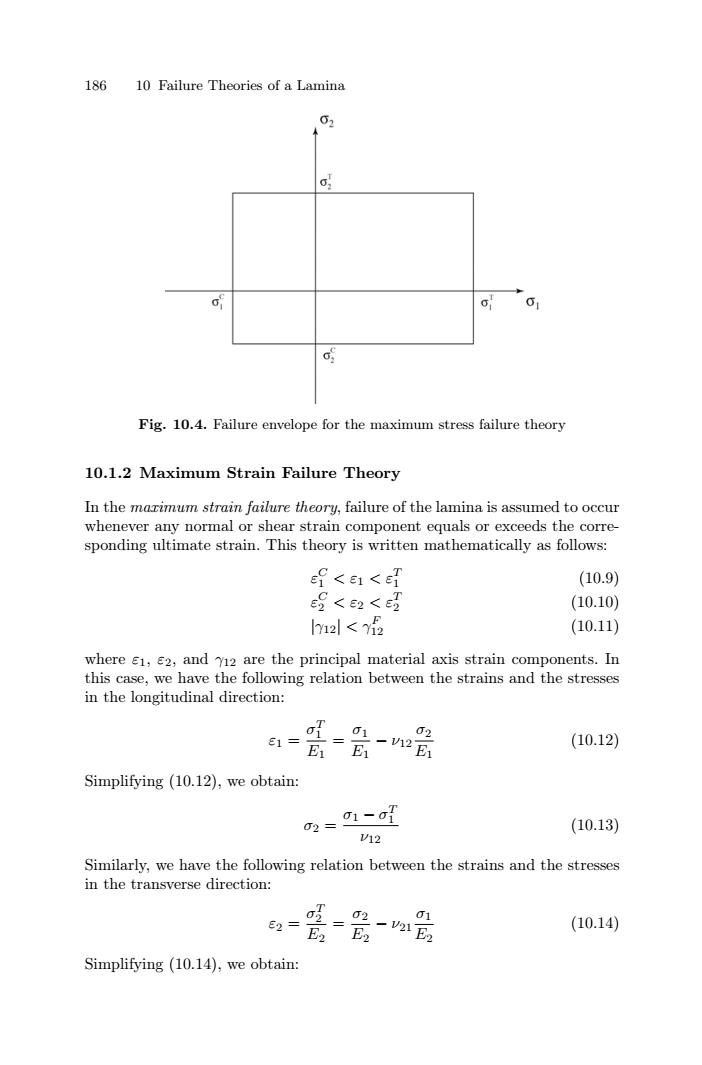
186 10 Failure Theories of a Lamina 0 o Fig.10.4.Failure envelope for the maximum stress failure theory 10.1.2 Maximum Strain Failure Theory In the marimum strain failure theory,failure of the lamina is assumed to occur whenever any normal or shear strain component equals or exceeds the corre- sponding ultimate strain.This theory is written mathematically as follows: ef <E1<ET (10.9) 8<e2< T (10.10) mal< (10.11) where E1,E2,and Y12 are the principal material axis strain components.In this case,we have the following relation between the strains and the stresses in the longitudinal direction: 61= 02 E1 01一2E1 E1 (10.12) Simplifying (10.12),we obtain: 01-a1 02= (10.13) 12 Similarly,we have the following relation between the strains and the stresses in the transverse direction: E2= 02 01 E2E 一21 (10.14) Simplifying (10.14),we obtain:186 10 Failure Theories of a Lamina Fig. 10.4. Failure envelope for the maximum stress failure theory 10.1.2 Maximum Strain Failure Theory In the maximum strain failure theory, failure of the lamina is assumed to occur whenever any normal or shear strain component equals or exceeds the corre￾sponding ultimate strain. This theory is written mathematically as follows: εC 1 < ε1 < εT 1 (10.9) εC 2 < ε2 < εT 2 (10.10) |γ12| < γF 12 (10.11) where ε1, ε2, and γ12 are the principal material axis strain components. In this case, we have the following relation between the strains and the stresses in the longitudinal direction: ε1 = σT 1 E1 = σ1 E1 − ν12 σ2 E1 (10.12) Simplifying (10.12), we obtain: σ2 = σ1 − σT 1 ν12 (10.13) Similarly, we have the following relation between the strains and the stresses in the transverse direction: ε2 = σT 2 E2 = σ2 E2 − ν21 σ1 E2 (10.14) Simplifying (10.14), we obtain: