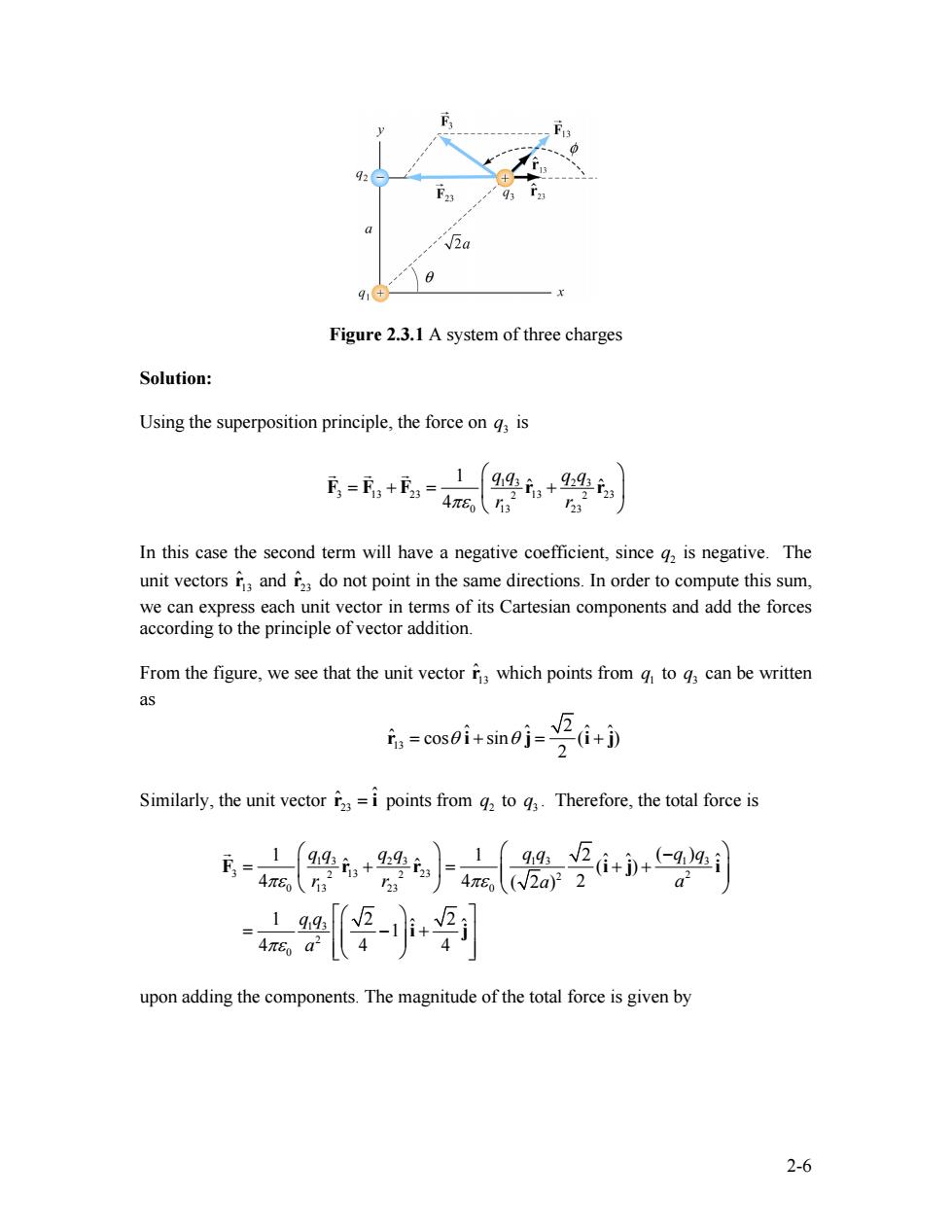
1 92- a 91+ Figure 2.3.1 A system of three charges Solution: Using the superposition principle,the force on g:is 10 E=+= 49i+99i In this case the second term will have a negative coefficient,since g2 is negative.The unit vectors and do not point in the same directions.In order to compute this sum, we can express each unit vector in terms of its Cartesian components and add the forces according to the principle of vector addition. From the figure,we see that the unit vector r which points from g to g3 can be written as -oim0j-9i+i Similarly,the unit vector=i points from to Therefore,the total force is F= 1 警+学小{%n, (2a)22 1 4T60 upon adding the components.The magnitude of the total force is given by 2-6Figure 2.3.1 A system of three charges Solution: Using the superposition principle, the force on is 3 q 1 3 2 3 3 13 23 2 2 13 23 0 13 23 1 ˆ ˆ 4 q q q q πε r r ⎛ ⎞ = + = ⎜ ⎟ + ⎝ ⎠ F F F r r G G G In this case the second term will have a negative coefficient, since is negative. The unit vectors and do not point in the same directions. In order to compute this sum, we can express each unit vector in terms of its Cartesian components and add the forces according to the principle of vector addition. 2 q 13 rˆ 23 rˆ From the figure, we see that the unit vector which points from to can be written as 13 rˆ 1 q 3 q 13 2 ˆ ˆ ˆ ˆ cos sin ( ) 2 ˆ r i = θ θ + =j i + j Similarly, the unit vector points from to . Therefore, the total force is 23 ˆ rˆ = i 2 q 3 q 1 3 2 3 1 3 1 3 3 1 2 2 3 23 2 2 0 13 23 0 1 3 2 0 1 1 2 ( ) ˆ ˆ ˆ ˆ ˆ ( ) 4 4 ( 2 ) 2 1 2 2 ˆ ˆ 1 4 4 4 q q q q q q q q r r a a q q a πε πε πε ⎛ ⎞ ⎛ ⎞ − = + ⎜ ⎟ = ⎜ ⎟ + + ⎝ ⎠ ⎝ ⎠ ⎡ ⎤ ⎛ ⎞ = − ⎢ ⎥ ⎜ ⎟ + ⎣ ⎦ ⎝ ⎠ F r r i j i i j G upon adding the components. The magnitude of the total force is given by 2-6