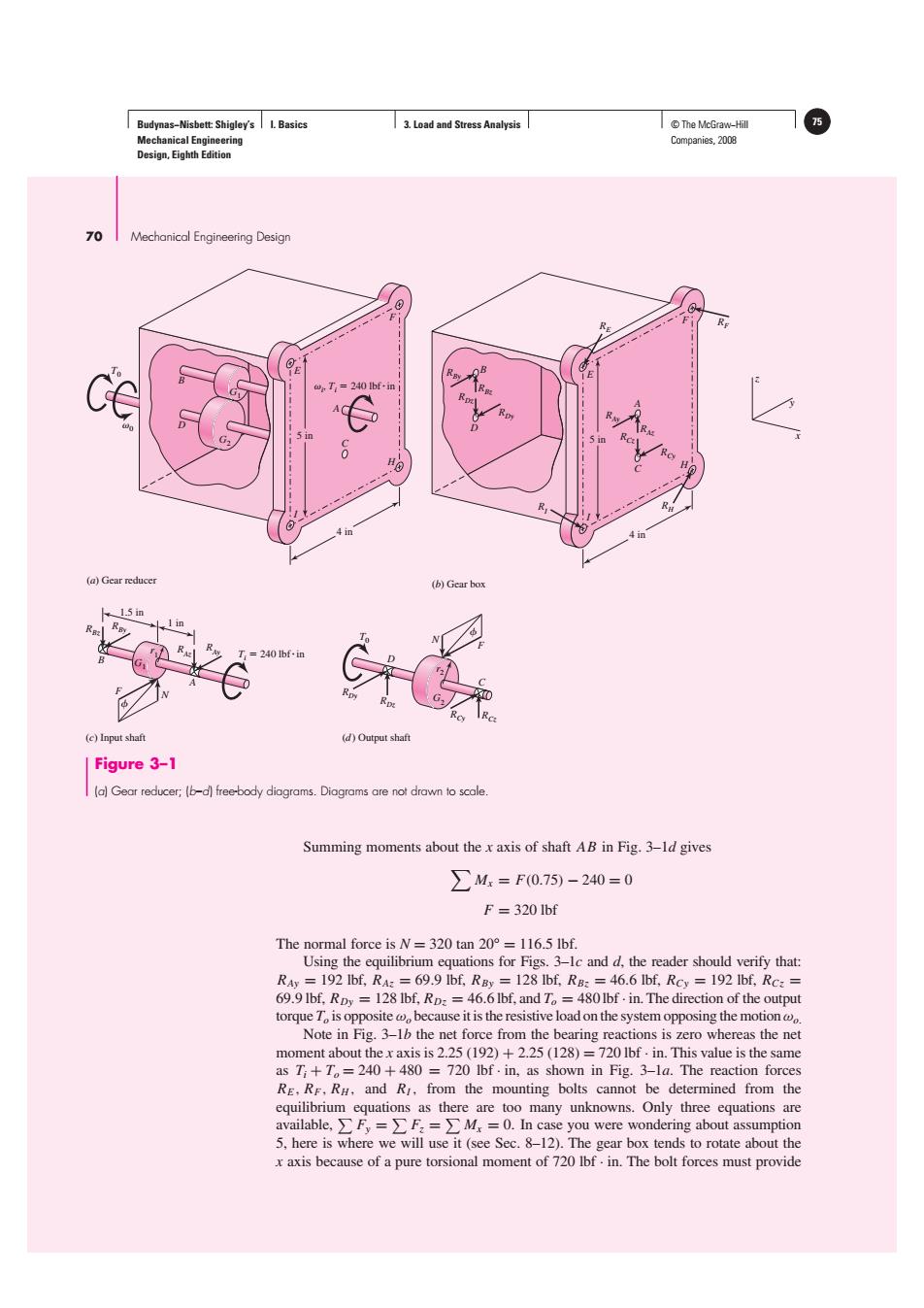
Budynas-Nisbett:Shigley's I.Basics 3.Load and Stress Analysis T©The McGraw-Hil Mechanical Engineering Companies,2008 Design,Eighth Edition 70 Mechanical Engineering Design urT,=240bfin R in (a)Gear reducer (b)Gear box 1.5in =240bfin (c)Input shaft (d)Output shaft Figure 3-1 (a)Gear reducer;(b-d)free-body diagrams.Diagrams are not drawn to scale. Summing moments about the x axis of shaft AB in Fig.3-ld gives ∑Mx=F0.75)-240=0 F =320 lbf The normal force is N=320 tan 20=116.5 lbf. Using the equilibrium equations for Figs.3-lc and d,the reader should verify that: RAy =192 Ibf,RA:=69.9 Ibf,RBy 128 Ibf,RB:=46.6 Ibf,RCy 192 Ibf,Rc:= 69.9 Ibf,Rpy =128 Ibf,Rp:=46.6 Ibf,and To =480 Ibf.in.The direction of the output torque T is oppositebecause it is the resistive load on the system opposing the motion. Note in Fig.3-16 the net force from the bearing reactions is zero whereas the net moment about the x axis is 2.25 (192)+2.25(128)=720 lbf.in.This value is the same as Ti+To=240+480 =720 Ibf.in,as shown in Fig.3-la.The reaction forces RE,RF,RH,and RI.from the mounting bolts cannot be determined from the equilibrium equations as there are too many unknowns.Only three equations are available,∑Fy=∑Fz=∑Mx=0.In case you were wondering about assumption 5,here is where we will use it (see Sec.8-12).The gear box tends to rotate about the x axis because of a pure torsional moment of 720 lbf.in.The bolt forces must provideBudynas−Nisbett: Shigley’s Mechanical Engineering Design, Eighth Edition I. Basics 3. Load and Stress Analysis © The McGraw−Hill 75 Companies, 2008 70 Mechanical Engineering Design Summing moments about the x axis of shaft AB in Fig. 3–1d gives Mx = F(0.75) − 240 = 0 F = 320 lbf The normal force is N = 320 tan 20° = 116.5 lbf. Using the equilibrium equations for Figs. 3–1c and d, the reader should verify that: RAy = 192 lbf, RAz = 69.9 lbf, RBy = 128 lbf, RBz = 46.6 lbf, RCy = 192 lbf, RCz = 69.9 lbf, RDy = 128 lbf, RDz = 46.6 lbf, and To = 480 lbf · in. The direction of the output torque To is opposite ωo because it is the resistive load on the system opposing the motionωo. Note in Fig. 3–1b the net force from the bearing reactions is zero whereas the net moment about the x axis is 2.25 (192) + 2.25 (128) = 720 lbf · in. This value is the same as Ti + To = 240 + 480 = 720 lbf · in, as shown in Fig. 3–1a. The reaction forces RE , RF , RH , and RI , from the mounting bolts cannot be determined from the equilibrium equations as there are too many unknowns. Only three equations are available, Fy = Fz = Mx = 0. In case you were wondering about assumption 5, here is where we will use it (see Sec. 8–12). The gear box tends to rotate about the x axis because of a pure torsional moment of 720 lbf · in. The bolt forces must provide (a) Gear reducer 5 in 4 in C A I E B D F H G2 G1 0 T0 i , Ti 240 lbfin (c) Input shaft B Ti 240 lbfin G1 r1 RBz RBy 1.5 in 1 in N A F RAz RAy (d) Output shaft D G2 r2 T0 RDz RDy N F C RCz RCy (b) Gear box z y x 5 in 4 in C A I E D F H B RDy RBy RBz RDz RCy RAy RAz RF RH RCz RI RE Figure 3–1 (a) Gear reducer; (b–d) free-body diagrams. Diagrams are not drawn to scale.