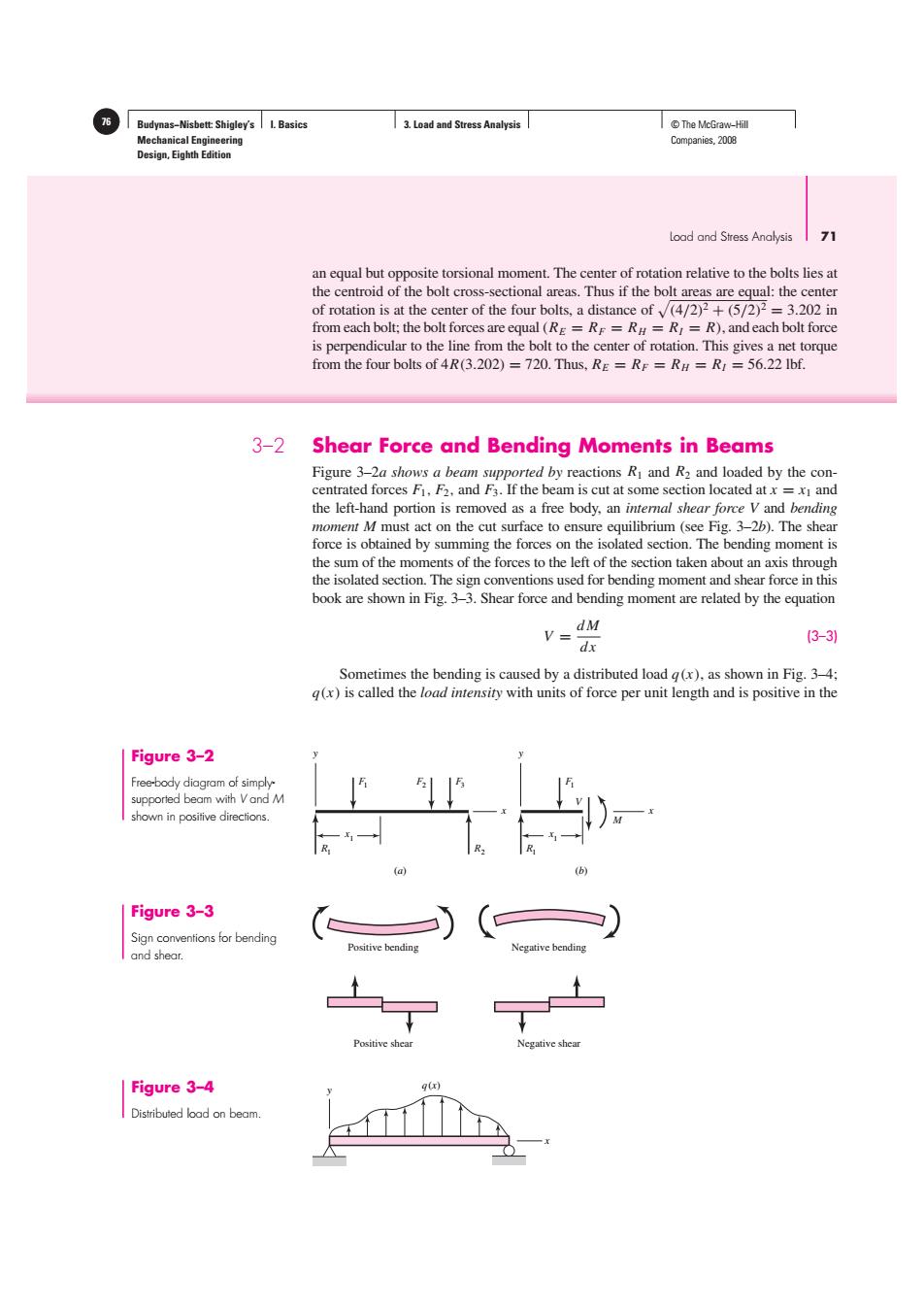
Budynas-Nisbett:Shigley's I.Basics 3.Load and Stress Analysis ©The McGraw-Hil Mechanical Engineering Companies,2008 Design,Eighth Edition Load and Stress Analysis 71 an equal but opposite torsional moment.The center of rotation relative to the bolts lies at the centroid of the bolt cross-sectional areas.Thus if the bolt areas are equal:the center of rotation is at the center of the four bolts,a distance of (4/2)2+(5/2)2=3.202 in from each bolt:the bolt forces are equal (Rg RF=R=RI=R),and each bolt force is perpendicular to the line from the bolt to the center of rotation.This gives a net torque from the four bolts of 4R(3.202)=720.Thus,RE =RF =RH=RI=56.22 Ibf. 3-2 Shear Force and Bending Moments in Beams Figure 3-2a shows a beam supported by reactions R and R2 and loaded by the con- centrated forces F1,F2,and F3.If the beam is cut at some section located at x =x and the left-hand portion is removed as a free body,an internal shear force V and bending moment M must act on the cut surface to ensure equilibrium (see Fig.3-2b).The shear force is obtained by summing the forces on the isolated section.The bending moment is the sum of the moments of the forces to the left of the section taken about an axis through the isolated section.The sign conventions used for bending moment and shear force in this book are shown in Fig.3-3.Shear force and bending moment are related by the equation V=d dx (3-3) Sometimes the bending is caused by a distributed load g(x).as shown in Fig.3-4; g(x)is called the load intensity with units of force per unit length and is positive in the Figure 3-2 Free-body diagram of simply supported beam with Vand M shown in positive directions. Figure 3-3 Sign conventions for bending and shear. Negative s Figure 3-4 q(x) Distributed load on beam.Budynas−Nisbett: Shigley’s Mechanical Engineering Design, Eighth Edition I. Basics 3. Load and Stress Analysis 76 © The McGraw−Hill Companies, 2008 Load and Stress Analysis 71 an equal but opposite torsional moment. The center of rotation relative to the bolts lies at the centroid of the bolt cross-sectional areas. Thus if the bolt areas are equal: the center of rotation is at the center of the four bolts, a distance of  (4/2)2 + (5/2)2 = 3.202 in from each bolt; the bolt forces are equal(RE = RF = RH = RI = R), and each bolt force is perpendicular to the line from the bolt to the center of rotation. This gives a net torque from the four bolts of 4R(3.202) = 720. Thus, RE = RF = RH = RI = 56.22 lbf. 3–2 Shear Force and Bending Moments in Beams Figure 3–2a shows a beam supported by reactions R1 and R2 and loaded by the con￾centrated forces F1, F2, and F3. If the beam is cut at some section located at x = x1 and the left-hand portion is removed as a free body, an internal shear force V and bending moment M must act on the cut surface to ensure equilibrium (see Fig. 3–2b). The shear force is obtained by summing the forces on the isolated section. The bending moment is the sum of the moments of the forces to the left of the section taken about an axis through the isolated section. The sign conventions used for bending moment and shear force in this book are shown in Fig. 3–3. Shear force and bending moment are related by the equation V = d M dx (3–3) Sometimes the bending is caused by a distributed load q(x), as shown in Fig. 3–4; q(x) is called the load intensity with units of force per unit length and is positive in the Figure 3–2 Free-body diagram of simply￾supported beam with V and M shown in positive directions. Figure 3–3 Sign conventions for bending and shear. Figure 3–4 Distributed load on beam. Positive bending Positive shear Negative shear Negative bending x y q(x) x1 x1 y y F1 F2 F3 F1 x x R1 R2 R1 V M (a) (b)