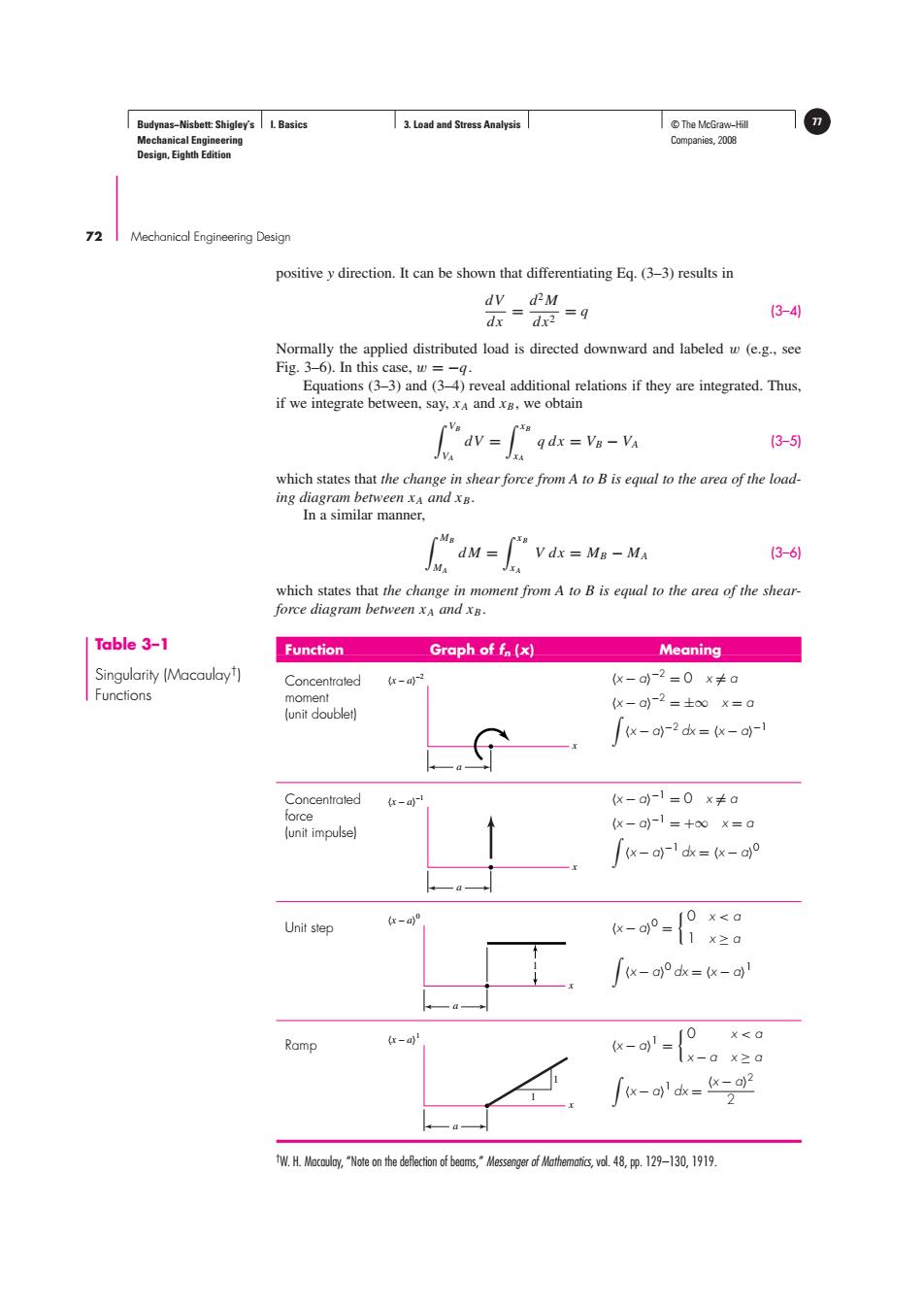
Budynas-Nisbett:Shigley's I.Basics 3.Load and Stress Analysis T©The McGraw-Hill Mechanical Engineering Companies,2008 Design,Eighth Edition 72 I Mechanical Engineering Design positive y direction.It can be shown that differentiating Eq.(3-3)results in dv dM dxdx=q (3-4 Normally the applied distributed load is directed downward and labeled w(e.g.,see Fig.3-6).In this case,w=-g. Equations(3-3)and (3-4)reveal additional relations if they are integrated.Thus, if we integrate between,say,xA and xg,we obtain XB dv= gdx =VB-VA (3-51 which states that the change in shear force from A to B is equal to the area of the load- ing diagram between xa and xB. In a similar manner, dM= V dx Mg-MA (3-61 which states that the change in moment from A to B is equal to the area of the shear- force diagram between xA and xB. Table 3-1 Function Graph of fn (x) Meaning Singularity (Macaulay) Concentrated (-a) K-a)-2=0x≠a Functions moment x-a)-2=±o0×=a (unit doublet) /(x-a)-2dk=(x-o)-1 Concentrated ix-a) K-a-1=0x≠a force x-a-1=+∞X=a (unit impulse) (x-a)-1dx=(x-ao Unit step (x-ajo x-a°= 10X<a 11x≥a x-o°dk=x-al (-o)= 0 Ramp X<a x-ax≥a /x-o!d=-0)2 2 tW.H.Mocoy,"Noten the deflection of beams,"Messenger ofMathematis,vol.48pp.129-30,1919Budynas−Nisbett: Shigley’s Mechanical Engineering Design, Eighth Edition I. Basics 3. Load and Stress Analysis © The McGraw−Hill 77 Companies, 2008 72 Mechanical Engineering Design positive y direction. It can be shown that differentiating Eq. (3–3) results in dV dx = d2M dx2 = q (3–4) Normally the applied distributed load is directed downward and labeled w (e.g., see Fig. 3–6). In this case, w = −q. Equations (3–3) and (3–4) reveal additional relations if they are integrated. Thus, if we integrate between, say, xA and xB, we obtain  VB VA dV =  xB xA q dx = VB − VA (3–5) which states that the change in shear force from A to B is equal to the area of the load￾ing diagram between xA and xB. In a similar manner,  MB MA d M =  xB xA V dx = MB − MA (3–6) which states that the change in moment from A to B is equal to the area of the shear￾force diagram between xA and xB. Function Graph of fn (x) Meaning x − a −2 = 0 x = a x − a −2 = ±∞ x = a  x − a −2 dx = x − a −1 Concentrated x − a −1 = 0 x = a force x − a −1 = +∞ x = a (unit impulse)  x − a −1 dx = x − a 0 Unit step x − a 0 =  0 x < a 1 x ≥ a  x − a 0 dx = x − a 1 Ramp x − a 1 =  0 x < a x − a x ≥ a  x − a 1 dx = x − a2 2 † W. H. Macaulay, “Note on the deflection of beams,” Messenger of Mathematics, vol. 48, pp. 129–130, 1919. Concentrated moment (unit doublet) x x – a –2 a x x – a –1 a x x – a 0 a 1 x x – a 1 a 1 1 Table 3–1 Singularity (Macaulay†) Functions