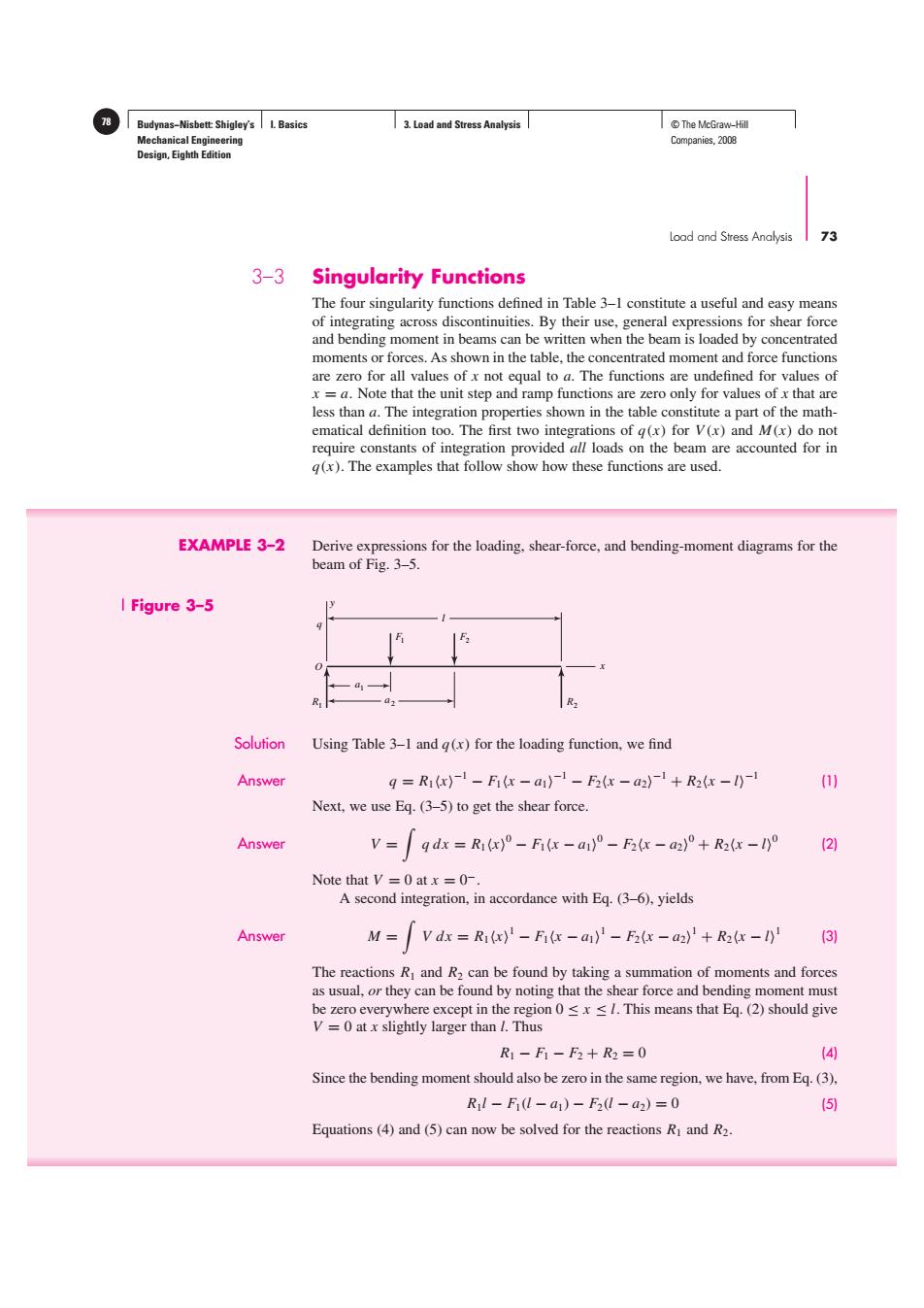
Budynas-Nisbett:Shigley's I.Basics 3.Load and Stress Analysis T©The McGraw-Hill Mechanical Engineering Companies,2008 Design,Eighth Edition Load and Stress Analysis 73 3-3 Singularity Functions The four singularity functions defined in Table 3-1 constitute a useful and easy means of integrating across discontinuities.By their use,general expressions for shear force and bending moment in beams can be written when the beam is loaded by concentrated moments or forces.As shown in the table,the concentrated moment and force functions are zero for all values ofx not equal to a.The functions are undefined for values of x=a.Note that the unit step and ramp functions are zero only for values of x that are less than a.The integration properties shown in the table constitute a part of the math- ematical definition too.The first two integrations of g(x)for V(x)and M(x)do not require constants of integration provided all loads on the beam are accounted for in (x).The examples that follow show how these functions are used. EXAMPLE 3-2 Derive expressions for the loading,shear-force,and bending-moment diagrams for the beam of Fig.3-5. I Figure 3-5 Solution Using Table 3-1 and g(x)for the loading function,we find Answer q=R1x)-1-Fc-a)-1-F2x-a2)-1+R2x-0-1 (1) Next,we use Eq.(3-5)to get the shear force. Answer V=qdx=R°-F-am°-Fx-am°+R2x-° 2 Note that V =0 atx =0- A second integration,in accordance with Eq.(3-6),yields Answer M=V dx=Ri(x)-Fi(x-ai)-F2(x -az)+Ra(x-1) 3) The reactions R and R2 can be found by taking a summation of moments and forces as usual,or they can be found by noting that the shear force and bending moment must be zero everywhere except in the region 0sxs1.This means that Eq.(2)should give V=0 at x slightly larger than l.Thus R1-F-F2+R2=0 (4④ Since the bending moment should also be zero in the same region,we have,from Eq.(3), Rl-F1l-a1)-F20-a2)=0 5) Equations(4)and(5)can now be solved for the reactions Ri and R2.Budynas−Nisbett: Shigley’s Mechanical Engineering Design, Eighth Edition I. Basics 3. Load and Stress Analysis 78 © The McGraw−Hill Companies, 2008 Load and Stress Analysis 73 3–3 Singularity Functions The four singularity functions defined in Table 3–1 constitute a useful and easy means of integrating across discontinuities. By their use, general expressions for shear force and bending moment in beams can be written when the beam is loaded by concentrated moments or forces. As shown in the table, the concentrated moment and force functions are zero for all values of x not equal to a. The functions are undefined for values of x = a. Note that the unit step and ramp functions are zero only for values of x that are less than a. The integration properties shown in the table constitute a part of the math￾ematical definition too. The first two integrations of q(x) for V(x) and M(x) do not require constants of integration provided all loads on the beam are accounted for in q(x). The examples that follow show how these functions are used. EXAMPLE 3–2 Derive expressions for the loading, shear-force, and bending-moment diagrams for the beam of Fig. 3–5. a1 a2 l F1 F2 R1 R2 x y q O Solution Using Table 3–1 and q(x) for the loading function, we find Answer q = R1x −1 − F1x − a1 −1 − F2x − a2 −1 + R2x − l −1 (1) Next, we use Eq. (3–5) to get the shear force. Answer V =  q dx = R1x 0 − F1x − a1 0 − F2x − a2 0 + R2x − l 0 (2) Note that V = 0 at x = 0−. A second integration, in accordance with Eq. (3–6), yields Answer M =  V dx = R1x 1 − F1x − a1 1 − F2x − a2 1 + R2x − l 1 (3) The reactions R1 and R2 can be found by taking a summation of moments and forces as usual, or they can be found by noting that the shear force and bending moment must be zero everywhere except in the region 0 ≤ x ≤ l. This means that Eq. (2) should give V = 0 at x slightly larger than l. Thus R1 − F1 − F2 + R2 = 0 (4) Since the bending moment should also be zero in the same region, we have, from Eq. (3), R1l − F1(l − a1) − F2(l − a2) = 0 (5) Equations (4) and (5) can now be solved for the reactions R1 and R2. Figure 3–5