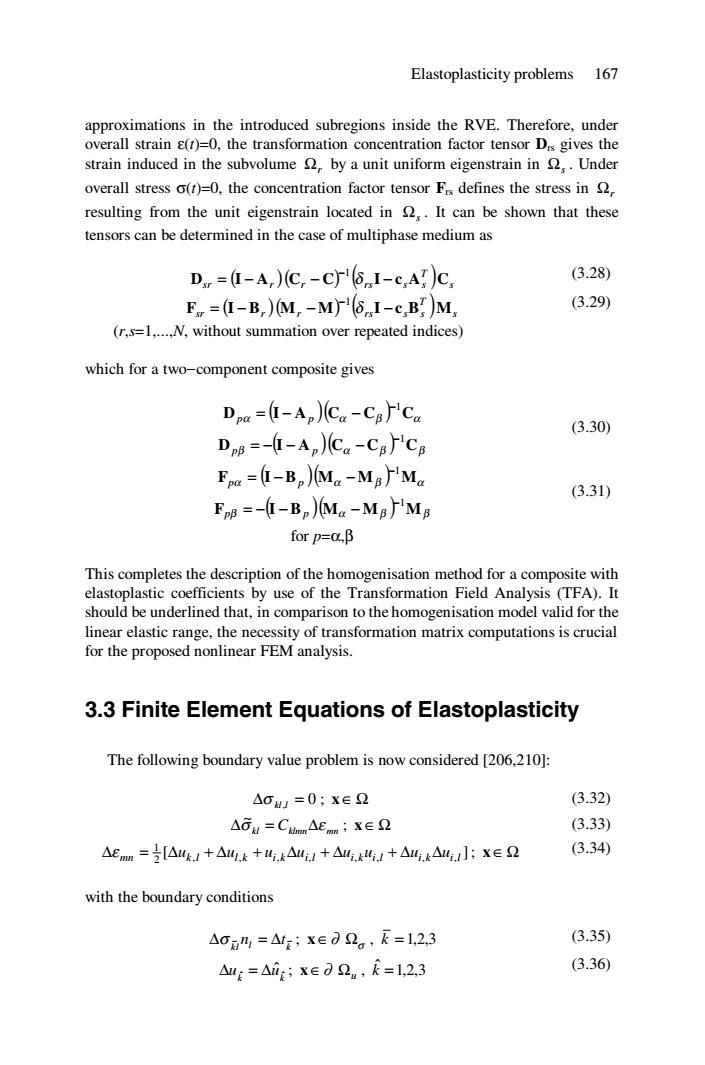
Elastoplasticity problems 167 approximations in the introduced subregions inside the RVE.Therefore,under overall strain e(t)=0,the transformation concentration factor tensor Ds gives the strain induced in the subvolume by a unit uniform eigenstrain in ,Under overall stress o(t)=0,the concentration factor tensor Fs defines the stress in resulting from the unit eigenstrain located in ,It can be shown that these tensors can be determined in the case of multiphase medium as D.r=(I-A,)(C,-C)(6I-e,AT)C, (3.28) Fr =(I-B,)(M,-M)6I-c,B M, (3.29) (r,s=1,...,N,without summation over repeated indices) which for a two-component composite gives Dpa=(I-Ap)(Ca-CB)Ca (3.30) DpB=-(I-Ap)(Ca-Cp}'CB Fpa=(I-Bp)(Ma-MB)Ma (3.31) FpB=-(I-Bp)(Ma-Mg)'Mg forp=oβ This completes the description of the homogenisation method for a composite with elastoplastic coefficients by use of the Transformation Field Analysis (TFA).It should be underlined that,in comparison to the homogenisation model valid for the linear elastic range,the necessity of transformation matrix computations is crucial for the proposed nonlinear FEM analysis. 3.3 Finite Element Equations of Elastoplasticity The following boundary value problem is now considered [206,210]: △oHu=0;x∈2 (3.32) △6u=Cumn AEm;x∈2 (3.33) △Emm=2[△k.1+△.k+,k△1+△.k1+△.k△小；X∈2 (3.34) with the boundary conditions △ca乃=△g;xe02。,k=l,2,3 (3.35) △lk=Ait;xe∂2n,R=l,2,3 (3.36)Elastoplasticity problems 167 approximations in the introduced subregions inside the RVE. Therefore, under overall strain ε(t)=0, the transformation concentration factor tensor Drs gives the strain induced in the subvolume Ωr by a unit uniform eigenstrain in Ωs . Under overall stress σ(t)=0, the concentration factor tensor Frs defines the stress in Ωr resulting from the unit eigenstrain located in Ωs . It can be shown that these tensors can be determined in the case of multiphase medium as ( )( ) ( ) s T Dsr = I − Ar Cr − C rsI − csAs C − δ 1 (3.28) ( )( ) ( ) s T Fsr = I − Br Mr − M rsI − csBs M − δ 1 (r,s=1,...,N, without summation over repeated indices) (3.29) which for a two-component composite gives ( )( ) α A Cα Cβ Cα D I −1 p = − p − ( )( ) β A Cα Cβ Cβ D I −1 p = − − p − (3.30) ( )( ) α B Mα Mβ Mα F I −1 p = − p − ( )( ) β B Mα Mβ Mβ F I −1 p = − − p − for p=α,β (3.31) This completes the description of the homogenisation method for a composite with elastoplastic coefficients by use of the Transformation Field Analysis (TFA). It should be underlined that, in comparison to the homogenisation model valid for the linear elastic range, the necessity of transformation matrix computations is crucial for the proposed nonlinear FEM analysis. 3.3 Finite Element Equations of Elastoplasticity The following boundary value problem is now considered [206,210]: ∆σ kl ,l = 0 ; x ∈Ω (3.32) kl Cklmn mn ∆σ = ∆ε ~ ; x ∈Ω (3.33) [ ] 2 , , , , , , , , 1 ∆ mn = ∆uk l + ∆ul k + ui k∆ui l + ∆ui kui l + ∆ui k∆ui l ε ; x ∈Ω (3.34) with the boundary conditions kl l k ∆σ n = ∆t ; σ x ∈∂ Ω , k = 1,2,3 (3.35) k k u ˆ u ˆ ∆ = ∆ ˆ ; ∈ Ωu x ∂ , 3 1,2, ˆ k = (3.36)