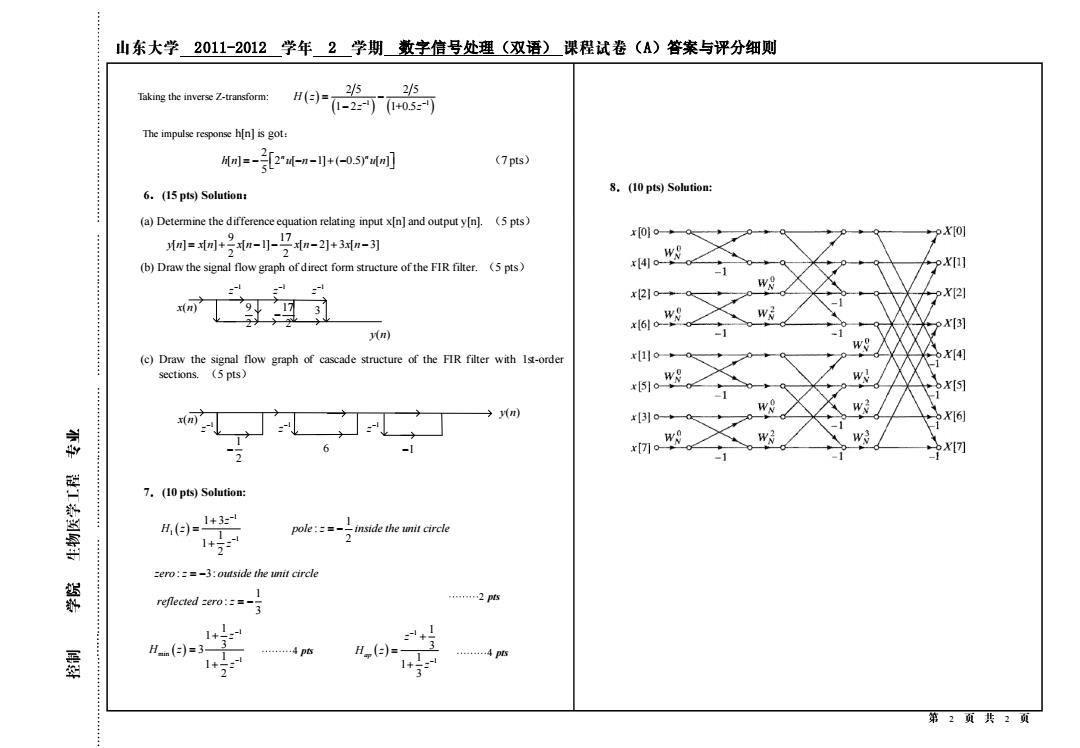
山东大学2011-2012学年2学期数字信号处理（双语）课程试卷()答案与评分细则 2/5 Taking the inverse Z-transform: 25 -2阿405可 The impulse response hn]is got: j--[2"4-n-I+(-05m]] (7pts) 8.(10 pts)Solution: 6.(15 pts)Solution: (a)Determine the difference equation relating input x[n]and outputy[n].(5pts) 网=网+n-明-7 9 x00-+ X[0] n-2]+3n-3列 (b)Draw the signal flow graph of direct form structure of the FIR filter.(5 pts) x[1] x2o→ w 6o9 X3 () w9 (c)Draw the signal flow graph of cascade structure of the FIR filter with Ist-order x[1o X4] sections.(5 pts) x[5] W x(n) X[6] W的 x[7] 2 7.(10 pts)Solution: g 1 pole:==- -inside the unit circle ero:==-3:outside the init circle 器 reflected sero=- …2ps 1+ + Hn(e）=33 H,(e)= 3 …4ps 1+ 第2项共2页2011-2012 2 数字信号处理（双语） （A）答案与评分细则 2 2 Taking the inverse Z-transform: ( ) ( ) ( ) 1 1 2 5 2 5 1 2 1+0.5 H z z z − − = − − The impulse response h[n] is got： 2 [ ] 2 [ 1] ( 0.5) [ ] 5 n n h n u n u n = − − − + −     （7 pts） 6．(15 pts) Solution： (a) Determine the difference equation relating input x[n] and output y[n]. （5 pts） 9 17 [ ] [ ] [ 1] [ 2] 3 [ 3] 2 2 y n x n x n x n x n = + − − − + − (b) Draw the signal flow graph of direct form structure of the FIR filter. （5 pts） x n( ) y n( ) 1 z − 1 z − 9 3 2 17 2 − 1 z − (c) Draw the signal flow graph of cascade structure of the FIR filter with 1st-order sections. （5 pts） x n( ) y n( ) 1 z − 1 z − 6 −1 1 2 − 1 z − 7．(10 pts) Solution: 8．(10 pts) Solution: ( ) 1 1 1 1 3 1 1 2 − − + = + z H z z 1 : 2 pole z inside the unit circle = − zero z outside the unit circle : 3: = − 1 : 3 reflected zero z = − ( ) 1 1 1 3 1 1 3 − − + = + ap z H z z ( ) 1 min 1 1 1 3 3 1 1 2 − − + = + z H z z 2 pts 4 pts 4 pts