
山东大学2011-2012学年2学期数字信号处理(双语)课程试卷(4)答案与评分细则 1.(10pts,2 pts for each)Solution: 1)Compute the 8-point DFT G[k]and ofthe two sequence gin]and n]; 1)B 2)Compute G[]]; 2)A 3)B 3)Compute IDFTof G[]H[]and y.(m)=IDFTG[]] (5pts) 4)A 5)B 4.(15 pts)Solution: 2.(10 pts)Solution: 3x+2 110.51 H,)=2+35+2+*+s+055+1 a)The system is generalized linear-phase system.Since h[4-n]=-hInl,M=4,By the odd (5 pts) symmetry of the sequence as in the figure we know it has the generalized linear Ht)=0.5e05+e phase. (.2亦) Since hn]=he(nT)is required,n=0.Seasr+e The point of symmetry is n=2,so we know the phase of H)is 0.5 (5 pts) ag[H(em】=-2o+π/2人 Then H()严1-eFti-erF (.3pt) When T=0.1s 1.5-(e-aas +0.5eal)= H()= em(5 ps) 0.5 1 Thus 5.(15 pts)Solution: 8w[x(e小-[l】小=-品-2a*2-2 (..2亦 a)System function:H()=7 2/52/5 -2可40.5可-21+052可 b)The system is type III FIR linear-phase system. (3p The poles and zeros of the system function in the z-plane: im 1 zero at Z= 3.(15 pts)Solution: e 怨 a)片(m)={-6,16,0,-19,2,4n=0,12,34,5} (5 pts) (5pts) b)y.(m)={-4,20,0,-19,n=0,12,3} (5pts) b)The ROC and the impulse response h[n]. 墨 e)yu[n]can be computed by using DFT when length N of DFT is not less than 6.The steps Ifthe system is stable,then the ROC includes the unit circle: <H<2.(3ps) are as following: 第1页共2页
2011-2012 2 数字信号处理(双语) (A)答案与评分细则 1 2 1.(10 pts, 2 pts for each) Solution: 1) B 2) A 3) B 4) A 5) B 2.(10 pts) Solution: a) The system is generalized linear-phase system. Since h[4-n]= -h[n], M=4,By the odd symmetry of the sequence as in the figure we know it has the generalized linear phase. The point of symmetry is n=2, so we know the phase of ( ) j H e is arg 2 / 2 ( ) j H e = − + ). Thus , b) The system is type III FIR linear-phase system. 3.(15 pts) Solution: a) y n n L ( ) 6,16,0, 19,2,4, 0,1,2,3,4,5 = − − = (5 pts) b) y n n c ( ) 4,20,0, 19, 0,1,2,3 = − − = (5 pts) c) yL[n] can be computed by using DFT when length N of DFT is not less than 6 . The steps are as following: 1) Compute the 8-point DFT G k[ ] and H k[ ] of the two sequence g n[ ] and hn[ ] ; 2) Compute G k[ ] H k[ ] ; 3) Compute IDFT of G k[ ] H k[ ] and ( ) L y n = IDFT{ G k[ ] H k[ ] }. (5 pts) 4.(15 pts) Solution: 2 3 2 1 1 0.5 1 ( ) 2 3 1 2 1 1 0.5 1 a s H s s s s s s s + = = + = + + + + + + + 0.5 ( ) 0.5 t t h t e e − − = + (5 pts) Since h[n] = hc(nT) is required, 0.5 [ ] 0.5 nT nT h n e e − − = + Then 0.5 1 1 0.5 1 ( ) 1 1 H z T T e z e z − − − − = + − − (5 pts) When T=0.1s 0.05 0.1 1 0.05 1 0.1 1 0.1 0.05 1 0.15 2 0.5 1 1.5 ( 0.5 ) ( ) 1 1 1 ( ) e e z H z e z e z e e z e z − − − − − − − − − − − + = + = − − − + + , 0.05 z e − (5 pts) 5.(15 pts) Solution: a) System function: ( ) ( ) ( ) 1 1 2 5 2 5 1 2 1+0.5 H z z z − − = − − ( )( ) 1 1 1 1 2 1+0.5 z z z − − − = − The poles and zeros of the system function in the z-plane: (5 pts) b) The ROC and the impulse response h[n]. If the system is stable, then the ROC includes the unit circle: 1 2 2 z 。(3 pts) ( ……3 pts ) ( ) (arg 2 / 2 2 ( ) ) ( ) jw d d j grd X e H e d d = − = − − + = ( ……2 pts ) ( ……2 pts ) ( ……3pts )
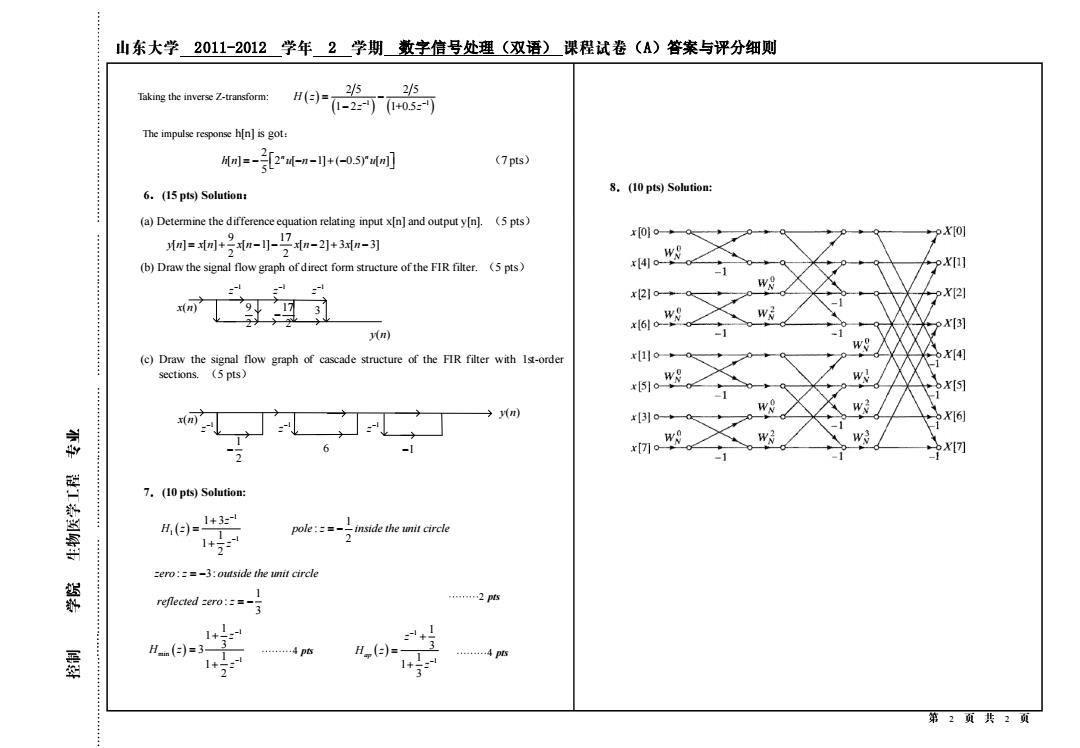
山东大学2011-2012学年2学期数字信号处理(双语)课程试卷()答案与评分细则 2/5 Taking the inverse Z-transform: 25 -2阿405可 The impulse response hn]is got: j--[2"4-n-I+(-05m]] (7pts) 8.(10 pts)Solution: 6.(15 pts)Solution: (a)Determine the difference equation relating input x[n]and outputy[n].(5pts) 网=网+n-明-7 9 x00-+ X[0] n-2]+3n-3列 (b)Draw the signal flow graph of direct form structure of the FIR filter.(5 pts) x[1] x2o→ w 6o9 X3 () w9 (c)Draw the signal flow graph of cascade structure of the FIR filter with Ist-order x[1o X4] sections.(5 pts) x[5] W x(n) X[6] W的 x[7] 2 7.(10 pts)Solution: g 1 pole:==- -inside the unit circle ero:==-3:outside the init circle 器 reflected sero=- …2ps 1+ + Hn(e)=33 H,(e)= 3 …4ps 1+ 第2项共2页
2011-2012 2 数字信号处理(双语) (A)答案与评分细则 2 2 Taking the inverse Z-transform: ( ) ( ) ( ) 1 1 2 5 2 5 1 2 1+0.5 H z z z − − = − − The impulse response h[n] is got: 2 [ ] 2 [ 1] ( 0.5) [ ] 5 n n h n u n u n = − − − + − (7 pts) 6.(15 pts) Solution: (a) Determine the difference equation relating input x[n] and output y[n]. (5 pts) 9 17 [ ] [ ] [ 1] [ 2] 3 [ 3] 2 2 y n x n x n x n x n = + − − − + − (b) Draw the signal flow graph of direct form structure of the FIR filter. (5 pts) x n( ) y n( ) 1 z − 1 z − 9 3 2 17 2 − 1 z − (c) Draw the signal flow graph of cascade structure of the FIR filter with 1st-order sections. (5 pts) x n( ) y n( ) 1 z − 1 z − 6 −1 1 2 − 1 z − 7.(10 pts) Solution: 8.(10 pts) Solution: ( ) 1 1 1 1 3 1 1 2 − − + = + z H z z 1 : 2 pole z inside the unit circle = − zero z outside the unit circle : 3: = − 1 : 3 reflected zero z = − ( ) 1 1 1 3 1 1 3 − − + = + ap z H z z ( ) 1 min 1 1 1 3 3 1 1 2 − − + = + z H z z 2 pts 4 pts 4 pts