山东大学 2013-2014学年2学期数字信号处理(双语)课程试卷(A) 号 三 四 五 七 八九 总分 阅卷人 得分 港人 2.(40 pts)The system function of a causal LTI system is 得分 6(4:1-1(-5) H(e)= Directions图 (e-2e-3) 好 1)The answers of this test should be in English. (a)(5 pts)Determine the poles and zeros of the system,is it a minimum-phase system? (b)(5 pts)Determine the difference equation relating input x[n]and output y[nl. 2)The full mark of this test is 100.The final course mark is based on this test (70%)and class (c)(5 pts)Draw the 2nd-order direct form II signal flow graph for the system function. record mark (30%). (d)(5 pts)Draw the signal flow graph of parallel-form structure for the system function. 3)Tables of properties of Discrete-time Fourier transform,z-transform and DFT are supplied to (e)(5 pts)Determine the ROC.Is the system stable?Why? you on the last page. (f)(7 pts)Determine the impulse response hfn]. 4)Unless otherwise indicated,answers must be derived or explained,not just simply written down. (g)(8 pts)Determine expressions for a minimum-phase system H()and an all-pass 得分 卷人 system H()such that H(z)=H()H() (10 pts,2 pts for each)Choose the best answer to fill in the blanks. 的 1)For a system for which the input and output satisfy a linear constant-coefficient difference equation,if the auxiliary information is in the form of N sequential values of the output, then the system A is LTI but noncausal system,; B.is LTI and causal system;; C.may not be LTI; D.is causal. 2)If all the three real poles (a,b,c)of a system function H(z)satisfy the condition: 00.D.right-sided sequence,and h[n]=0,for n<0; 说 3)The minimum-phase system is( A.stable and causal; B.stable but not causal; C.causal but not stable. D.neither stable nor causal 4)Consider an L-point sequencex[n]and a P-point sequencexn],for the circular convolutionx[n]x,In]and linear convolutionxInx[n]to be identical,the circular 器 convolution must have a length N of at least )points. A.L+P+1, B.L+P-I; C.L+P: D.L: 5)A Type III FIR Linear-Phase System can be used as a( A.low-pass filter, B.Band-stop filter; 暴 C.high-pass filter, D.Band-pass filter: 第1负共4页
2013-2014 2 数字信号处理(双语) (A) 1 4 题号 一 二 三 四 五 六 七 八 九 十 总分 阅卷人 得分 Directions: 1) The answers of this test should be in English. 2) The full mark of this test is 100. The final course mark is based on this test (70%) and class record mark (30%). 3) Tables of properties of Discrete-time Fourier transform, z-transform and DFT are supplied to you on the last page. 4) Unless otherwise indicated, answers must be derived or explained, not just simply written down. 1.(10 pts, 2 pts for each) Choose the best answer to fill in the blanks. 1) For a system for which the input and output satisfy a linear constant-coefficient difference equation, if the auxiliary information is in the form of N sequential values of the output, then the system ( ). A is LTI but noncausal system;; B. is LTI and causal system;; C. may not be LTI; D. is causal. 2) If all the three real poles (a, b, c) of a system function H (z) satisfy the condition: 00; D. right-sided sequence, and h[n]=0, for n<0; 3) The minimum-phase system is ( ). A. stable and causal; B. stable but not causal; C. causal but not stable; D. neither stable nor causal 4) Consider an L-point sequence 1 x n[ ] and a P-point sequence 2 x n[ ] , for the circular convolution 1 x n[ ] ○N 2 x n[ ] and linear convolution 1 2 x n x n [ ]* [ ] to be identical, the circular convolution must have a length N of at least ( ) points. A. L+P+1; B. L+P-1; C. L+P; D. L; 5) A Type III FIR Linear-Phase System can be used as a ( ). A. low-pass filter; B. Band-stop filter; C. high-pass filter; D. Band-pass filter; 2.(40 pts) The system function of a causal LTI system is ( ) ( )( ) ( )( ) 1 1 1 1 6 4 1 5 2 3 z z H z z z − − − − − − = − − . (a)(5 pts)Determine the poles and zeros of the system, is it a minimum-phase system? (b)(5 pts)Determine the difference equation relating input x[n] and output y[n]. (c)(5 pts)Draw the 2nd-order direct form II signal flow graph for the system function. (d)(5 pts)Draw the signal flow graph of parallel-form structure for the system function. (e)(5 pts)Determine the ROC. Is the system stable? Why? (f)(7 pts)Determine the impulse response h[n]. (g) (8 pts) Determine expressions for a minimum-phase system H z min ( ) and an all-pass system ( ) H z ap such that ( ) min ( ) ( ) 得分 阅卷人 H z H z H z = ap 得分 阅卷人
山东大学2013-2014学年2学期数字信号处理(双语)课程试卷(A) 滋 家 得分 侧卷人 3.(15 pts)A discrete-time lowpass filter is to be designed by applying the impulse invariance method to a continuous-time Butterworth filter having magnitude-squared function The specifications for the discrete-time system are:0.89125Hs10s H(es0.177830.3nssx Assume that aliasing will not be a problem,and suppose the integer order N and e of the 海分卷人 4.(15 pts)Give proof of the DFS periodic convolution property:i.e. continuous-time Butterworth filter have been determined with Td=1,Le.N=6 =0.7032; Letandbe two periodic sequences,each with period N and 器 and we have determined the six poles (s,=12,3,4,5,6)for system function He(s)in he magniude-fucton(dH.(--i+(ia,产 .with Td=l with DFS coefficients denoted by]and]respectively.If we form the product If Td1,it also can be determined that N=6,T=0.7032,and the six poles are then prove that the periodic sequence with Fourier series s,k=1,2,3,4,5,6.Prove that the discrete-time filter system function H (z)which results from impulse invariance design with Td is the same as the result for Td=1. coefreients-2o-叫 第2页共4页
2013-2014 2 数字信号处理(双语) (A) 2 4 3.(15 pts) A discrete-time lowpass filter is to be designed by applying the impulse invariance method to a continuous-time Butterworth filter having magnitude-squared function ( ) ( ) 2 2 1 1 c N c H j j j = + . The specifications for the discrete-time system are:0.89125 1, 0 0.2 ( ) j H e ( ) 0.17783, 0.3 j H e . Assume that aliasing will not be a problem, and suppose the integer order N and c of the continuous-time Butterworth filter have been determined with Td=1, i.e. N=6 c =0.7032; and we have determined the six poles ( , 1,2,3,4,5,6) k s k = for system function H s c ( ) in the magnitude-squared function ( ) ( ) ( ) 2 1 1 c c N c H s H s s j − = + with Td=1. If Td≠1, it also can be determined that N=6, cTd =0.7032, and the six poles are , 1,2,3,4,5,6 k d s T k = . Prove that the discrete-time filter system function H (z) which results from impulse invariance design with Td≠1 is the same as the result for Td=1. 4.(15 pts) Give proof of the DFS periodic convolution property: i.e. Let x n 1 and x n 2 be two periodic sequences, each with period N and with DFS coefficients denoted by X k 1 and X k 2 respectively. If we form the product X k X k X k 3 1 2 = ,then prove that the periodic sequence x n 3 with Fourier series coefficients X k 3 is 1 3 1 2 0 N m x n x m x n m − = = − . 得分 阅卷人 得分 阅卷人

山东大学2013-2014学年2学期数字信号处理(双语)课程试卷() 得分侧卷人 6.(10 pts)The figure below shows the flow graph for an 8-point decimation-in-time FFT algorithm.Let x[n]be the sequence whose DFT is x[k].In the flow graph,A[],B[].C[],and D[]represent separate arrays that are indexed consecutively in the same order as the indicated nodes.Determine and sketch the sequence C[r],,1,.7,if the output Fourier transform is X[k]=1,k=0,1,...7. B间 A PD间 82]w CR] A29+ 4。g 制w质 C 都 A4。+ C刊w8 啊。 C问wW D5例 得分 卷人 5.(10pts)Two 8-point sequences xi[n]and x2[n]shown in the following figures have 8-point DFTs Yilk]and Xlk],respectively. A 句W哭 C16的w D间 Determine the relationship between Xi[k]and X[k]. 对刀w C刀w x(n] D 01月456司 器 聂 第3项共4页
2013-2014 2 数字信号处理(双语) (A) 3 4 5.(10pts) Two 8-point sequences x1[n] and x2[n] shown in the following figures have 8-point DFTs X1[k] and X2[k], respectively. Determine the relationship between X1[k] and X2[k]. 6.(10 pts) The figure below shows the flow graph for an 8-point decimation-in-time FFT algorithm. Let x[n] be the sequence whose DFT is X[k]. In the flow graph, A[·], B[·], C[.], and D[·] represent separate arrays that are indexed consecutively in the same order as the indicated nodes. Determine and sketch the sequence C[r], r=0, 1, ... ,7, if the output Fourier transform is X[k]=1, k=0, 1, .. ,7. 得分 阅卷人 得分 阅卷人
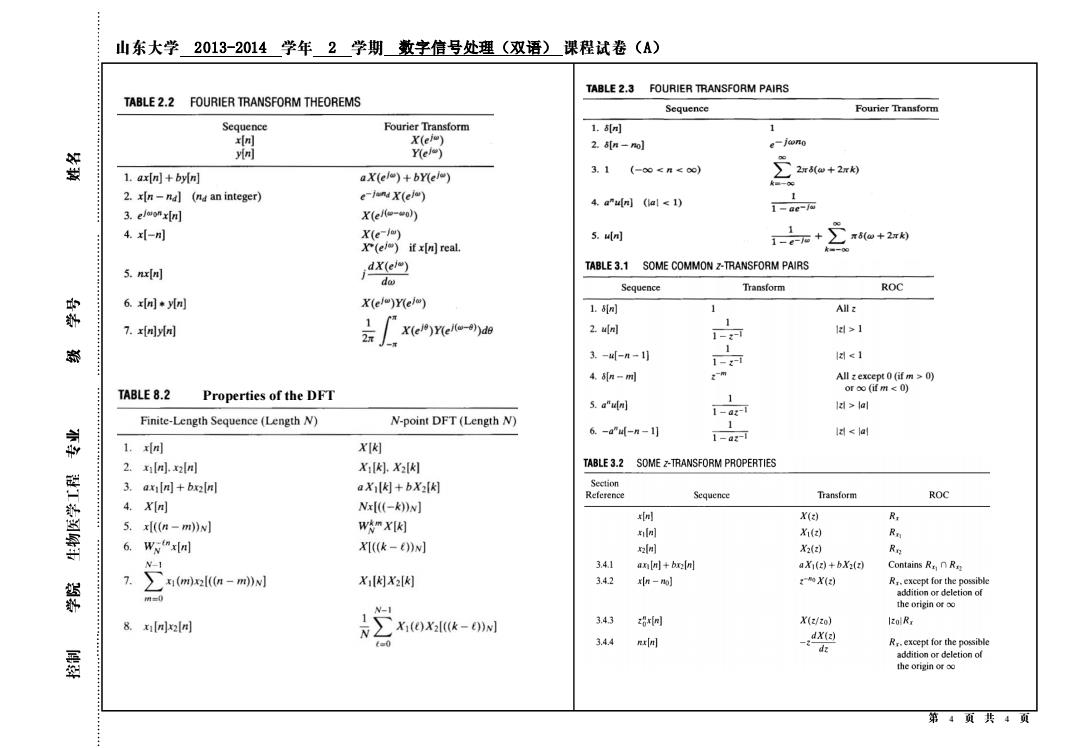
山东大学2013-2014学年2学期数字信号处理(双语)课程试卷(A) TABLE 2.3 FOURIER TRANSFORM PAIRS TABLE 2.2 FOURIER TRANSFORM THEOREMS Sequence Fourier Transform Sequence Fourier Transform 1. 1 X(ej) 2.m-o】 e-jono 好 y Y(el) 1.ax(n]+byin] ax(el)+bY(el) 3.1(-o0n←0∞) ∑2红e+2的 2.x(n-ndl (n an integer) e-junX(ejo) 4.an(al1 游 3.-d-n- 1-x可 10) TABLE8.2 or oo (if m<0) Properties of the DFT 5.a"uln] 1-a可 la lal Finite-Length Sequence(Length N) N-point DFT (Length N) 6.-ad-n-1] la<lal 1.x(n] XI周 2.x[n].x2ln] X:[).X2[k] TABLE 3.2 SOME z-TRANSFORM PROPERTIES 说 3.ax[n]+bx2in] aX因+bXl附 Section Reference Sequence Transform ROC 4.X Nx[((-k))N] xin] X(2) Rr 5.x[((n-m))N] WmX[内 a X R 6.Wyx[n] X(-)》N X2(z) Rn N- 3.41 网+br aX(z)+bx2(z) Contains R:nR X内X2R 3.42 x[n -no] 刷X() R.except for the possible 器 ∑n(mxI(n-mjN m= addition or deletion of N-1 the origin oroo 8.x[n]x2In] x()x2((k-6)N] 3.43 网 X(z/zo) lzoRr 3.4.4 网 Rr.except for the possible 是 addition or deletion of the origin or oo 第4页共4页
2013-2014 2 数字信号处理(双语) (A) 4 4 Properties of the DFT