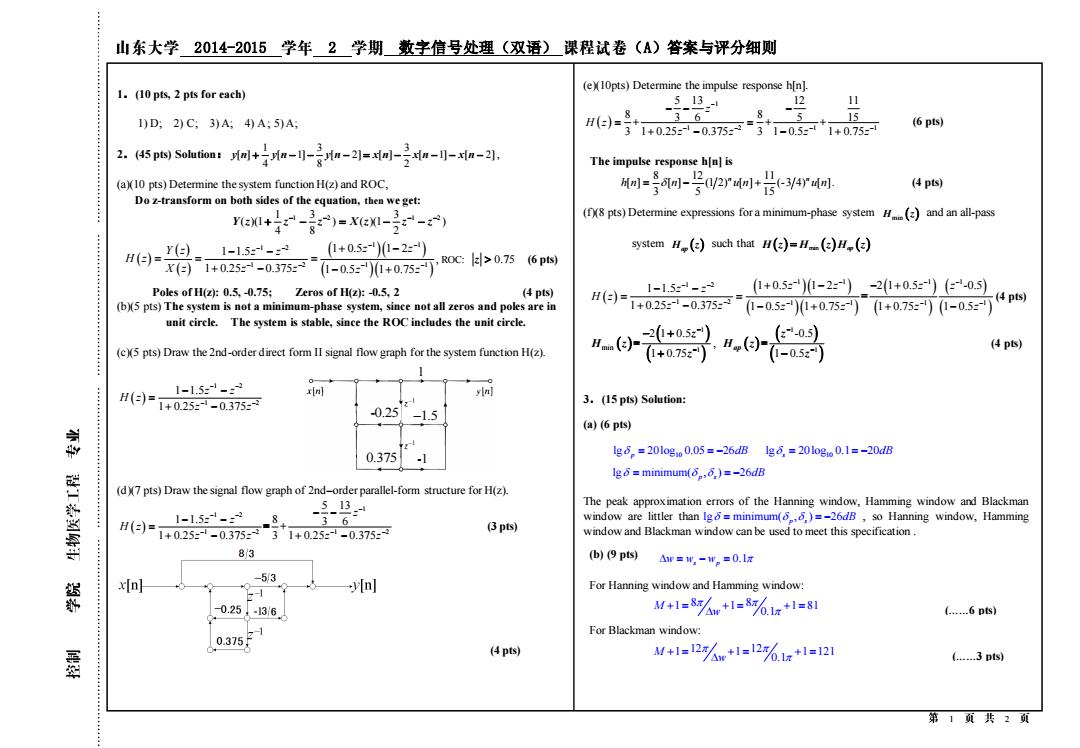
山东大学2014-2015学年2学期数字信号处理(双语)课程试卷()答案与评分细则 1.(10 pts,2 pts for each) (eX1Opts)Determine the impulse response h[n]. 513- _2 山 8 1)D;2)C;3)A,4)A,5)A, 361 5 31+0.25--0.375--31-0.5-1+0.75- (6 pts) 2.asp购so咖ioa:fa+好a-川-2a-2=a-子a-小-a-2. The impulse response hn]is (a)(10 pts)Determine the system function H(z)and ROC, ia-m-号o2r4a 4. 11 (4 pts) Do z-transform on both sides of the equation,then we get: e+-=e0-- (f8 pts)Determine expressions fora minimum-phase system H()and an all-pass H=y但.、1-15- 0+0.52-)0-2-) systemH()such that ()=H()H() X(日1+025z-0.37527 -05-0+0.75-可R0c>0.756ps Poles of H(z):0.5,-0.75;Zeros of H(z):-0.5,2 (4ps) 1-1.52-2 +050-21.-20+05e)04p (b)(5 pts)The system is not a minimum-phase system,since not all zeros and poles are in H)=1+0.25:-0.375 0-0.52)1+0.75-1+0.752-)0-0.52-) unit cirele.The system is stable,since the ROC includes the unit cirele. 0付离 (-0.5) (4ps) (c)(5 pts)Draw the 2nd-order direct form II signal flow graph for the system function H(z). 1-1.52--2 H(日)=1+0.25-0.3752 x(n] yin] 3.(15 pts)Solution: 0.25 -1.5 (a)(6 pts) 0.375 g6,=201ogo0.05=-26 dB 1g6,=200ge0.1=-20dB 的 lg6=minimum(6.,6.)=-26dB (d7 pts)Draw the signal flow graph of 2nd-order parallel-form structure for H(z). 部 The peak approximation errors of the Hanning window,Hamming window and Blackman 1-1.5-2 H(e)=1+0.25-0.375 =8+ 361 window are littler than Ig=minimum(,6,)=-26dB,so Hanning window,Hamming 31+0.25--0.375- (3 pts) window and Blackman window can be used to meet this specification. 83 (b)(9 pts) △1w=1w-1w。=0.1z -53 强 x[n] For Hanning window and Hamming window: 0.25-136. M+1=8%w+1=8%r+1=81 (......6 pts) For Blackman window: 0.375 (4 pts) 61x+1=121 M+1=12x人+1=12x人 (..3pts) 第1页共2页
2014-2015 2 数字信号处理(双语) (A)答案与评分细则 1 2 1.(10 pts, 2 pts for each) 1) D; 2) C; 3) A; 4) A ; 5) A; 2.(45 pts) Solution: 1 3 3 [ ] [ 1] [ 2] [ ] [ 1] [ 2] 4 8 2 y n y n y n x n x n x n + − − − = − − − − , (a)(10 pts) Determine the system function H(z) and ROC, Do z-transform on both sides of the equation, then we get: 1 3 3 1 2 1 2 ( )(1 ) ( )(1 ) 4 8 2 Y z z z X z z z − − − − + − = − − ( ) ( ) ( ) ( )( ) ( )( ) 1 1 1 2 1 2 1 1 1 1.5 1 0.5 1 2 1 0.25 0.375 1 0.5 1 0.75 Y z z z z z H z X z z z z z − − − − − − − − − − + − = = = + − − + , ROC: z 0.75 (6 pts) Poles of H(z): 0.5, -0.75; Zeros of H(z): -0.5, 2 (4 pts) (b)(5 pts) The system is not a minimum-phase system, since not all zeros and poles are in unit circle. The system is stable, since the ROC includes the unit circle. (c)(5 pts) Draw the 2nd-order direct form II signal flow graph for the system function H(z). ( ) 1 2 1 2 1 1.5 1 0.25 0.375 z z H z z z − − − − − − = + − (d)(7 pts) Draw the signal flow graph of 2nd–order parallel-form structure for H(z). ( ) 1 1 2 1 2 1 2 5 13 1 1.5 8 3 6 = + 1 0.25 0.375 1 0.25 0.375 3 z z z H z z z z z − − − − − − − − − − − = + − + − (3 pts) (4 pts) (e)(10pts) Determine the impulse response h[n]. ( ) 1 1 2 1 1 5 13 12 11 8 8 3 6 5 15 + + + 3 3 1 0.25 0.375 1 0.5 1 0.75 z H z z z z z − − − − − − − − = = + − − + (6 pts) The impulse response h[n] is 8 12 11 [ ] [ ] (1 2) [ ] (-3 4) [ ] 3 5 15 n n h n n u n u n = − + . (4 pts) (f)(8 pts) Determine expressions for a minimum-phase system H z min ( ) and an all-pass system ( ) H z ap such that ( ) min ( ) ( ) H z H z H z = ap ( ) ( )( ) ( )( ) ( ) ( ) ( ) ( ) 1 1 1 1 1 2 1 2 1 1 1 1 1 1.5 1 0.5 1 2 2 1 0.5 -0.5 = 1 0.25 0.375 1 0.5 1 0.75 1 0.75 1 0.5 z z z z z z H z z z z z z z − − − − − − − − − − − − − − + − − + = = + − − + + − (4 pts) ( ) ( ) ( ) 1 min 1 2 1 0.5 = 1 0.75 z H z z − − − + + , ( ) ( ) ( ) 1 1 -0.5 = 1 0.5 ap z H z z − − − (4 pts) 3.(15 pts) Solution: (a) (6 pts) The peak approximation errors of the Hanning window, Hamming window and Blackman window are littler than , so Hanning window, Hamming window and Blackman window can be used to meet this specification . (b) (9 pts) For Hanning window and Hamming window: For Blackman window: 1 0.375 −1.5 -1 -0.25 (……6 pts) 10 lg 20log 0.1 20 s lg 20log 0.05 26 p = = − 10 dB = = − dB 0.1 = − = w w w s p lg minimum( , ) 26 p s = = − dB lg minimum( , ) 26 p s = = − dB 8 8 1 1 1 81 0.1 M w + = + = + = 12 12 1 1 1 121 0.1 M w + = + = + = (……3 pts)
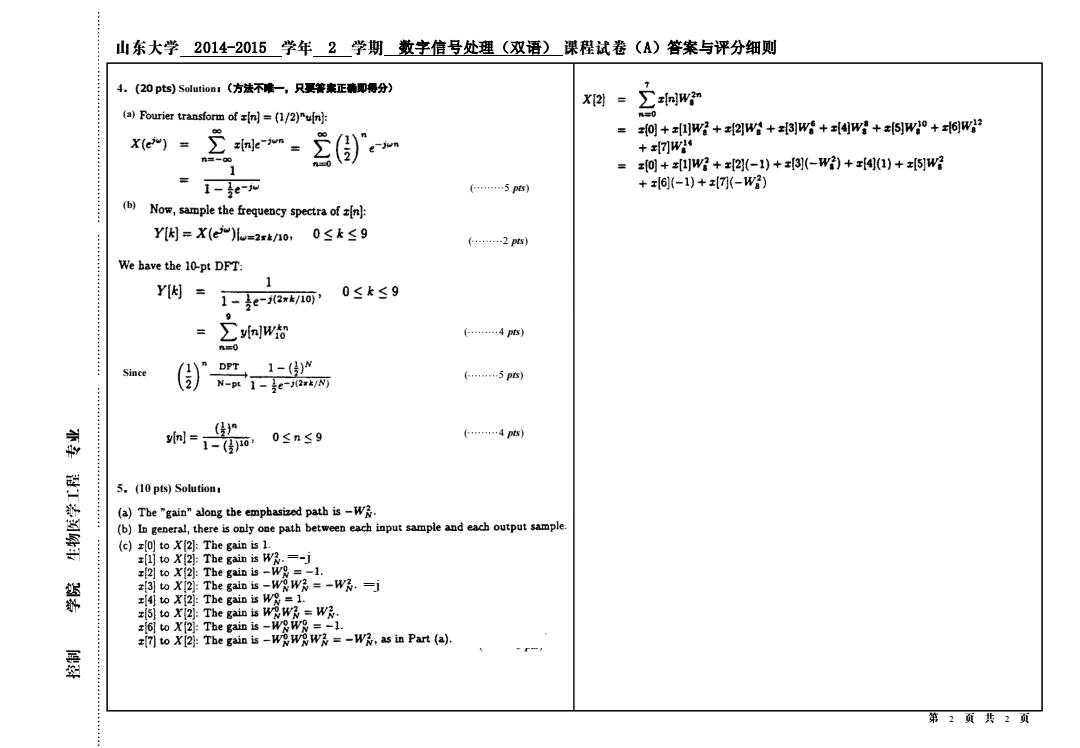
山东大学2014-2015学年2学期数字信号处理(双语) 课程试卷(A)答案与评分细则 4. (20pts)Solution:(方法不唯一,只要答案正确即得分) X12 ∑nwm (a)Fourier transform of n]=(1/2)"un): n= 0+w+w塔+3w赠+wW+5w+6w X(e)= E[nje-jn 三 +=7wi x0+wg+2-1)+3(-w)+(1)+z5w 1-e-加 …5p本) +6(-1)+f可(-w) (b) Now,sample the frequency spectra of n): Y因=X(e0)儿w=2xtno,0≤k≤9 (-2ps) We have the 10-pt DFT: Y( 1-c-2mk/1可 0≤k≤9 9 = ∑nw给 (…4ps) n= n Since 1N DPT 1-()N N-pt 1-se-j(aE/N) …5p) yin]= (严 1-(线)0 0≤n≤9 (…4p) 5. (I0pts)Solution▣ (a)The "gain"along the emphasized path is-W. (b)In general,there is only one path between each input sample and each output sample. (c)[0]to2]:The gain is 1. [1]toX(2):The gain is w2.=-j z2]to X2):The gain is -=-1. 器 z[3]to X2):The gain is-W&W=-W.=j toX2):The gain is W&=1. 5]to x2):The gain is WW=W. 6l to):The gain is -=-1 z[7]toX2):The gain is -W&WRWA=-WR,as in Part (a). 墨 第2项共2页
2014-2015 2 数字信号处理(双语) (A)答案与评分细则 2 2 4.(20 pts) Solution:(方法不唯一,只要答案正确即得分) (a) (b) Since 5.(10 pts) Solution: ( 5 ) pts ( 2 ) pts ( 4 ) pts ( 5 ) pts ( 4 ) pts ( 2 ) pts ( 3 ) pts ( 5 ) pts =-j =j