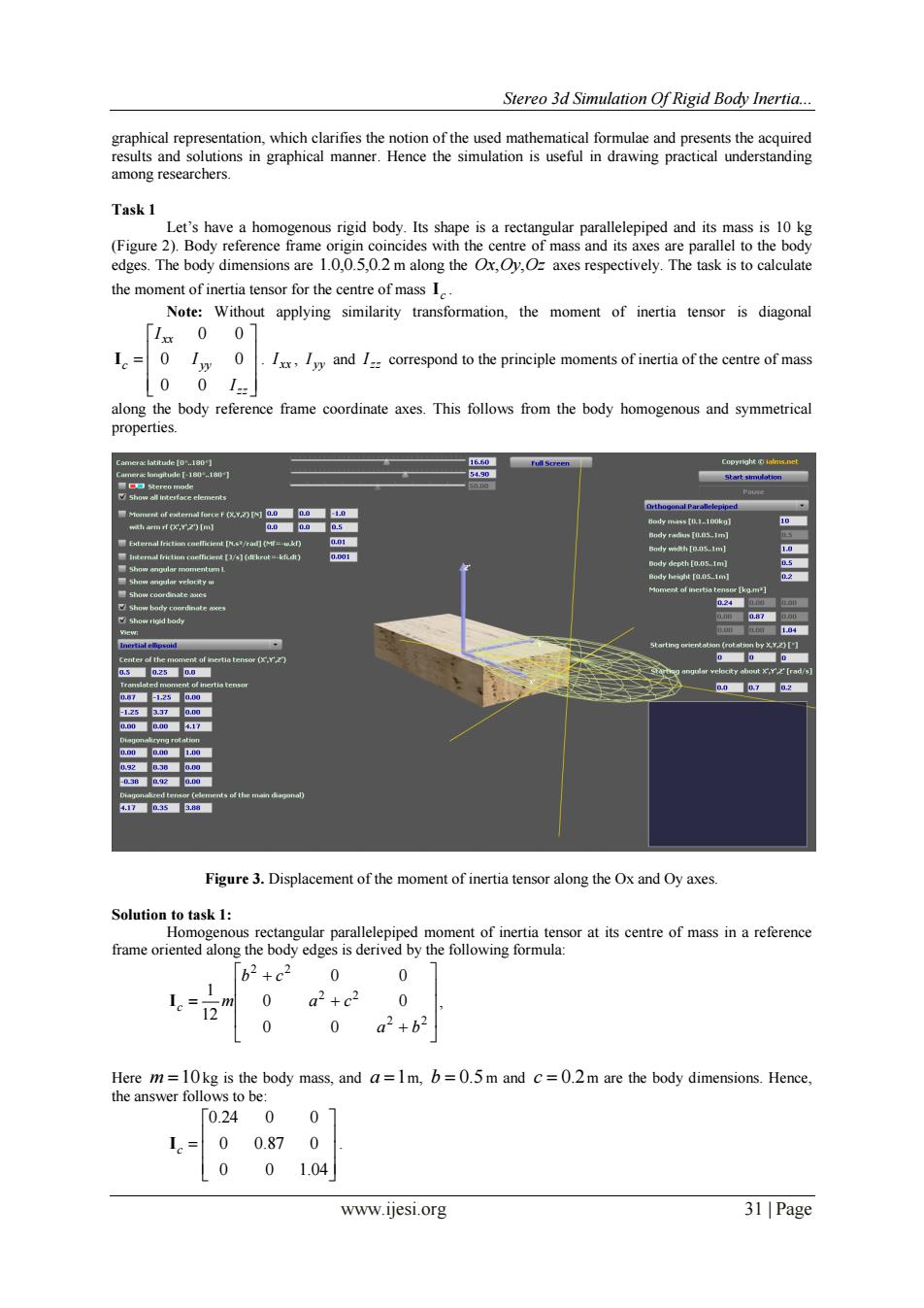
Stereo 3d Simulation Of Rigid Body Inertia... graphical representation,which clarifies the notion of the used mathematical formulae and presents the acquired results and solutions in graphical manner.Hence the simulation is useful in drawing practical understanding among researchers. Task 1 Let's have a homogenous rigid body.Its shape is a rectangular parallelepiped and its mass is 10 kg (Figure 2).Body reference frame origin coincides with the centre of mass and its axes are parallel to the body edges.The body dimensions are 1.0,0.5,0.2 m along the Ox,Ov,O=axes respectively.The task is to calculate the moment of inertia tensor for the centre of mass I. Note:Without applying similarity transformation,the moment of inertia tensor is diagonal [ 0 0 I=0 1 0 and correspond to the principle moments of inertia of the centre of mass 0 along the body reference frame coordinate axes.This follows from the body homogenous and symmetrical properties. 1660 Camerac longitude【-180180】 54.90 start simulation ■口5 tereo mode Show all interfoce elements Orthogonal Parallelepiped wth arm rf (.[m] 10 Sndy radius【.5.,1m] .01■ 10 ■Interal frictinn coeficent D/s】(dEkrot=-k.d) 0.001 Body depth [0.05..Im] 0.5 Body height [o.05.1m] 02 ■5 how coordnate ate Moment of inertia tensor [kg.m2] 0.240 ☑show rio时body 0nm0.700 Wiewt Starting orientatson (rotatinn by X.Ye) Center of the moment of inertia tensor (XY2) 00■0 0.502500 anguiar velocity about[rad/s] of ine 00■07■02 0B7-125000 1z53370.00 0.000006,17 0000001.00■ 092030■0.00 0380920.00 ts of the main daganal) .170353.88■ Figure 3.Displacement of the moment of inertia tensor along the Ox and Oy axes Solution to task 1: Homogenous rectangular parallelepiped moment of inertia tensor at its centre of mass in a reference frame oriented along the body edges is derived by the following formula: b2+c2 0 0 1 Ic= 0 a2+c2 0 0 0 a2+b2 Here m=10kg is the body mass,and a=1m,b=0.5m and c=0.2m are the body dimensions.Hence, the answer follows to be: 「0.24 0 0 1= 0 0.87 0 0 0 1.04 www.ijesi.org 31 |PageStereo 3d Simulation Of Rigid Body Inertia... www.ijesi.org 31 | Page graphical representation, which clarifies the notion of the used mathematical formulae and presents the acquired results and solutions in graphical manner. Hence the simulation is useful in drawing practical understanding among researchers. Task 1 Let’s have a homogenous rigid body. Its shape is a rectangular parallelepiped and its mass is 10 kg (Figure 2). Body reference frame origin coincides with the centre of mass and its axes are parallel to the body edges. The body dimensions are 1.0,0.5,0.2 m along the Ox,Oy,Oz axes respectively. The task is to calculate the moment of inertia tensor for the centre of mass c I . Note: Without applying similarity transformation, the moment of inertia tensor is diagonal            zz yy xx c I I I 0 0 0 0 0 0 I . xx I , yy I and zz I correspond to the principle moments of inertia of the centre of mass along the body reference frame coordinate axes. This follows from the body homogenous and symmetrical properties. Figure 3. Displacement of the moment of inertia tensor along the Ox and Oy axes. Solution to task 1: Homogenous rectangular parallelepiped moment of inertia tensor at its centre of mass in a reference frame oriented along the body edges is derived by the following formula:               2 2 2 2 2 2 0 0 0 0 0 0 12 1 a b a c b c Ic m , Here m 10 kg is the body mass, and a 1 m, b  0.5 m and c  0.2 m are the body dimensions. Hence, the answer follows to be:            0 0 1.04 0 0.87 0 0.24 0 0 c I