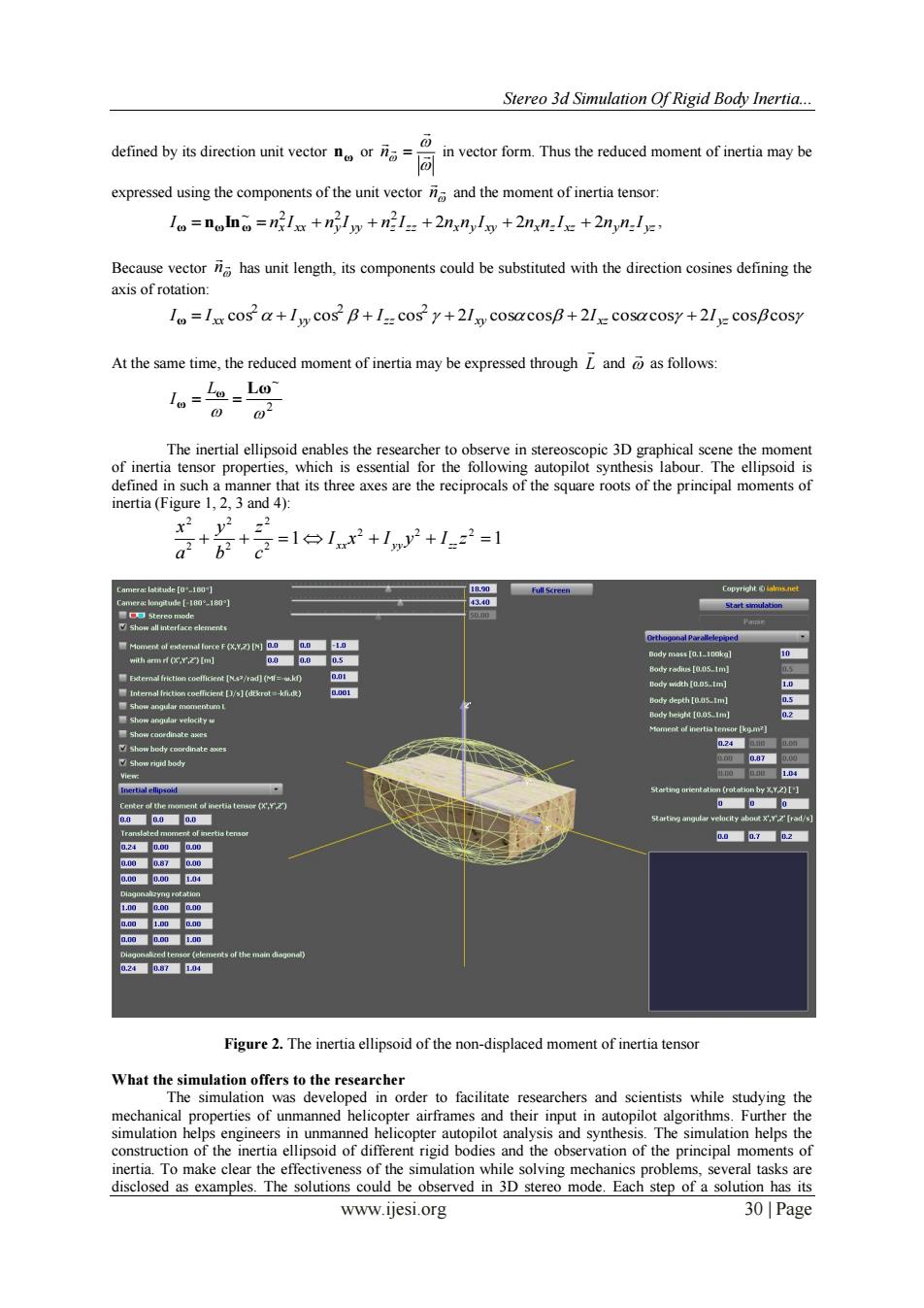
Stereo 3d Simulation Of Rigid Body Inertia... 0 defined by its direction unit vector n or n= in vector form.Thus the reduced moment ofieria may be expressed using the components of the unit vector n and the moment of inertia tensor: Io=nolno=nglxx +nlwy+nI+2nxny lgy +2ngn-le+2nyn-Iy Because vector n has unit length,its components could be substituted with the direction cosines defining the axis of rotation: 1=Ix cos a+lw cos B+I=cosy+21 cosacosB+21cosacosy+21=cosBcosy At the same time,the reduced moment of inertia may be expressed through L and as follows: lo=Lo=Lo- 002 The inertial ellipsoid enables the researcher to observe in stereoscopic 3D graphical scene the moment of inertia tensor properties,which is essential for the following autopilot synthesis labour.The ellipsoid is defined in such a manner that its three axes are the reciprocals of the square roots of the principal moments of inertia(Figure 1,2,3 and 4): y2 22 +存+ Q =1←1xx2+1wy2+1_22=1 Lamera:la成udc[010u】 18.90 Full Screen Copyright ialms.net Camera:longtude [1m1阙】 43.40 start smulation ■O5 tereo mode Panse Show all interface elements Orthogonal parallelepped Body mass [0.1.100kg] with arm rl (x.Y'2)[m] 00■0.0■05 10 0.0t 05 External friction coefficient [Ns7/rad](Mf=kf) Body width [o.05..m] 1.0 Internal friction coefficient [/s](dEkrot-kfi.d) 0.001 Body depth [] 0.5 ■Show angudar velocity Body height [005-Im] 0.2 Moment of inertiatencor [kgmz] MShow body coordinate axes 0.24 0.10 0.0 Show rigid body 000■0870.0 Inertial ellipsoid Starting orientation (rotation by X,Y,2)[] Center of the moment ot inertia tensor 000 00■000.0 Starting angular velocity about [rad/s] Transloted moment of mertid tensor 000.7■02 0.240.00■000■ 0.000.87000 0.000.00104 Diagonalizyng rotation 1.000.00000 0.001.0000■ a.000.001.00 Diagonalized tenso (elements of themain di) 0.24087104 Figure 2.The inertia ellipsoid of the non-displaced moment of inertia tensor What the simulation offers to the researcher The simulation was developed in order to facilitate researchers and scientists while studying the mechanical properties of unmanned helicopter airframes and their input in autopilot algorithms.Further the simulation helps engineers in unmanned helicopter autopilot analysis and synthesis.The simulation helps the construction of the inertia ellipsoid of different rigid bodies and the observation of the principal moments of inertia.To make clear the effectiveness of the simulation while solving mechanics problems,several tasks are disclosed as examples.The solutions could be observed in 3D stereo mode.Each step of a solution has its www.ijesi.org 30|PageStereo 3d Simulation Of Rigid Body Inertia... www.ijesi.org 30 | Page defined by its direction unit vector nω or       n   in vector form. Thus the reduced moment of inertia may be expressed using the components of the unit vector    n and the moment of inertia tensor: x xx y yy z zz x y xy x z xz y z yz I n I n I n I 2n n I 2n n I 2n n I ~ 2 2 2 ω  nωInω       , Because vector    n has unit length, its components could be substituted with the direction cosines defining the axis of rotation: cos  cos  cos  2 coscos 2 coscos 2 cos cos 2 2 2 xx yy z z xy xz yz Iω  I  I  I  I  I  I At the same time, the reduced moment of inertia may be expressed through L  and   as follows: 2 ~   ω Lω ω   L I The inertial ellipsoid enables the researcher to observe in stereoscopic 3D graphical scene the moment of inertia tensor properties, which is essential for the following autopilot synthesis labour. The ellipsoid is defined in such a manner that its three axes are the reciprocals of the square roots of the principal moments of inertia (Figure 1, 2, 3 and 4): 1 1 2 2 2 2 2 2 2 2 2     I x  I y  I z  c z b y a x x x y y zz Figure 2. The inertia ellipsoid of the non-displaced moment of inertia tensor What the simulation offers to the researcher The simulation was developed in order to facilitate researchers and scientists while studying the mechanical properties of unmanned helicopter airframes and their input in autopilot algorithms. Further the simulation helps engineers in unmanned helicopter autopilot analysis and synthesis. The simulation helps the construction of the inertia ellipsoid of different rigid bodies and the observation of the principal moments of inertia. To make clear the effectiveness of the simulation while solving mechanics problems, several tasks are disclosed as examples. The solutions could be observed in 3D stereo mode. Each step of a solution has its