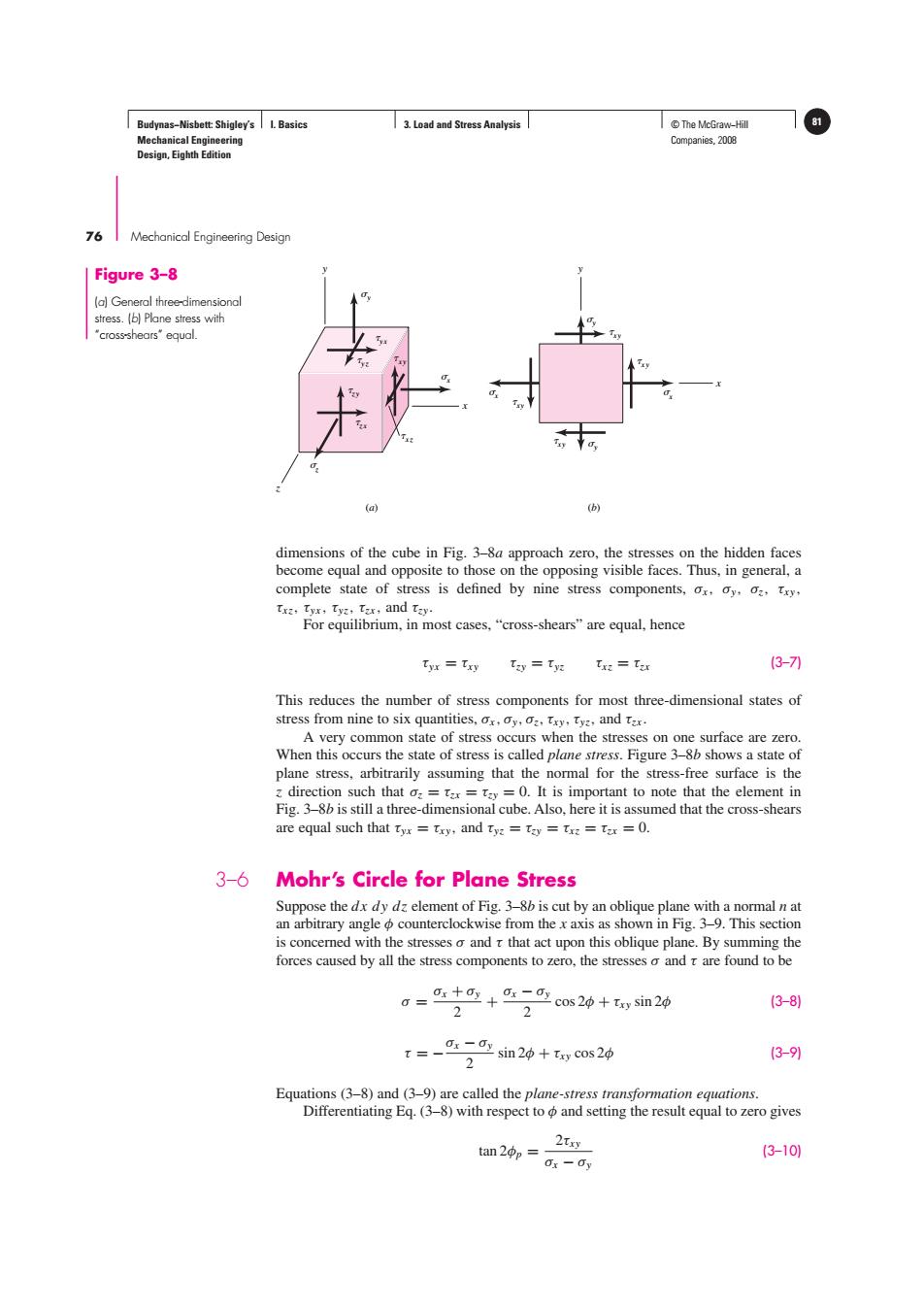
Budynas-Nisbett:Shigley's I.Basics 3.Load and Stress Analysis T©The McGraw-Hil 81 Mechanical Engineering Companies,2008 Design,Eighth Edition 76 Mechanical Engineering Desigr Figure 3-8 (a)General three-dimensional stress.(b)Plane stress with "cross-shears"equal. (a) (b) dimensions of the cube in Fig.3-8a approach zero,the stresses on the hidden faces become equal and opposite to those on the opposing visible faces.Thus,in general,a complete state of stress is defined by nine stress components,ox,oy.o.Txy. Txz,Tyx,Tyz,Tax,and Tay. For equilibrium,in most cases,"cross-shears"are equal,hence tyx Txy tiy=Tyz Txz Ta (3-7 This reduces the number of stress components for most three-dimensional states of stress from nine to six quantities,ox,oy.o.txy,ty,and ta. A very common state of stress occurs when the stresses on one surface are zero. When this occurs the state of stress is called plane stress.Figure 3-8b shows a state of plane stress,arbitrarily assuming that the normal for the stress-free surface is the z direction such that o=a=y=0.It is important to note that the element in Fig.3-8b is still a three-dimensional cube.Also,here it is assumed that the cross-shears are equal such that tyx =Txy,and tyz=tay =tx:=ta=0. 3-6 Mohr's Circle for Plane Stress Suppose the dx dy dz element of Fig.3-8b is cut by an oblique plane with a normal n at an arbitrary angle counterclockwise from the x axis as shown in Fig.3-9.This section is concerned with the stresses o and t that act upon this oblique plane.By summing the forces caused by all the stress components to zero,the stresses o and t are found to be a=sycos 20+txy sin 2 2 2 (3-8) t=xax sin20+tsy cos2 2 (3-91 Equations (3-8)and (3-9)are called the plane-stress transformation equations. Differentiating Eq.(3-8)with respect toand setting the result equal to zero gives tan2φp= 2Uxy (3-10) Ox-OyBudynas−Nisbett: Shigley’s Mechanical Engineering Design, Eighth Edition I. Basics 3. Load and Stress Analysis © The McGraw−Hill 81 Companies, 2008 76 Mechanical Engineering Design dimensions of the cube in Fig. 3–8a approach zero, the stresses on the hidden faces become equal and opposite to those on the opposing visible faces. Thus, in general, a complete state of stress is defined by nine stress components, σx , σy , σz, τxy , τxz, τyx , τyz, τzx , and τzy . For equilibrium, in most cases, “cross-shears” are equal, hence τyx = τxy τzy = τyz τxz = τzx (3–7) This reduces the number of stress components for most three-dimensional states of stress from nine to six quantities, σx , σy , σz, τxy , τyz, and τzx . A very common state of stress occurs when the stresses on one surface are zero. When this occurs the state of stress is called plane stress. Figure 3–8b shows a state of plane stress, arbitrarily assuming that the normal for the stress-free surface is the z direction such that σz = τzx = τzy = 0. It is important to note that the element in Fig. 3–8b is still a three-dimensional cube. Also, here it is assumed that the cross-shears are equal such that τyx = τxy , and τyz = τzy = τxz = τzx = 0. 3–6 Mohr’s Circle for Plane Stress Suppose the dx dy dz element of Fig. 3–8b is cut by an oblique plane with a normal n at an arbitrary angle φ counterclockwise from the x axis as shown in Fig. 3–9. This section is concerned with the stresses σ and τ that act upon this oblique plane. By summing the forces caused by all the stress components to zero, the stresses σ and τ are found to be σ = σx + σy 2 + σx − σy 2 cos 2φ + τxy sin 2φ (3–8) τ = −σx − σy 2 sin 2φ + τxy cos 2φ (3–9) Equations (3–8) and (3–9) are called the plane-stress transformation equations. Differentiating Eq. (3–8) with respect to φ and setting the result equal to zero gives tan 2φp = 2τxy σx − σy (3–10) y y x y yx xy xy xy x y xy xz x x y x y z x z yz zy z x (a) (b) Figure 3–8 (a) General three-dimensional stress. (b) Plane stress with “cross-shears” equal