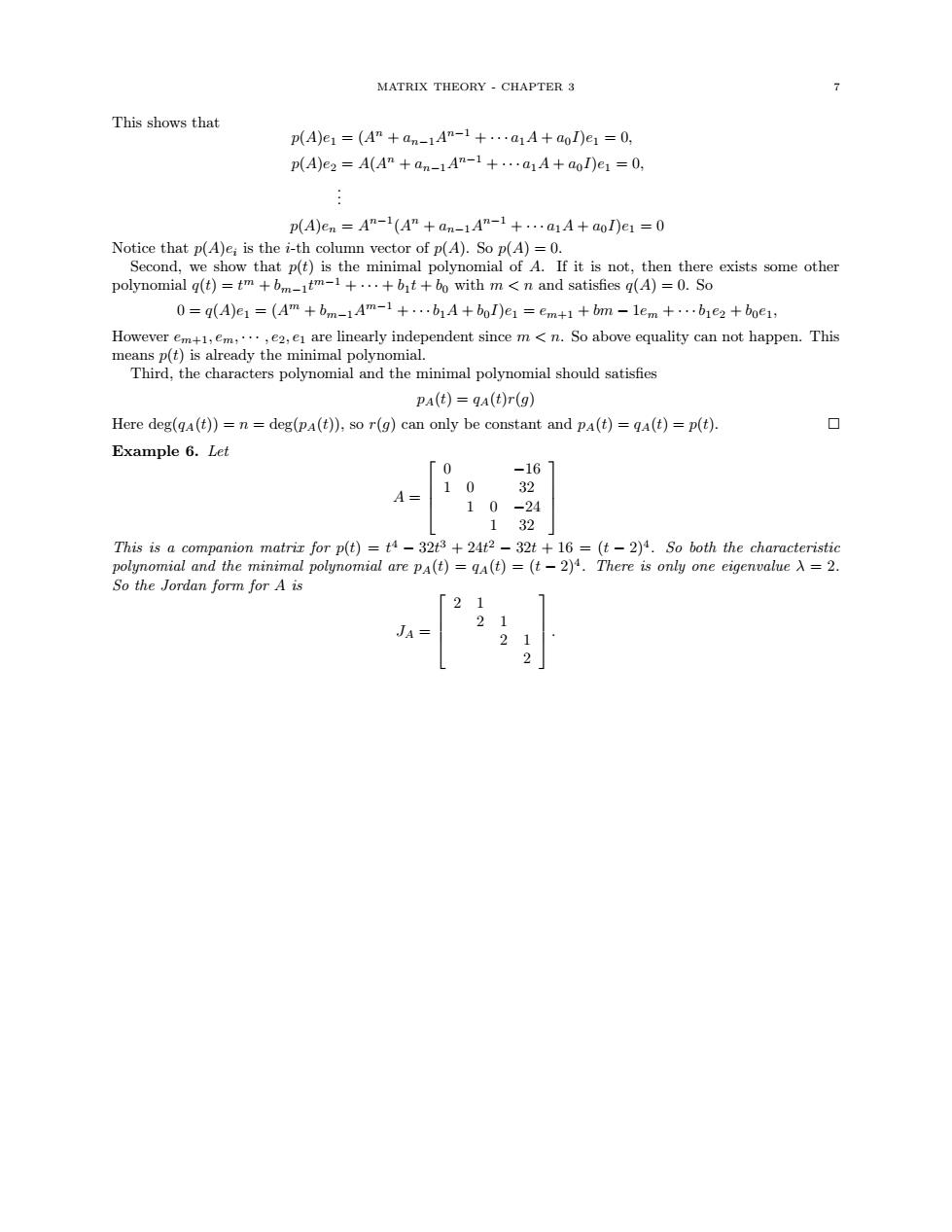
MATRIX THEORY CHAPTER 3 This shows that p(A)e1=(A"an-1Am-1+...aA+aol)e=0. p(A)e2 A(A"an-1A"-1+...aA+ao)e=0 p(A)e. -A-1(4+a- 4n-11 .…a1A+a0I)e1=0 Second,we show that p(t)is the minimal polynomial of A.itnot.then these exists some other 0=q(A)e1 =(Am+om-1Am-1+...A+bol)e1 em+i+om-lem+...oe2+boe1: However em+,em.,e2,e are linearly independent since m<n.So above equality can not happen.This PA(t)=gA(r(g) Here deg(A(t))=n=deg(pA()).sor(g)can only be constant and pA(t)=(t)-p(t). Example 6.Let 0 6 A= 10-24 132 This is a co anion matrir for p(t)=t-32t3+24t2-32t+16 =(t-2)4.So both the characteristic mAmn0=-m的mA= 2 21 21 2 MATRIX THEORY - CHAPTER 3 7 This shows that p(A)e1 = (A n + an−1A n−1 + · · · a1A + a0I)e1 = 0, p(A)e2 = A(A n + an−1A n−1 + · · · a1A + a0I)e1 = 0, . . . p(A)en = A n−1 (A n + an−1A n−1 + · · · a1A + a0I)e1 = 0 Notice that p(A)ei is the i-th column vector of p(A). So p(A) = 0. Second, we show that p(t) is the minimal polynomial of A. If it is not, then there exists some other polynomial q(t) = t m + bm−1t m−1 + · · · + b1t + b0 with m < n and satisfies q(A) = 0. So 0 = q(A)e1 = (A m + bm−1A m−1 + · · · b1A + b0I)e1 = em+1 + bm − 1em + · · · b1e2 + b0e1, However em+1, em, · · · , e2, e1 are linearly independent since m < n. So above equality can not happen. This means p(t) is already the minimal polynomial. Third, the characters polynomial and the minimal polynomial should satisfies pA(t) = qA(t)r(g) Here deg(qA(t)) = n = deg(pA(t)), so r(g) can only be constant and pA(t) = qA(t) = p(t). Example 6. Let A =     0 −16 1 0 32 1 0 −24 1 32     This is a companion matrix for p(t) = t 4 − 32t 3 + 24t 2 − 32t + 16 = (t − 2)4 . So both the characteristic polynomial and the minimal polynomial are pA(t) = qA(t) = (t − 2)4 . There is only one eigenvalue λ = 2. So the Jordan form for A is JA =     2 1 2 1 2 1 2     .